1.2 Inductive Reasoning

Goal
Use inductive reasoning
to make conjectures.
Key Words
• conjecture
• inductive reasoning
• counterexample
Student Help
STUDY TIP
Copy this table in your
notebook and complete
it. Do not write in your
textbook.
8 Chapter 1 Basics of Geometry
1.2 Inductive Reasoning
Scientists and mathematicians look for patterns and try to draw
conclusions from them. A conjecture is an unproven statement that
is based on a pattern or observation. Looking for patterns and making
conjectures is part of a process called inductive reasoning .
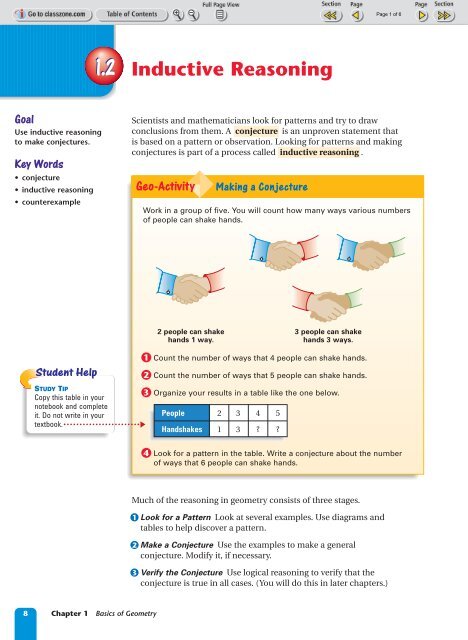
Geo-Activity
Making a Conjecture
Work in a group of five. You will count how many ways various numbers
of people can shake hands.
2 people can shake 3 people can shake
hands 1 way. hands 3 ways.
●1 Count the number of ways that 4 people can shake hands.
●2 Count the number of ways that 5 people can shake hands.
●3 Organize your results in a table like the one below.
People 2 3 4 5
Handshakes 1 3 ? ?
●4 Look for a pattern in the table. Write a conjecture about the number
of ways that 6 people can shake hands.
Much of the reasoning in geometry consists of three stages.
●1 Look for a Pattern Look at several examples. Use diagrams and
tables to help discover a pattern.
●2 Make a Conjecture Use the examples to make a general
conjecture. Modify it, if necessary.
●3 Verify the Conjecture Use logical reasoning to verify that the
conjecture is true in all cases. (You will do this in later chapters.)

EXAMPLE 1 Make a Conjecture
Science Complete the conjecture.
SCIENTISTS use inductive
reasoning as part of the
scientific method. They
make observations, look for
patterns, and develop
conjectures (hypotheses) that
can be tested.
Student Help
READING TIP
Recall that the symbols
and p are two ways
of expressing
multiplication.
Conjecture: The sum of any two odd numbers is __?__.
Solution
Begin by writing several examples.
1 1 2 5 1 6 3 7 10
3 13 16 21 9 30 101 235 336
Each sum is even. You can make the following conjecture.
ANSWER The sum of any two odd numbers is even.
EXAMPLE 2 Make a Conjecture
Complete the conjecture.
Conjecture: The sum of the first n odd positive integers is __?__.
Solution
List some examples and look for a pattern.
1 1 3 1 3 5 1 3 5 7
1 1 2
4 2 2
9 3 2
ANSWER The sum of the first n odd positive integers is n 2 .
Make a Conjecture
Complete the conjecture based on the pattern in the examples.
1. Conjecture: The product of any two odd numbers is __?__.
EXAMPLES
1 1 1 3 5 15 3 11 33
7 9 63 11 11 121 1 15 15
16 4 2
2. Conjecture: The product of the numbers (n 1) and (n 1) is __?__.
EXAMPLES
1 p 3 2 2 1 3 p 5 4 2 1 5 p 7 6 2 1
7 p 9 8 2 1 9 p 11 10 2 1 11 p 13 12 2 1
1.2 Inductive Reasoning 9

IStudent Help
I CLASSZONE.COM
MORE EXAMPLES
More examples at
classzone.com
10 Chapter 1 Basics of Geometry
Counterexamples Just because something is true for several
examples does not prove that it is true in general. To prove a
conjecture is true, you need to prove it is true in all cases.
A conjecture is considered false if it is not always true. To prove a
conjecture is false, you need to find only one counterexample.
A counterexample is an example that shows a conjecture is false.
EXAMPLE 3 Find a Counterexample
Show the conjecture is false by finding a counterexample.
Conjecture: The sum of two numbers is always greater than the larger
of the two numbers.
Solution
Here is a counterexample. Let the two numbers be 0 and 3.
The sum is 0 3 3, but 3 is not greater than 3.
ANSWER The conjecture is false.
EXAMPLE 4 Find a Counterexample
Show the conjecture is false by finding a counterexample.
Conjecture: All shapes with four sides the same length are squares.
Solution
Here are some counterexamples.
These shapes have four sides the same length, but they are
not squares.
ANSWER The conjecture is false.
Find a Counterexample
Show the conjecture is false by finding a counterexample.
3. If the product of two numbers is even, the numbers must be even.
4. If a shape has two sides the same length, it must be a rectangle.

1.2
Exercises
Guided Practice
Vocabulary Check
Practice and Applications
Extra Practice
See p. 675.
Skill Check
Homework Help
Example 1: Exs. 8−16
Example 2: Exs. 8−16
Example 3: Exs. 17–19
Example 4: Exs. 17–19
1. Explain what a conjecture is.
2. How can you prove that a conjecture is false?
Complete the conjecture with odd or even.
3. Conjecture: The difference of any two odd numbers is __?__.
4. Conjecture: The sum of an odd number and an even number
is __?__.
Show the conjecture is false by finding a counterexample.
5. Any number divisible by 2 is divisible by 4.
6. The difference of two numbers is less than the greater number.
7. A circle can always be drawn around a four-sided shape so that it
touches all four corners of the shape.
8. Rectangular Numbers The dot patterns form rectangles with a
length that is one more than the width. Draw the next two figures
to find the next two “rectangular” numbers.
2
9. Triangular Numbers The dot patterns form triangles. Draw the
next two figures to find the next two “triangular” numbers.
1
6
3
Technology Use a calculator to explore the pattern. Write a
conjecture based on what you observe.
12
10. 101 25 __?__ 11. 11 11 __?__ 12. 3 4 __?__
101 34 __?__ 111 111 __?__ 33 34 __?__
101 49 __?__ 1111 1111 __?__ 333 334 __?__
6
20
10
1.2 Inductive Reasoning 11

sun
Earth’s
orbit
IStudent Help
I CLASSZONE.COM
HOMEWORK HELP
Extra help with problem
solving in Exs. 13–14 is
at classzone.com
Science
Earth
moon
moon’s
orbit
Not drawn to scale
FULL MOONS happen when
Earth is between the moon
and the sun.
Application Links
CLASSZONE.COM
12 Chapter 1 Basics of Geometry
Making Conjectures Complete the conjecture based on the pattern
you observe.
13. Conjecture: The product of an odd number and an even number
is __?__.
3 p 6 18 22 p 13 286 43 p 102 4386
5 p 12 60 5 p 2 10 254 p 63 16,002
14 p 9 126 11 p (4) 44
14. Conjecture: The sum of three consecutive integers is always three
times __?__.
3 4 5 3 p 4 6 7 8 3 p 7 9 10 11 3 p 10
4 5 6 3 p 5 7 8 9 3 p 8 10 11 12 3 p 11
5 6 7 3 p 6 8 9 10 3 p 9 11 12 13 3 p 12
15. Counting Diagonals In the shapes below, the diagonals are
shown in blue. Write a conjecture about the number of diagonals
of the next two shapes.
16. Moon Phases A full moon occurs when the moon is on the
opposite side of Earth from the sun. During a full moon, the
moon appears as a complete circle. Suppose that one year, full
moons occur on these dates:
July
21
Thursday
S M T W T F S
1 2
3 4 5 6 7 8 9
10 11 12 13 14 15 16
17 18 19 20 21 22 23
24 25 26 27 28 29 30
31
0
August
19
Friday
S M T W T F S
1 2 3 4 5 6
7 8 9 10 11 12 13
14 15 16 17 18 19 20
21 22 23 24 25 26 27
28 29 30 31
2
September
18
Sunday S M T W T F S1
S M T W T F S
1 2 3
4 5 6 7 8 9 10
11 12 13 14 15 16 17
18 19 20 21 22 23 24
25 26 27 28 29 30
October
17
Monday
2 3 4 5 6 7 8
9 1011 12 13 14 15
16 17 18 19 20 21 22
23 24 25 26 27 28 29
30 31
November
16
Wednesday
S M T W T F S
1 2 3 4 5
6 7 8 9 10 11 12
13 14 15 16 17 18 19
20 21 22 23 24 25 26
27 28 29 30
Determine how many days are between these full moons and
predict when the next full moon occurs.
Error Analysis Show the conjecture is false by finding a
counterexample.
December
15
Thursday
S M T W T F S
1 2 3
4 5 6 7 8 9 10
11 12 13 14 15 16 17
18 19 20 21 22 23 24
25 26 27 28 29 30 31
17. Conjecture: If the product of two numbers is positive, then the
two numbers must both be positive.
18. Conjecture: All rectangles with a perimeter of 20 feet have the
same area. Note: Perimeter 2(length width).
19. Conjecture: If two sides of a triangle are the same length, then the
third side must be shorter than either of those sides.
5
9

Standardized Test
Practice
Mixed Review
Algebra Skills
20. Telephone Keypad Write a conjecture about the letters you
expect on the next telephone key. Look at a telephone to see
whether your conjecture is correct.
21. Challenge Prove the conjecture below by writing a variable
statement and using algebra.
Conjecture: The sum of five consecutive integers is always
divisible by five.
x (x 1) (x 2) (x 3) (x 4) __?__
22. Multiple Choice Which of the following is a counterexample of
the conjecture below?
Conjecture: The product of two positive numbers is always
greater than either number.
A 2, 2 B 1
2 , 2 C 3, 10 D 2, 1
23. Multiple Choice You fold a large piece of paper in half four times,
then unfold it. If you cut along the fold lines, how many identical
rectangles will you make?
F 4 G 8 H 16 J 32
Describing Patterns Sketch the next figure you expect in the
pattern. (Lesson 1.1)
24. 25.
Using Integers Evaluate. (Skills Review, p. 663)
26. 8 (3) 27. 2 9 28. 9 (1) 29. 7 3
30. 3(5) 31. (2)(7) 32. 20 (5) 33. (8) (2)
Evaluating Expressions Evaluate the expression when x 3.
(Skills Review, p. 670)
34. x 7 35. 5 x 36. x 9 37. 2x 5
38. x 2 6 39. x 2 4x 40. 3x 2
1
Z
2
Y
X
41. 2x 3
1.2 Inductive Reasoning 13
3