Exercise 1

Dynamics 2
Lecturer: Prof. Dr. G. Lohmann
Due date: 22.04.2011
Exercise 1
Summer semester 2013
Exercise 1
15.04.2011
April 16, 2013
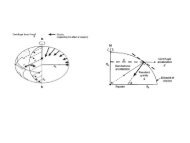
1. Ekman spiral
Show the Ekman equation and solution which was shown in the lecture. Draw a
figure.
4 points
2. Wind-driven ocean circulation
When the windstress is only zonal, the Sverdrup transport is
ρ 0 βV = curl τ = − ∂ ∂y τ x (1)
and Ekman transports and Ekman pumping velocity of the surface layer are
Assume furthermore
ρ 0 fV E = −τ x (2)
ρ 0 w E = − ∂ ∂y τ x . (3)
τ x = −τ ∗ cos(πy/B) (4)
for an ocean basin 0 < x < L, 0 < y < B. Westerlies are in the northern part and
easterlies are in the equatorward part.
Tasks:
a) Calculate V, V E , w E .
a) At which latitudes are |V | and |V E | at their maximum? Calculate their magnitudes.
Take constant f = 10 −4 s −1 and β = 1.8 · 10 −11 m −1 s −1 and B =
5000 km, τ ∗ /ρ 0 = 10 −4 m 2 s −2 .
b) calculate the maximum of w E for constant f (value see above).
4 points
1

Dynamics 2
Lecturer: Prof. Dr. G. Lohmann
Due date: 22.04.2011
Summer semester 2013
Exercise 1
15.04.2011
3. The Stommel model: of the wind-driven circulation in a homogeneous ocean of
constant depth h is described by
R∇ 2 ψ + β∂ x ψ =
1
ρ 0
(∂ x τ y 0 − ∂ y τ x 0 ) (5)
where R is a coefficient of bottom friction, β the derivative of the Coriolis frequency
at a central latitude, and the 2-dimensional τ 0 the windstress vector. The depth
integrated velocity is
(U, V ) =
Finally, ψ is the related streamfunction
∫ 0
−h
(u, v)dz .
U = −∂ y ψ, V = ∂ x ψ .
a) Derive this equation from the conservation of momentum (linearized) and mass
(volume!) assuming w = 0 at the mean surface z = 0 and at the bottom z = −h.
For simplicity take Cartesian coordinates for the horizontal, β = df/dy. Boundary
condition for the flux of momentum are τ(z = 0) = τ 0 and τ(z = −h) = R(−V, U).
b) in the boundary layer the terms on the left hand side of (5) get large. Show by
scaling that the width of the layer is W = R/β.
c) how large must R be to get a width W = 100 km? (β = 2 × 10 −11 m −1 s −1 ).
6 points
Notes on submission form of the exercises: Working in study groups is encouraged,
but each student is responsible for his/her own solution. The answers to the questions
shall be provided at the tutorium at the due date.
2