Explicit inverses of some tridiagonal matrices - Estudo Geral ...

More documents

Recommendations

Info

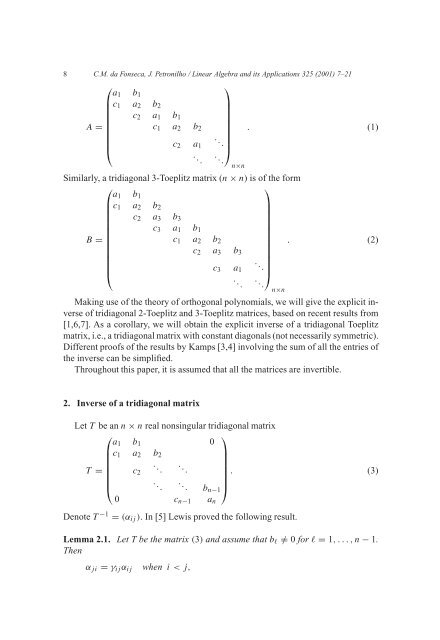
8 C.M. da Fonseca, J. Petronilho / Linear Algebra and its Applications 325 (2001) 7–21 ⎛ ⎞ a 1 b 1 c 1 a 2 b 2 c 2 a 1 b 1 A = c 1 a 2 b 2 . (1) ⎜ . ⎝ c 2 a .. 1 ⎟ ⎠ . .. . .. Similarly, a tridiagonal 3-Toeplitz matrix (n × n) is of the form ⎛ ⎞ a 1 b 1 c 1 a 2 b 2 c 2 a 3 b 3 c 3 a 1 b 1 B = c 1 a 2 b 2 . (2) c 2 a 3 b 3 ⎜ . ⎝ c 3 a .. 1 ⎟ ⎠ . .. . .. Making use of the theory of orthogonal polynomials, we will give the explicit inverse of tridiagonal 2-Toeplitz and 3-Toeplitz matrices, based on recent results from [1,6,7]. As a corollary, we will obtain the explicit inverse of a tridiagonal Toeplitz matrix, i.e., a tridiagonal matrix with constant diagonals (not necessarily symmetric). Different proofs of the results by Kamps [3,4] involving the sum of all the entries of the inverse can be simplified. Throughout this paper, it is assumed that all the matrices are invertible. n×n n×n 2. Inverse of a tridiagonal matrix Let T be an n × n real nonsingular tridiagonal matrix ⎛ ⎞ a 1 b 1 0 c 1 a 2 b 2 T = . c .. . .. 2 . (3) ⎜ ⎝ . .. . ⎟ .. bn−1 ⎠ 0 c n−1 a n Denote T −1 = (α ij ). In [5] Lewis proved the following result. Lemma 2.1. Let T be the matrix (3) and assume that b l /= 0 for l = 1,...,n− 1. Then α ji = γ ij α ij when i
where C.M. da Fonseca, J. Petronilho / Linear Algebra and its Applications 325 (2001) 7–21 9 γ ij = j−1 ∏ l=i c l b l . Of course this reduces to α ij = α ji , when T is symmetric. Using this lemma, one can prove that there exist two finite sequences {u i } and {v i } (i = 1,...,n− 1) such that ⎛ ⎞ u 1 v 1 u 1 v 2 u 1 v 3 ··· u 1 v n γ 12 u 1 v 2 u 2 v 2 u 2 v 3 ··· u 2 v n T −1 = γ 13 u 1 v 3 γ 23 u 2 v 3 u 3 v 3 ··· u 3 v n . (4) ⎜ . . . ⎝ . . . . .. . ⎟ . ⎠ γ 1,n u 1 v n γ 2,n u 2 v n γ 3,n u 3 v n ··· u n v n Since {u i } and {v i } are only defined up to a multiplicative constant, we make u 1 = 1. The following result shows how to compute {u i } and {v i }. The determination of these sequences is very similar to the particular case studied in [8], where the reader may check for details. Lemma 2.2. (i) The {v i } (i = 1,...,n− 1) can be computed from where v 1 = 1 d 1 , v k =− b k−1 d k v k−1 , k = 2,...,n, d n = a n , d i = a i − b ic i , i = n − 1,...,1. d i+1 (ii) The {u i } (i = 1,...,n− 1) can be computed from u n = 1 , δ n v n where δ 1 = a 1 , u k =− b k δ k u k+1 , k = n − 1,...,1, δ i = a i − b i−1c i−1 δ i−1 , i = 2,...,n− 1. Notice that for centro-symmetric matrices, i.e., a ij = a n+1−i,n+1−j , we have v i = u n+1−i . The following proposition is known in the literature. We give a proof based on the lecture of [8]. Theorem 2.1. Let T be the n × n tridiagonal matrix defined in (3) and assume that b l /= 0 for l = 1,...,n− 1.Then
Page 1: Linear Algebra and its Applications
Page 5 and 6: C.M. da Fonseca, J. Petronilho / Li
Page 11 and 12: where C.M. da Fonseca, J. Petronilh
Page 15: References C.M. da Fonseca, J. Petr

Explicit inverses of some tridiagonal matrices - Estudo Geral ...

Create successful ePaper yourself

Delete template?

Save as template?