Smart Materials Solve Contradictions - Systematic Innovation

More documents

Recommendations

Info

able to replace. Of the 30,000 or so wrist replacement operations carried out in the UK every year, almost half are replacementreplacements, where a surgeon is replacing a previous replacement that has somehow failed. big opportunity, then we might start making some innovation progress. Figure 2 illustrates what the contradiction-aware designer might see when looking at this wrist replacement problem: BECAUSE durable replacement joint compressive load spreading AND AND taper tensile loading no taper REQUIRES Figure 2: Wrist Replacement Joint Contradiction Figure 1: X-Ray Of Typical Wrist Replacement One of the main problems, as may be seen from the figure is that a lot of metal is required and there isn’t a lot of bone around to hold it. The problem is further complicated because, unlike, for example, hip replacements, wrists have to cope with a much wider range of loads. A hip joint spends nearly all of its time in compression, and when it is in tension, the load is limited to the weight of the leg. A wrist joint, on the other hand, experiences both tension and compression loads on a regular basis. In both cases, these loads can exceed the weight of a person’s whole body. Although wrist replacement joint engineers might not explicitly recognise it, they are caught in the middle of a challenging contradiction. The main problem area is the tapered pin inserted into the prepared end of the radius. When the joint is in compression (such as, say, when the patient is doing a push-up exercise), it is desirable to distribute the loads over as much of the taper as possible in order to minimise the likelihood of splintering the radius. Conversely when the joint is in tension (such as when the patient is doing a pull-up exercise), the taper is a bad thing, because it becomes very easy to dis-lodge the pin. One possible remedy to this problem, of course, is to tell the patient they shouldn’t do pullups or lift heavy objects anymore. Well, if that sounds like a pretty big compromise to expect the patient to make, it is ultimately not too far different from the compromise that the joint designer is also making. By recognising that a taper is a good idea for the compression case, an optimization-focused design strategy now sets about ‘optimizing’ the taper angle. If the designer was made aware that every time he or she started conducting sophisticated mathematical design calculations to find the optimum anything, they were missing a It is difficult for anyone – TRIZ-aware or otherwise – to contemplate a problem statement that says we want a design solution that has a taper and doesn’t have a taper. But, of course, that is exactly what we need to be thinking about if we are to create some kind of a breakthrough solution to the problem. One of the reasons the Figure 2 template (Reference 2) is useful, is that it offers the problem solver several contradiction resolution opportunities. Essentially each of the six lines in the picture represents a conflict or contradiction. Fail to solve one of them, and we still have five other possibilities. Were the designer to start with the ‘taper and no taper’ physical contradiction, they would immediately be presented with the usual separate-in-space, separate-in-time, etc strategies. Recently, these strategies have been complemented with a model that allows a problem solver to map the eligible separation strategies to the Inventive Principles that are most likely to help resolve the contradiction (Reference 3). The eventual model is illustrated in Figure 3: 6, 8, 12, 33 38, 39 1, 28, 30 31, 40 CONDITION SPACE 14, 17, 26, 29 2, 3, 4 5, 13, 22 24, 25, 35 11, 16,19 20, 23 7, 15, 27 34, 37 TIME 2, 3, 4 5, 13, 22 24, 25, 35 32, 36 9, 10, 18, 21 Figure 3: Mapping Physical Contradiction Resolution Strategies To The 40 Inventive Principles For this particular wrist replacement ‘taper-andno-taper’ problem, all three of the time, space
and condition resolution strategies appear to be possible, and so the place to start looking for the most relevant Inventive Principles is at the region at the centre of the figure where all three regions intersect. What this means is that the most likely Principles to solve the wrist replacement problem – based on the thousands of problem solvers that have travelled a similar road before us – are 2, 3, 4, 5, 13, 22, 25 and 35. Although it might not look or feel like it, Principle 35 is the one that is trying to point problem solvers in the direction of smart material solutions. As discussed in Reference 4, Principle 35 is simultaneously the most frequently recommended Inventive Principle and the one that is the least well applied by problem solvers. When applied to the ‘taper and no taper’ problem, it is easy to see why – simply telling a designer to ‘change one of the design parameters’ is only slightly better than useless in the vast majority of cases. 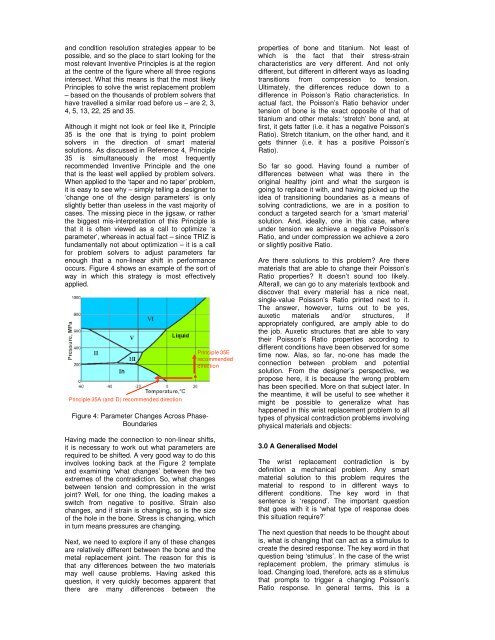The missing piece in the jigsaw, or rather the biggest mis-interpretation of this Principle is that it is often viewed as a call to optimize ‘a parameter’, whereas in actual fact – since TRIZ is fundamentally not about optimization – it is a call for problem solvers to adjust parameters far enough that a non-linear shift in performance occurs. Figure 4 shows an example of the sort of way in which this strategy is most effectively applied. Principle 35A (and D) recommended direction Figure 4: Parameter Changes Across Phase- Boundaries Principle 35E recommended direction Having made the connection to non-linear shifts, it is necessary to work out what parameters are required to be shifted. A very good way to do this involves looking back at the Figure 2 template and examining ‘what changes’ between the two extremes of the contradiction. So, what changes between tension and compression in the wrist joint? Well, for one thing, the loading makes a switch from negative to positive. Strain also changes, and if strain is changing, so is the size of the hole in the bone. Stress is changing, which in turn means pressures are changing. Next, we need to explore if any of these changes are relatively different between the bone and the metal replacement joint. The reason for this is that any differences between the two materials may well cause problems. Having asked this question, it very quickly becomes apparent that there are many differences between the properties of bone and titanium. Not least of which is the fact that their stress-strain characteristics are very different. And not only different, but different in different ways as loading transitions from compression to tension. Ultimately, the differences reduce down to a difference in Poisson’s Ratio characteristics. In actual fact, the Poisson’s Ratio behavior under tension of bone is the exact opposite of that of titanium and other metals: ‘stretch’ bone and, at first, it gets fatter (i.e. it has a negative Poisson’s Ratio). Stretch titanium, on the other hand, and it gets thinner (i.e. it has a positive Poisson’s Ratio). So far so good. Having found a number of differences between what was there in the original healthy joint and what the surgeon is going to replace it with, and having picked up the idea of transitioning boundaries as a means of solving contradictions, we are in a position to conduct a targeted search for a ‘smart material’ solution. And, ideally, one in this case, where under tension we achieve a negative Poisson’s Ratio, and under compression we achieve a zero or slightly positive Ratio. Are there solutions to this problem? Are there materials that are able to change their Poisson’s Ratio properties? It doesn’t sound too likely. Afterall, we can go to any materials textbook and discover that every material has a nice neat, single-value Poisson’s Ratio printed next to it. The answer, however, turns out to be yes, auxetic materials and/or structures, if appropriately configured, are amply able to do the job. Auxetic structures that are able to vary their Poisson’s Ratio properties according to different conditions have been observed for some time now. Alas, so far, no-one has made the connection between problem and potential solution. From the designer’s perspective, we propose here, it is because the wrong problem has been specified. More on that subject later. In the meantime, it will be useful to see whether it might be possible to generalize what has happened in this wrist replacement problem to all types of physical contradiction problems involving physical materials and objects: 3.0 A Generalised Model The wrist replacement contradiction is by definition a mechanical problem. Any smart material solution to this problem requires the material to respond to in different ways to different conditions. The key word in that sentence is ‘respond’. The important question that goes with it is ‘what type of response does this situation require?’ The next question that needs to be thought about is, what is changing that can act as a stimulus to create the desired response. The key word in that question being ‘stimulus’. In the case of the wrist replacement problem, the primary stimulus is load. Changing load, therefore, acts as a stimulus that prompts to trigger a changing Poisson’s Ratio response. In general terms, this is a
Page 1: Smart Materials Solve Contradiction
Page 5 and 6: Applying a bit of thermochromic ink

Smart Materials Solve Contradictions - Systematic Innovation

Create successful ePaper yourself

Delete template?

Save as template?