Chapter 3 Shearing Force and Bending Moment Diagram - FET

Department of Chemical Engineering
Strength of Materials for Chemical Engineers (0935381)
Chapter 3
Shearing Force and Bending Moment Diagram
Beam: is a structural member subjected to a system of external forces at right angles to
axis.
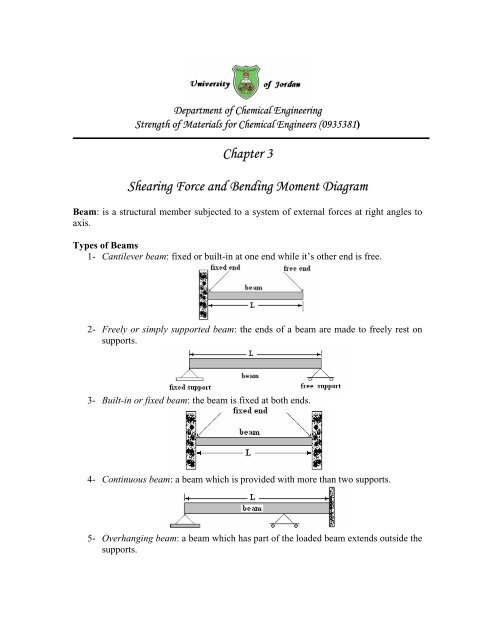
Types of Beams
1- Cantilever beam: fixed or built-in at one end while it’s other end is free.
2- Freely or simply supported beam: the ends of a beam are made to freely rest on
supports.
3- Built-in or fixed beam: the beam is fixed at both ends.
4- Continuous beam: a beam which is provided with more than two supports.
5- Overhanging beam: a beam which has part of the loaded beam extends outside the
supports.

Statically Determinate Beams
Cantilever, simply supported, overhanging beams are statically determinate beams as the
reactions of these beams at their supports can be determined by the use of equations of
static equilibrium and the reactions are independent of the deformation of the beam.
There are two unknowns only.
Statically Indeterminate Beams
Fixed and continuous beams are statically indeterminate beams as the reactions at
supports cannot be determined by the use of equations of static equilibrium. There are
more than two unknown.
Types of Loads:
1- Concentrated load assumed to act at a point and immediately introduce an
oversimplification since all practical loading system must be applied over a finite
area.
2- Distributed load are assumed to act over part, or all, of the beam and in most cases
are assumed to be equally or uniformly distributed.
a- Uniformly distributed.
b- Uniformly varying load.

Shearing Force (S.F.)
Shearing force at the section is defined as the algebraic sum of the forces taken on one
side of the section.
Bending Moment (B.M)
Bending moment is defined as the algebraic sum of the moments of the forces about the
section, taken on either sides of the section.
1. S.F.and B.M. Diagrams for Beams Carrying Concentrated Loads Only:

• If the S.F. is zero the bending moment will remain constant.
• If the S.F. is positive the slope of the B.M. curve is positive.
• If the S.F. is negative the slope of the B.M. curve is negative.
• The difference in B.M. between any two points equals the area under the S.F.
curve for the same points.
• Between concentrated loads, there is no change in shear and the shear force curve
plots as a straight horizontal line.
• At each concentrated load or reaction, the value of the shear force changes
abruptly by an amount equal to the load or reaction force.
• The maximum bending moment occurs at a point where the shear curve crosses
its zero axis.
2. S.F.and B.M. Diagrams for Beams Carrying Distributed Loads Only:

3. S.F. and B.M. Diagrams for Beams Carrying Combined Concentrated and
Uniformly Distributed Loads:

Points of Contraflexure
It is a point where the curvature of the beam changes sign and occurs at a point where the
B.M. is zero (other than the ends).
In order to find the exact location of the contraflexure point you have to solve and find
the zeros of the bending moment equation applied in the interval where the curve crosses
the zero line.
For the above example find the zeros of the second order bending moment equation in the
third interval.
4. S.F. and B.M. Diagrams for Beams Carrying Couple or Moment:

At each couple or moment, the value of the bending moment changes abruptly by an
amount equal to the couple or moment.
Relationship between Shear Force and Bending Moment
dM
• The maximum or minimum B.M. occurs where = S. F.
= 0
dx
• Thus where S.F. is zero and crosses the zero axis B.M. is maximum or minimum.
dM
• If S.F. is zero then = 0 ⇒ ∫ dM = ∫ 0dx
⇒ M = constant
dx
dM
• Since = S.F.
then where the S.F. is positive the slope of the B.M. diagram is
dx
positive, and where the S.F. is negative the slope of the B.M. diagram is also
negative.
• The area of the S.F. diagram between any two points, from basic calculus
dM
= S.
F.
⇒ ∫ dM = ∫ S.
F.
dx ⇒ M = ∫ S.
F.
dx
dx