Mass-Radius Relation of Degenerate Configurations

1Compact Stars – White Dwarfs, Planets, Neutron StarsWhite dwarf structure dominated by P (ρ) for degenerateelectron gas.[ ] (n + 1) K 1/2R =ρ c (1−n)/(2n) ξ4πG1[ ]M = 4πR (3−n)/(1−n) (n + 1) K n/(n−1)ξ (3−n)/(n−1)[ (n + 1) K= 4π4πG4πG] 3/2ρ c(3−n)/(2n)1( )−ξ1 2 θ′ 1 .( )−ξ1 2 θ′ 1Non-relativistic γ = 5/3:K =(3π2 ) 2/35¯h 2(N o Y e ) 5/3 = 10 13 Ye5/3m eR = 1.121 × 10 4 ρ −1/6c,6(2Y e ) 5/6 kmM = 0.496ρ 1/2c,6 (2Y e) 5/2 M ⊙ = 0.701Relativistic γ = 4/3:cgs( ) R −310 4 (2Y e ) 5 M ⊙ .km(1)(3π2 ) 1/3K = ¯hc (N o Y e ) 4/3 = 1.24 × 10 15 Ye4/34R = 3.35 × 10 4 ρ −1/3c,6(2Y e ) 2/3 kmM = 1.457 (2Y e ) 2 M ⊙ .cgs(2)

2Very low density (Thomas-Fermi regime γ = 10/3):K = 31/3 π 3 e 210( ) 10/3 ( 4πNo 3¯hcAm e c 2 ¯hce 2 ) 6= 1.05 × 10 13 ( 12A) 10/3cgsR = 1.18 × 10 5 ( 12A) 5/3ρ 2/3ckm( ) 12 5M = 0.001915 ρ 3 cAM ⊙= 2.88 × 10 −8 ( A12) 5/2 ( ) R 9/210 4 M ⊙ .kmPhysical reasoning behind the Chandrasekhar mass:(3)Consider N degenerate fermions in a star of radius R, sothat number density n ∝ NR −3 . Momentum of a fermion is∼ ¯hn 1/3 and Fermi energy is E F ∼ ¯hcN 1/3 R −1 . The gravitationalenergy per fermion is ∼ −GMm B R −1 if M = Nm B .The total energy isE = E F + E G = ¯hcN 1/3 /R − GNm 2 b /R.Equilibrium is reached when this is minimized. Both termsscale as 1/R.When E is positive, E can be decreased by increasing R.This decreases E F so that eventually the fermions become nonrelativistic:then E F ∼ p 2 F ∼ R−2 . This then decreases fasterthan E G , so E becomes negative. However, as R → ∞, E → 0.This implies there is a minimum of E at a finite value of R.When E is negative, E can be decreased without bound bydecreasing R so that no equilibrium state is possible and ablack hole forms.

3The maximum baryon number for equilibrium is determinedby setting E = 0:N max ∼(¯hcGm 2 b) 3/2∼ 2 × 10 57M max ∼ N max m B ∼ 1.5 M ⊙ .Note that the mass is independent of the fermion’s mass.The radius at equilibrium is set by the condition E F ≥ mc 2 ,R ≤ ¯hcmc 2 (¯hcGm 2 B) 1/2∼{5 × 103 km, m = me3 km, m = m n .At sufficiently high density, neutronization and pyconuclearreactions can occur. Thus, both A and N −Z will increase withdensity. The neutronization threshold for 56 Fe is about 10 9 gcm −3 . At this density, the Fermi energy of an electron is aboutm c c 2 +3.695 MeV, the threshold for the inverse beta-decay 56 Fe+ e − → 56 Mn + ν e . The Mn immediately electron captures:56 Mn + e − → 56 Cr + ν e . The Cr is stable until densities above10 10 g cm −3 are reached.Lighter nuclei have other thresholds: 4 He is at 20.6 MeV,12 C is at 13.4 MeV, 16 O is at 10.4 MeV and 20 Ne is at 7.0MeV. The loss of electrons softens the EOS: the Chandrasekharmass decreases. A white dwarf at these densities will begin togravitationally collapse. Thus the maximum density < ∼ 10 10 gcm −3 , with a minimum radius > ∼ 1500 km.

4Electrostatic correctionsIn a degenerate system in which the nuclei are ordered ineither a solid or liquid, there is Coulomb energy associated withthe ordering. If the nuclei are equally spaced and surroundedby a uniform density electron gas, the interaction energy perelectron isE c /Z = − 9 Ze 2= − 9 ( 4π10 R c 10 3) 1/3Z 2/3 e 2 n 1/3ewhere n e = 3Z/(4πRc). 3 The corresponding pressure isP c = n 2 ∂E c /Ze = − 3 ( ) 4π 1/3Z 2/3 e 2 n 4/3e .∂n e 10 3In the extreme relativistic limit this is just a constant fraction(a few percent) of the degeneracy pressure:(P c= − 25/3 3 1/3 e2P d 5 π)¯hc Z2/3 .In the non-relativistic limit, P c becomes more important atlower and lower densities:P c= − m ee 2 Z 2/3P d (2n e ) 1/3 π¯h 2.At low enough density, P c = −P d :n e,c = Z2 ( m e e 2) 32π 3¯h 6 (ρ c ≃ 0.4Z 2 g cm −3) ,and the total pressure vanishes. For iron this is about 250 gcm −3 , which is not the laboratory value of 7.86 g cm −3 becauseit is incorrect to treat the e − gas as uniform.

5The electron Fermi energy, modified by Coulomb potentialE F = −eV (r) + p2 F2m e.is constant in space, otherwise electrons would move to a lowerE F . The electron density isn e = 8π3h 3p3 F = 8π3h 3 [2m e (E F + eV (r))] 3/2 .The potential is determined by Poisson’s equation∇ 2 V = 4πen e + nuclear contributionwhere the last term is effectively a delta function at the origin.Omitting it for r > 0, we have the boundary condition:rV (r) → Ze as r → 0. The electric field should vanish atthe outer boundary, since this volume must be overall neutral:dV/dr| Rc= 0. Poisson’s equation in spherical geometry isd 2 φdx 2 = φ3/2x( )E F + eV (r) = Ze2 φ (r) 128Z 1/3 me e 2, x = rr9π 2 ¯h 2 .Eq. (4) has boundary conditionsφ (0) = 1,1/2,(4)φ (x o ) ′ ≡ dφ∣dx xo= φ (x o),x owhere x o corresponds to r = R c . The latter condition canbe seen by evaluation of Z = 4π ∫ n e r 2 dr over the entire volume.The equation Eq. (4) has a unique solution, when φ ′ (0) =−1.588071, in that as x o → ∞, φ(x o ) → 144x −3 o → 0. Otherwise,for larger values of φ ′ (x o ), φ doesn’t vanish anywhere

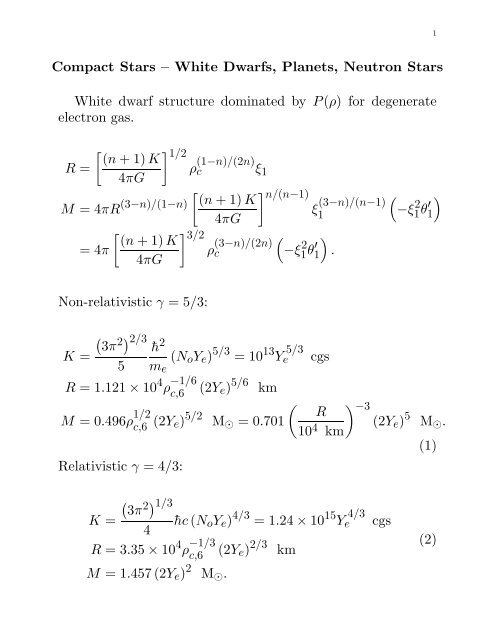
6and diverges as x → ∞. At some point, the second boundarycondition will be satisfied.The pressure at the outer boundary is that of free particlesP =8π15h 3 m ep 5 F (R c) = 110π Z2 e 2 ( 128Z9π 2 ) 4/3 (me e 2¯h 2 ) 4 [ φ (xo )x o] 5/2.The density is the total mass divided by the volume:ρ = 3Am B4π(128Z me e 2 ) 39π 2 ¯h 2 = 4Am BZx o 3( 2me e 2π¯h 2 x o) 3.For low densities, the solution approaches the unique solutionφ(x o ) → 144x −3 o . Thus, P ∝ ρ 10/3 , with K given by Eq. (3).Mass-Radius Relation for Degenerate ObjectsThe mass-radius diagram for cold compact objects is shownin the figure: the solid lines are the limiting expressions Eqs(1–3), the dashed line is the full result, for 12 C. The maximumradius configuration has the properties, approximately, of theplanet Jupiter.In the relativistic limit, for radii much smaller than 5000km, the equation of state will deviate from that of a γ = 4/3gas. Electron capture will reduce Y e and the value of the Chandrasekharmass. Therefore, a regime where dM/dρ c < 0 willexist. Such a regime is dynamically unstable. At sufficientlyhigh density, where nuclear forces become important, the effectivevalue of γ will increase, the mass will reach a minimumvalue (M min ≃ 0.01 M ⊙ , where R ≃ 300 km), and stability isrestored. As the central density increases further, dM/dρ c > 0.This is the neutron star regime. As the mass increases, and theradius shrinks, general relativity, which we have heretofore ignored,becomes important. The most important feature thatgeneral relativity introduces is that at densities well in excess of

7the nuclear saturation density, ρ s = 2.7 · 10 14 g cm −3 , the massreaches a maximum value, in the range 1.5-3 M ⊙ . Larger densityconfigurations are once again dynamically unstable. Themaximum mass is discussed in a subsequent lecture.Cooling of white dwarfsThe interior of a white dwarf has energy transport dominatedby conduction. The electrons are extremely degenerate,nowever, so they must have very large mean free paths. Thethermal conductivity is very high. The temperature gradientmust be rather small. The interior is roughly isothermal. Nearthe surface, isothermality breaks down as the opacity increases.The surface regions are diffusive, with a temperature gradientdTdr = − 3 κρ L4ac T 3 4πr 2.At the high densities, Kramer’s opacity is dominant: κ =κ o ρT −3.5 , with κ o ≃ 4.3 × 10 24 Z(1 + X) cm 2 g −1 . With hydrostaticequilibrium,dPdT = 4ac34πGm (r) T 6/5κ o L ρ .

8The surface layer is thin, so m(r) = M. Using the nondegeneratepressure P = N o ρkT/µ, and eliminating ρ, we haveP dP = 4ac 4πGM kN o3 κ o L µ T 7.5 dT.Integrating from P = 0 at T = 0 to the interior,√2 4ac 4πGM µρ =T 3.25 .8.5 3 κ o L kN oThe surface approximation breaks down in the interior whenmatter becomes degenerate. This occurs when the non-degeneratepressure equals the degenerate pressure at radius r ∗ where onehas ρ ∗ and T ∗ . This results inρ ∗ = 2/4 × 10 −8 T∗3/2 Ye −1 g cm −3 ,L = 5.7 × 10 5 µYe2 MT∗ 3.5 ergs −1 .Z (1 + X) M ⊙This is similar to the blackbody law L = 4πR 2 σTeff 4 , but involvesthe interior temperature, not the visible temperature ofthe surface. It suggests a relation like T eff ∝ T∗7/8 . From L andM, and the composition, one can deduce T ∗ . L of 10 −2 − 10 −5L ⊙ imply T ∗ = 10 6 − 10 7 K, ρ ∗ < 10 3 g cm −3 andR − r ∗R≃ 4.25RN okT ∗GMµ < ∼ 10 −2 .The energy that is radiated as thermal energy by the whitedwarf is the residual ion thermal energy, since the electrons aredegenerate and the star can’t release any gravitational energy.For a monatomic non-degenerate ion gas, with c v = 3k/2 thetotal thermal energy is (with T ∗ = T )U = 3 N o k2 A T M.

9The cooling rate is L = −dU/dt. Using L = CMT 7/2 ,t = t o + 3 kN( oT −5/2 − T −5/2 )o .5 ACTaking T