Vestibular UFBA â 2006 â2a FASE. Resolução da prova de ...
Vestibular UFBA â 2006 â2a FASE. Resolução da prova de ...
Vestibular UFBA â 2006 â2a FASE. Resolução da prova de ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
<strong>Vestibular</strong> <strong>UFBA</strong> – <strong>2006</strong> –2 a <strong>FASE</strong>.<br />
Resolução <strong>da</strong> <strong>prova</strong> <strong>de</strong> Matemática<br />
Por Profa. Maria Antônia Conceição Gouveia.<br />
Questão 01 (Valor: 15 pontos)<br />
Consi<strong>de</strong>re a equação, na variável x, ax 2 + bx + c = 0 e a função quadrática<br />
f(x) = ax 2 + bx + c, com a, b, e c números reais.<br />
Sabendo que uma raiz <strong>da</strong> equação ax 2 + bx + c = 0 é o número complexo <strong>de</strong> módulo 2<br />
π<br />
e argumento e que a imagem <strong>da</strong> função f(x) = ax 2 + bx + c é o intervalo ] – ∞, –1]<br />
4<br />
calcule f(2).<br />
RESOLUÇÃO:<br />
⎛<br />
z = 2 ⎜cos<br />
⎝<br />
π<br />
4<br />
π ⎞ ⎛ 2 2 ⎞<br />
+ isen ⎟ = 2 ⎜ i⎟<br />
= 1+<br />
i<br />
4 ⎠ +<br />
2 2<br />
⇒ f(x) = a[x – (1 – i)][x- (1 + i)] ⇒<br />
⎝ ⎠<br />
f(x) = a( x 2 – 2 x +2) = ax 2 – 2a x +2a.<br />
Sendo a imagem <strong>da</strong> função f(x) = ax 2 – 2a x +2a o intervalo ] – ∞, –1] então<br />
2<br />
− ∆ − (4a − 8a<br />
=<br />
4a 4a<br />
2<br />
)<br />
= −1⇒<br />
a = −1⇒<br />
f(x) = –x 2 + 2x – 2 ⇒ f(2) = –4 + 4 – 2 = – 2.<br />
Questão 02 (Valor: 15 pontos)<br />
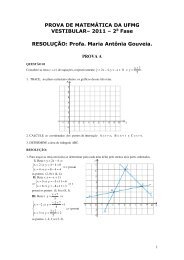
y<br />
5<br />
O gráfico representa a função f: R→]1,+∞[; f(x) =<br />
a+b.2 kx , sendo a, b, e k constantes reais. A partir<br />
<strong>de</strong>ssas informações, calcule f –1 (x).<br />
3<br />
1<br />
-1 0<br />
x<br />
RESOLUÇÃO:<br />
Analisando o gráfico <strong>da</strong> função f(x) = a+b.2 kx , concluímos que é uma translação do gráfico<br />
<strong>da</strong> função h(x) = b.2 kx , segundo o vetor u = 1 ⇒ a = 1.<br />
Logo f(x) = 1+b.2 kx .
Ain<strong>da</strong> pelo gráfico vemos que f(0) = 3 e f(–1) = 5 ⇒<br />
⎧1<br />
+ b = 3 ⎧b<br />
= 2 ⎧b<br />
= 2 ⎧b<br />
= 2 ⎧b<br />
= 2<br />
⎨<br />
⇒ ⎨<br />
⇒ ⎨ ⇒ ⎨ ⇒ ⎨<br />
⎩ +<br />
− k<br />
−k<br />
−k<br />
1 b.2 = 5 ⎩1<br />
+ b.2 = 5 ⎩2.2<br />
= 4 ⎩−<br />
k + 1 = 2 ⎩k<br />
= − 1<br />
f(x) = 1+2.2 –x = 1+2 –x+1 .<br />
Se (x,y) é um par or<strong>de</strong>nado que satisfaz a f(x), então o par (y,x) satisfaz a f –1 (x) ⇒<br />
x = 1+2 –y+1 ⇒ 2 –y+1 =x – 1 ⇒ –y + 1 = log 2<br />
(x − 1) ⇒ y= – log 2<br />
(x − 1) +1⇒<br />
f –1 (x) = – log 2<br />
(x − 1) +1.<br />
Questão 03 (Valor: 15 pontos)<br />
Consi<strong>de</strong>rando, no plano cartesiano, os pontos A(x, 0), B(1, 0) e C(4, 0), <strong>de</strong>termine todos os<br />
valores <strong>de</strong> x para os quais a soma <strong>da</strong> distância <strong>de</strong> A a B e <strong>da</strong> distância <strong>de</strong> A a C seja menor ou<br />
igual a 7.<br />
RESOLUÇÃO:<br />
Distância <strong>de</strong> A a B: d = ( −1) 2 = ( x −1)<br />
x .<br />
Distância <strong>de</strong> A a C: d 1 = ( − 4) 2 = ( x − 4)<br />
x .<br />
Pelos <strong>da</strong>dos do problema: ( x −1)<br />
+ ( − 4)<br />
x ≤ 7<br />
⎧x<br />
−1,<br />
se x ≥ 1<br />
⎨<br />
⎩−<br />
(x −1),<br />
se x < 1<br />
Por <strong>de</strong>finição <strong>de</strong> módulo: ( x −1)<br />
=<br />
e ( x − 4)<br />
⎧x<br />
− 4, se x ≥ 4<br />
= ⎨<br />
⎩−<br />
(x − 4), se x < 4<br />
⇒<br />
1 4<br />
– x + 1 + (– x + 4) ≤ 7<br />
– 2x ≤ 2<br />
x ≥ –1<br />
S 1 = [–1,1[<br />
x – 1 + (– x + 4) ≤ 7<br />
0 ≤ 4 ( V )<br />
S 2 = [1,4[<br />
x – 1 + x – 4 ≤ 7<br />
2x ≤ 12<br />
x ≤ 6<br />
S 3 = [4,6]<br />
S = {x ∈ (S 1 ∪ S 2 ∪ S 3 )} = [–1,6].<br />
Questão 04 (Valor: 15 pontos)<br />
Em uma certa época, uma epi<strong>de</strong>mia atingiu <strong>de</strong>termina<strong>da</strong> região. A fim <strong>de</strong> combater a doença, a<br />
população local foi dividi<strong>da</strong> em três grupos, por faixa etária, e to<strong>da</strong>s as pessoas foram vacina<strong>da</strong>s,<br />
ca<strong>da</strong> uma recebendo a dose <strong>da</strong> vacina <strong>de</strong> acordo com o especificado no quadro a seguir.
Grupo Faixa etária 1 a aplicação 2 a aplicação 3 a aplicação<br />
I<br />
até 15 anos<br />
1ml<br />
2ml<br />
3ml<br />
II<br />
<strong>de</strong> 16 a 59 anos<br />
3ml<br />
2ml<br />
1ml<br />
III<br />
a partir dos 60 anos<br />
5ml<br />
2ml<br />
1ml<br />
Consi<strong>de</strong>rando que, na primeira aplicação, foram gastos 800000ml <strong>da</strong> vacina, na segun<strong>da</strong>,<br />
600000ml e, na terceira, 500000ml, calcule o número <strong>de</strong> pessoas <strong>de</strong> ca<strong>da</strong> grupo.<br />
RESOLUÇÃO:<br />
Consi<strong>de</strong>remos como x o número <strong>de</strong> pessoas até 15 anos, y o <strong>de</strong> pessoas <strong>de</strong> 16 a 59 anos e z<br />
o <strong>de</strong> pessoas com i<strong>da</strong><strong>de</strong> a partir dos 60 anos.<br />
Temos então o sistema:<br />
⎧x<br />
+ 3y + 5z = 80<br />
⎪<br />
⎨2x<br />
+ 2y + 2z = 60 → 2L<br />
1<br />
− L<br />
⎪<br />
⎩3x<br />
+ y + z = 50 → 3L1<br />
− L3<br />
2<br />
⎧x<br />
+ 3y + 5z = 80<br />
⎪<br />
⇒ ⎨4y<br />
+ 8z = 100<br />
⎪<br />
⎩8y<br />
+ 14z = 190 → 2L<br />
2<br />
− L<br />
3<br />
⎧x<br />
+ 3y + 5z = 80<br />
⎪<br />
4y + 8z = 100<br />
⇒ ⎨<br />
⎪2z<br />
= 10<br />
⎪<br />
⎩z<br />
= 5<br />
Em 4y + 8z = 100 substituindo z por 5.<br />
⎧x<br />
+ 3y + 5z = 80<br />
⎪<br />
⎪<br />
4y + 40 = 100<br />
⎨4y<br />
= 60 Em L<br />
⎪y<br />
= 15<br />
⎪<br />
⎪⎩<br />
z = 5<br />
1<br />
substituindo y por 15 e z por<br />
⎧x<br />
+ 3y + 5z = 80<br />
⎪<br />
⎪<br />
x + 45 + 25 = 80<br />
5 ⇒ ⎨x<br />
= 10<br />
⎪y<br />
= 15<br />
⎪<br />
⎪⎩<br />
z = 5<br />
Os valores pedidos são respectivamente 100000, 150000 e 50000 pessoas.<br />
Questão 05 (Valor: 20 pontos)<br />
As medi<strong>da</strong>s dos lados <strong>de</strong> um triângulo ABC formam uma progressão aritmética <strong>de</strong> razão igual a 1.<br />
Determine a altura do triângulo ABC, relativa ao lado AB, sabendo que<br />
3<br />
cos(A BˆC) = .<br />
5<br />
AC < AB < BC e
RESOLUÇÃO:<br />
3<br />
Sendo cos(A BˆC) = , no triângulo retângulo BHC<br />
5<br />
5x, BH = 3x e CH = 4x.<br />
BH 3 = então po<strong>de</strong>mos consi<strong>de</strong>rar BC =<br />
BC 5<br />
Como as medi<strong>da</strong>s AC< AB < BC formam nesta or<strong>de</strong>m uma progressão aritmética <strong>de</strong> razão 1, AB<br />
= BC – 1 e AC= AB – 1, logo sendo BC = 5x, temos AB = 5x – 1 e<br />
AC = 5x – 2 .<br />
Aplicando o Teorema <strong>de</strong> Pitágoras ao triângulo retângulo AHC <strong>da</strong> figura acima:<br />
2<br />
2 2<br />
2<br />
2<br />
2<br />
( 5x − 2) = ( 2x −1) + 16x ⇒ 25x − 20x + 4 = 4x − 4x + 1+<br />
16x<br />
16 ± 256 − 60 16 ± 14<br />
5x 2 − 16x + 3 = 0 ⇒ x =<br />
= ⇒ x = 3 ou x = 0,2<br />
10 10<br />
Para x = 3, AB = 14 e para x = 0,2, AB = 0 ( impossível).<br />
Logo x = 3 e a altura procura<strong>da</strong> que é 4x = 12.<br />
Questão 06 (Valor: 20 pontos)<br />
Consi<strong>de</strong>re a reta r, que tem como equação y = 1, e a reta s, que passa pelos pontos A(4, −3) e<br />
B(2, 0).<br />
Sendo M a região do plano limita<strong>da</strong> pelos eixos coor<strong>de</strong>nados cartesianos Ox e Oy e pelas retas r<br />
e s, calcule o volume do sólido obtido pela rotação <strong>da</strong> região M em torno do eixo Oy.<br />
RESOLUÇÃO<br />
Consi<strong>de</strong>re a reta r, que tem como equação y = 1, e a reta s, que passa pelos pontos A(4, −3) e<br />
B(2, 0).
Sendo M a região do plano limita<strong>da</strong> pelos eixos coor<strong>de</strong>nados cartesianos Ox e Oy e pelas retas r<br />
e s, calcule o volume do sólido obtido pela rotação <strong>da</strong> região M em torno do eixo Oy.<br />
Po<strong>de</strong>mos obter a reta s que passa pelos pontos A(4, −3) e B(2, 0) <strong>da</strong> seguinte forma:<br />
4<br />
− 3<br />
2<br />
0<br />
x<br />
y<br />
4<br />
= 0 ⇒<br />
− 3<br />
2y − 3x − 4y + 6<br />
3<br />
= 0 ⇒ 2y = −3x<br />
+ 6 ⇒ y = − x + 3<br />
2<br />
A reta s: y = 3 − x + 3 cuja raiz é x = 2.<br />
2<br />
A interseção entre as retas s: y = 1 e r: 3 − x + 3 = 1 é <strong>da</strong><strong>da</strong> pela solução do sistema<br />
2<br />
⎧y<br />
= 1<br />
⎪<br />
⎨ 3 ⇒ 3x = 4 ⇒ x = 4/3.<br />
⎪y<br />
= − x + 3<br />
⎩ 2<br />
A área M que está representa<strong>da</strong> na figura é um trapézio <strong>de</strong> altura 1 e bases 2 e 4/3, respectivamente.<br />
O sólido gerado pela rotação <strong>da</strong> região M em torno do eixo Oy é um tronco <strong>de</strong> cone cujo volume é a<br />
diferença entre os volumes dos cone ACB e DCE que são semelhantes.<br />
x 4/3<br />
Calculemos a altura x do cone DCE: = ⇒ 6x = 4x + 4 ⇒ x = 2 .<br />
x + 1 2<br />
O volume procurado é:<br />
V =<br />
1 1 16 12π<br />
32π<br />
108π<br />
− 32π<br />
76<br />
.4π .3 − . π.2<br />
= − =<br />
=<br />
π u.v.<br />
3 3 9 3 27 27 27