Sossinsky:Knots. Mathematics with a twist.pdf - English

6 KNOTS
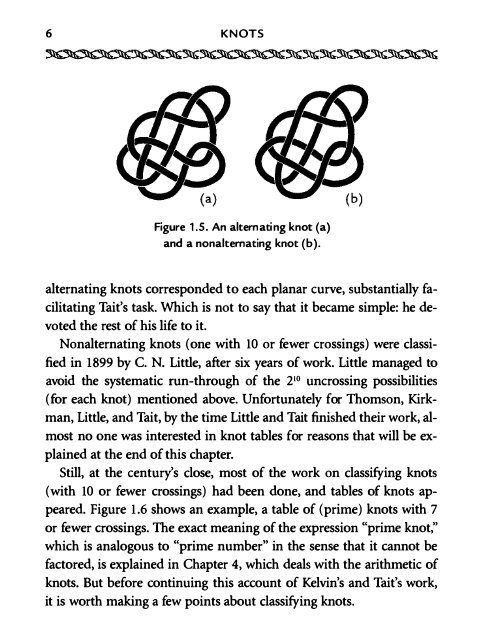
Figure 1.5. An altemating knot (a)
and a nonaltemating knot (b).
alternating knots corresponded to each planar curve, substantially fa­
cilitating Tait's task. Which is not to say that it became simple: he de­
voted the rest of his life to it.
Nonalternating knots (one with lO or fe wer crossings) were dassi­
fied in 1899 by C. N. Little, after six years of work. Little managed to
avoid the systematic run-through of the 210 uncrossing possibilities
(for each knot) mentioned above. Unfortunately for Thomson, Kirk­
man, Little, and Tait, by the time Little and Tait finished their work, al­
most no one was interested in knot tables for reasons that will be ex­
plained at the end of this chapter.
Still, at the century's dose, most of the work on dassifying knots
(with lO or fewer crossings) had been done, and tables of knots appeared.
Figure l.6 shows an example, a table of (prime) knots with 7
or fe wer crossings. The exact meaning of the expression "prime knot:'
which is analogous to "prime number" in the sense that it cannot be
factored, is explained in Chapter 4, which deals with the arithmetic of
knots. Sut before continuing this account of Kelvin's and Tait's work,
it is worth making a few points about dassifying knots.