The Friedrichs Extension of Singular Differential Operators

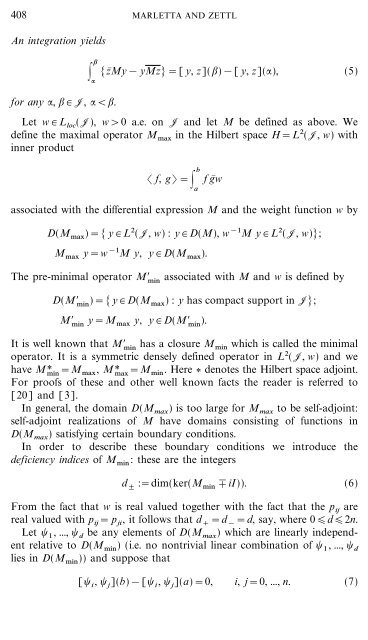
408 MARLETTA AND ZETTL
An integration yields
| ;
:
for any :, ; # J, :0 a.e. on J and let M be defined as above. We
define the maximal operator M max in the Hilbert space H=L 2 (J, w) with
inner product
(f, g)=| b
fgw
associated with the differential expression M and the weight function w by
D(M max)=[y# L 2 (J, w) :y # D(M), w &1 My# L 2 (J, w)];
M max y=w &1 My, y # D(M max).
The pre-minimal operator M$ min associated with M and w is defined by
D(M$ min)=[y# D(M max) :y has compact support in J];
M$ min y=M max y, y # D(M$ min ).
It is well known that M$ min has a closure M min which is called the minimal
operator. It is a symmetric densely defined operator in L 2 (J, w) and we
have M* min =M max, M* max =M min. Here V denotes the Hilbert space adjoint.
For proofs of these and other well known facts the reader is referred to
[20] and [3].
In general, the domain D(M max) is too large for M max to be self-adjoint:
self-adjoint realizations of M have domains consisting of functions in
D(M max) satisfying certain boundary conditions.
In order to describe these boundary conditions we introduce the
deficiency indices of M min: these are the integers
a
d \ :=dim(ker(M min iI)). (6)
From the fact that w is real valued together with the fact that the p ij are
real valued with p ij= p ji, it follows that d +=d &=d, say, where 0 d 2n.
Let 1, ..., d be any elements of D(M max) which are linearly independent
relative to D(M min) (i.e. no nontrivial linear combination of 1, ..., d
lies in D(M min)) and suppose that
[ i, j](b)&[ i, j](a)=0, i, j=0, ..., n. (7)