Open Learning Jan-June -2010 - An Awareness Magazine

eqDr Open f'k{kk Learning January-June tuojh&twu 2010
Corollary
The circum-centre, centroid and orthocenter of a nonequilateral
triangle are collinear.
Let S be the circum-centre and O be the orthocenter
of the triangle ABC which is non-equilateral and A'
be the mid-point of BC (Fig. 3). SA, SO and AA'
are joined. AA' cuts OS at G. To show that O, G
and S are collinear.
A
B D A' C
Fig. 3
Proof: Let H and K be the mid-points of AG and
OG respectively. HS, KA' and HK are joined.
Now, HK || AO and HK = AO/2.
Then, HK || SA' and HK = SA' (since by theorem
2, SA' = AO/2).
Therefore HKA'S is a parallelogram.
Now, diagonals of a parallelogram bisect each
other.
Comments
(i)
The line OS is called the Euler line of the
triangle ABC.
(ii) For an equilateral triangle O, G and S
coincide.
We know that the circum-centre of a right-angled
triangle lies at the mid-point of its hypotenuse.
For this reason, one can get the following
beautiful relation between the circum-radius and
in-radius of a right-angled triangle stated in the
following theorem.
Theorem 3
The sum of the circum-radius and in-radius of a
right-angled triangle is half the sum of the sides
containing right angle.
Proof: The detail proof of the theorem is left to
the readers.
Let us terminate this article with a discussion on
the nine-point circle. We shall see that the ninepoint
circle passes through particular nine points
related to a triangle.
Theorem 4
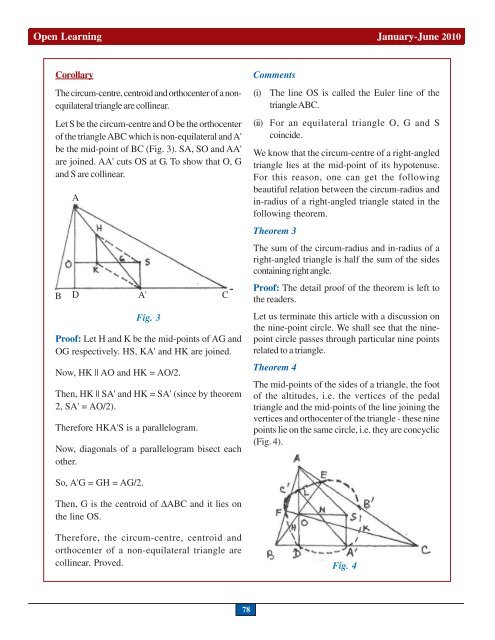
The mid-points of the sides of a triangle, the foot
of the altitudes, i.e. the vertices of the pedal
triangle and the mid-points of the line joining the
vertices and orthocenter of the triangle - these nine
points lie on the same circle, i.e. they are concyclic
(Fig. 4).
So, A'G = GH = AG/2.
Then, G is the centroid of ΔABC and it lies on
the line OS.
Therefore, the circum-centre, centroid and
orthocenter of a non-equilateral triangle are
collinear. Proved.
Fig. 4
78