Vol. 8 No 7 - Pi Mu Epsilon

edition, p. 43). Here we will present a somewhat unusual proof,
deriving the formula from Simpson's Rule which is usually used for
approximating integrals and not for deriving exact formulas. Our proof
also suggests why the formula cannot be extended to the situation in
which A(x) is a polynomial of degree larger than three.
Before proving the theorem, we will give several examples of how
the formula works.
Example. 1. If we place our x-axis so that it is perpendicular to
the base of a rectangular box (Figure 1) then the cross-sectional area
is a rectangle and A(x) = -&i>.
If we take h = b - a, we have
V = (h/6)(£ + 4to + to) = (h/6)(6to) = &*.
of x. Also, b - a = 2r. Thus, the volume of a sphere of radius r is
2 3
V = (2~/6)(0 + 4nr + 0) = (4/3)(irr )
since the cross-sectional area of each end of the sphere is 0 and in the
2 * .-
middle is m .
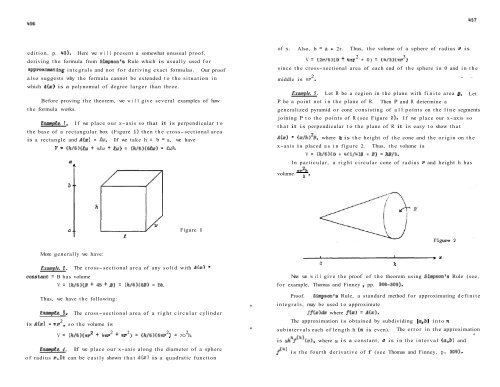
Example. 5. Let R be a region in the plane with finite area B. Let
P be a point not in the plane of R.
Then P and R determine a
generalized pyramid or cone consisting of all points on the line segments
joining P to the points of R (see Figure 2). If we place our x-axis so
that it is perpendicular to the plane of R it is easy to show that
A(x) = (z/h)~, where h is the height of the cone and the origin on the
x-axis is placed as in figure 2.
V = (h/6)(0 + 4(1/4)B t B) = hB/3.
Thus, the volume is
In particular, a right circular cone of radius r and height h has
nrLh
volume 3 -
Figure 1
More generally we have:
Example. 2. The cross-sectional area of any solid with A(x) =
constant = B has volume
V = (h/6)(B + 4B + B) = (h/6)(6B) = Bh.
Thus, we have the following:
EW&C 3' The cross-sectional area of a right circular cylinder
2
is A(x) = try , so the volume is
2 2 2 2
V = (h/6)(w2 + 4nr + nr ) = (h/6)(6nr ) = TO h.
E ~ p 4. h If we place our x-axis along the diameter of a sphere
of radius r,it can be easily shown that A(x) is a quadratic function
Now we will give the proof of the theorem using Simpson's Rule (see,
for example, Thomas and Finney , pp. 308-309).
Proof. Simpson's Rule, a standard method for approximating definite
integrals, may be used to approximate
ff(x)dx where f(x) = A(x).
The approximation is obtained by subdividing [a,b] into n
subintervals each of length h (n is even). The error in the approximation
is vh4f4(c), where is a constant, c is in the interval (a,b) and
f(4) is the fourth derivative of f (see Thomas and Finney, p. 309).
A