COMPLEX FUNCTIONS Contents 1. Complex numbers, Cauchy ...

COMPLEX FUNCTIONS 7
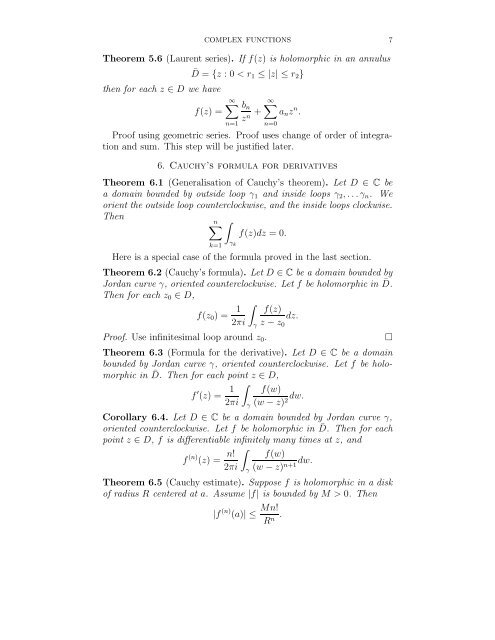
Theorem 5.6 (Laurent series). If f(z) is holomorphic in an annulus
¯D = {z : 0 < r 1 ≤ |z| ≤ r 2 }
then for each z ∈ D we have
f(z) =
∞∑ b n
z + ∑ ∞
a n n z n .
n=1
Proof using geometric series. Proof uses change of order of integration
and sum. This step will be justified later.
n=0
6. Cauchy’s formula for derivatives
Theorem 6.1 (Generalisation of Cauchy’s theorem). Let D ∈ C be
a domain bounded by outside loop γ 1 and inside loops γ 2 , . . . γ n . We
orient the outside loop counterclockwise, and the inside loops clockwise.
Then
n∑
∫
f(z)dz = 0.
γ k
k=1
Here is a special case of the formula proved in the last section.
Theorem 6.2 (Cauchy’s formula). Let D ∈ C be a domain bounded by
Jordan curve γ, oriented counterclockwise. Let f be holomorphic in ¯D.
Then for each z 0 ∈ D,
f(z 0 ) = 1 ∫
2πi γ
Proof. Use infinitesimal loop around z 0 .
f(z)
z − z 0
dz.
Theorem 6.3 (Formula for the derivative). Let D ∈ C be a domain
bounded by Jordan curve γ, oriented counterclockwise. Let f be holomorphic
in ¯D. Then for each point z ∈ D,
f ′ (z) = 1
2πi
∫
γ
f(w)
(w − z) 2 dw.
Corollary 6.4. Let D ∈ C be a domain bounded by Jordan curve γ,
oriented counterclockwise. Let f be holomorphic in ¯D. Then for each
point z ∈ D, f is differentiable infinitely many times at z, and
f (n) (z) = n!
2πi
∫
γ
f(w)
dw.
(w − z)
n+1
Theorem 6.5 (Cauchy estimate). Suppose f is holomorphic in a disk
of radius R centered at a. Assume |f| is bounded by M > 0. Then
|f (n) (a)| ≤ Mn!
R n .
□