DUAL INTEGRAL EQUATIONS INVOLVING LEGENDRE ...
DUAL INTEGRAL EQUATIONS INVOLVING LEGENDRE ...
DUAL INTEGRAL EQUATIONS INVOLVING LEGENDRE ...
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
54 P. K. BANERJI, DESHNA LOONKER<br />
(<br />
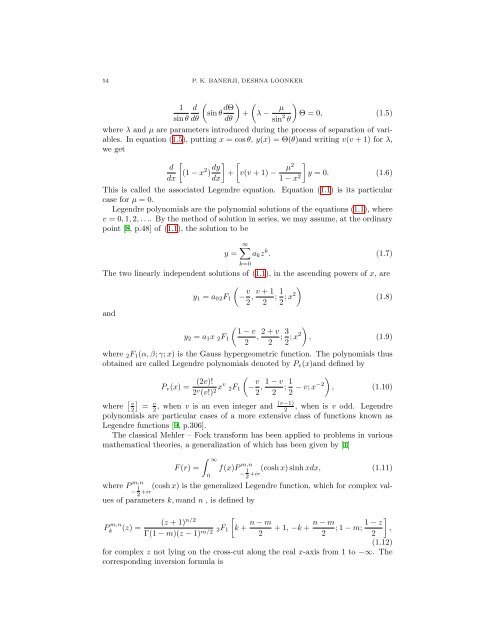
1 d<br />
sin θ dΘ ) (<br />
+ λ − µ<br />
sin θ dθ dθ<br />
)<br />
Θ = 0, (1.5)<br />
sin 2 θ<br />
where λ and µ are parameters introduced during the process of separation of variables.<br />
In equation (1.5), putting x = cos θ, y(x) = Θ(θ)and writing v(v + 1) for λ,<br />
we get<br />
[<br />
d<br />
(1 − x 2 ) dy ]<br />
]<br />
+<br />
[v(v + 1) − µ2<br />
dx dx<br />
1 − x 2 y = 0. (1.6)<br />
This is called the associated Legendre equation. Equation (1.1) is its particular<br />
case for µ = 0.<br />
Legendre polynomials are the polynomial solutions of the equations (1.1), where<br />
v = 0, 1, 2, . . .. By the method of solution in series, we may assume, at the ordinary<br />
point [8, p.48] of (1.1), the solution to be<br />
y =<br />
∞∑<br />
a k z k . (1.7)<br />
k=0<br />
The two linearly independent solutions of (1.1), in the ascending powers of x, are<br />
and<br />
y 1 = a 02 F 1<br />
(<br />
− v 2 , v + 1<br />
2 ; 1 2 ; x2 )<br />
(1.8)<br />
y 2 = a 1 x 2 F 1<br />
( 1 − v<br />
2 , 2 + v<br />
2 ; 3 2 ; x2 )<br />
, (1.9)<br />
where 2 F 1 (α, β; γ; x) is the Gauss hypergeometric function. The polynomials thus<br />
obtained are called Legendre polynomials denoted by P v (x)and defined by<br />
P v (x) =<br />
(2v)! (<br />
2 v (v!) 2 xv 2F 1 − v 2 , 1 − v<br />
2 ; 1 )<br />
2 − v; x−2 , (1.10)<br />
where [ ]<br />
v<br />
2 =<br />
v<br />
(v−1)<br />
2<br />
, when v is an even integer and<br />
2<br />
, when is v odd. Legendre<br />
polynomials are particular cases of a more extensive class of functions known as<br />
Legendre functions [9, p.306].<br />
The classical Mehler – Fock transform has been applied to problems in various<br />
mathematical theories, a generalization of which has been given by [1]<br />
∫ ∞<br />
F (r) = f(x)P m,n<br />
0<br />
− 1 x) sinh xdx, (1.11)<br />
2<br />
+ir(cosh<br />
where P m,n<br />
− 1 x) is the generalized Legendre function, which for complex values<br />
of parameters k, mand n , is defined<br />
2<br />
+ir(cosh<br />
by<br />
P m,n<br />
k<br />
(z) =<br />
(z + 1) n/2 [<br />
Γ(1 − m)(z − 1) m/2 2 F 1 k + n − m<br />
2<br />
+ 1, −k + n − m ; 1 − m; 1 − z<br />
2<br />
2<br />
(1.12)<br />
for complex z not lying on the cross-cut along the real x-axis from 1 to −∞. The<br />
corresponding inversion formula is<br />
]<br />
,