Lecture Notes Topology (2301631) Phichet Chaoha Department of ...

18 Topology (2301631)
Remark 1.62. Definition 1.59 is generally known as the local definition of
continuity while Theorem 1.61 is usually taken to be the global definition. Moreover,
using the global definition, one can easily prove that f : X → Y is continuous iff
f −1 (C) is closed in X for each closed subset C of Y .
Example 1.63. Let τ and τ ′ be topologies on X. It is easy to verify that the
identity map 1 X : (X, τ) → (X, τ ′ ) is always continuous iff τ is finer than τ ′ .
Remark 1.64. If the topology of Y is generated by a basis B, it is easy to see
that a map f : X → Y is continuous iff f −1 (B) is open for each B ∈ B. This is
because f −1 ( ∪ α B α) = ∪ α f −1 (B α ). Note also that f −1 (B) may not be a basis
element of X.
Exercise 1.65. If the topology of Y is generated by a subbasis S, prove that
a map f : X → Y is continuous iff f −1 (S) is open in X for each S ∈ S.
Theorem 1.66. A map f : X → Y is continuous iff f(A) ⊆ f(A) for any
subset A of X.
Proof. (⇒) Let A ⊆ X, y ∈ f(A) and V be a neighborhood of y. Then, there
exists x ∈ A such that f(x) = y. Hence, x ∈ f −1 (V ). By the continuity of f, it
follows that f −1 (V ) is a neighborhood of x. Therefore, f −1 (V ) ∩ A ≠ ∅ and hence
∅ ̸= f(f −1 (V ) ∩ A) ⊆ f(f −1 (V )) ∩ f(A) ⊆ V ∩ f(A).
This implies y ∈ f(A).
(⇐) Let C be a closed subset of Y and A = f −1 (C). Since
f(A) ⊆ f(A) = f(f −1 (C)) ⊆ C = C,
then we have A ⊆ f −1 (f(A)) ⊆ f −1 (C) = A.
desired.
Therefore, A is closed in X as
□
Example 1.67. It is easy to verify that a constant map, an inclusion and a
restriction of a continuous map are continuous.
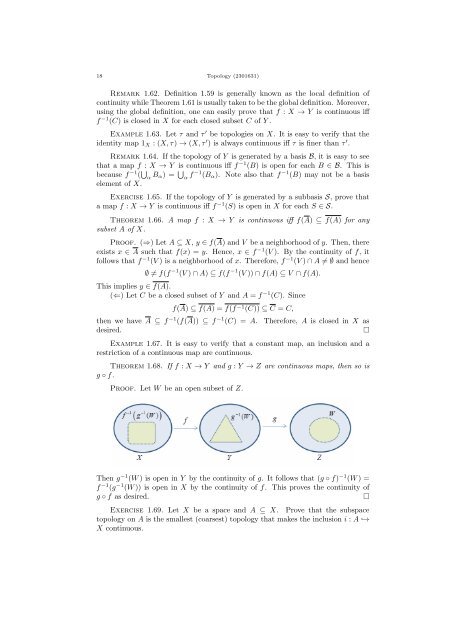
Theorem 1.68. If f : X → Y and g : Y → Z are continuous maps, then so is
g ◦ f.
Proof. Let W be an open subset of Z.
Then g −1 (W ) is open in Y by the continuity of g. It follows that (g ◦ f) −1 (W ) =
f −1 (g −1 (W )) is open in X by the continuity of f. This proves the continuity of
g ◦ f as desired.
□
Exercise 1.69. Let X be a space and A ⊆ X. Prove that the subspace
topology on A is the smallest (coarsest) topology that makes the inclusion i : A ↩→
X continuous.