View - Jean-Pierre QUADRAT - Free

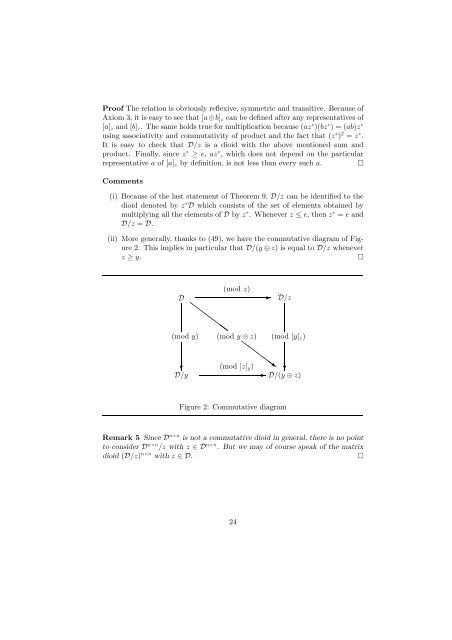
Proof The relation is obviously reflexive, symmetric and transitive. Because ofAxiom 3, it is easy to see that [a⊕b] z can be defined after any representatives of[a] z and [b] z . The same holds true for multiplication because (az ∗ )(bz ∗ )=(ab)z ∗using associativity and commutativity of product and the fact that (z ∗ ) 2 = z ∗ .It is easy to check that D/z is a dioid with the above mentioned sum andproduct. Finally, since z ∗ ≥ e, az ∗ , which does not depend on the particularrepresentative a of [a] z by definition, is not less than every such a. □Comments(i) Because of the last statement of Theorem 9, D/z can be identified to thedioid denoted by z ∗ D which consists of the set of elements obtained bymultiplying all the elements of D by z ∗ . Whenever z ≤ e, then z ∗ = e andD/z = D.(ii) More generally, thanks to (49), we have the commutative diagram of Figure2. This implies in particular that D/(y ⊕ z) is equal to D/z wheneverz ≥ y.□D(mod z)✲D/z❩ ❩❩❩❩(mod y) (mod y ⊕ z) (mod [y] z )❩ ❩❩❩❩7❄D/y(mod [z] y )❄✲ D/(y ⊕ z)Figure 2: Commutative diagramRemark 5 Since D n×n is not a commutative dioid in general, there is no pointto consider D n×n /z with z ∈D n×n . But we may of course speak of the matrixdioid (D/z) n×n with z ∈D.□24