Sequences and Series

More documents

Recommendations

Info

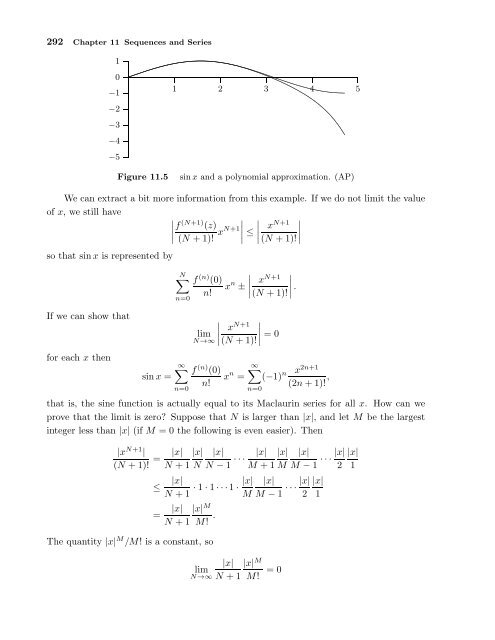
292 Chapter 11 Sequences and Series10−1−2−3−4−51 2 3 4 5..Figure 11.5 sinx and a polynomial approximation. (AP)We can extract a bit more information from this example. If we do not limit the valueof x, we still have∣ f (N+1) (z) ∣∣∣ ∣ (N +1)! xN+1 ≤x N+1∣(N +1)! ∣so that sinx is represented byIf we can show thatfor each x thensinx =N∑n=0∞∑n=0f (n) (0)n!limN→∞f (n) (0)n!x n ±x N+1∣(N +1)! ∣ .x N+1∣(N +1)! ∣ = 0x n =∞∑n=0(−1) n x2n+1(2n+1)! ,that is, the sine function is actually equal to its Maclaurin series for all x. How can weprove that the limit is zero? Suppose that N is larger than |x|, and let M be the largestinteger less than |x| (if M = 0 the following is even easier). Then|x N+1 |(N +1)! = |x| |x|N +1 N|x|N −1 ··· |x| |x|M +1 M|x| |x| |x| ···M −1 2 1≤ |x| |x| |x| |x| |x|·1·1···1· ···N +1 M M −1 2 1= |x| |x| MN +1 M! .The quantity |x| M /M! is a constant, solimN→∞|x| |x| MN +1 M!= 0
11.11 Taylor’s Theorem 293and by the Squeeze Theorem (11.3)limN→∞x N+1∣(N +1)! ∣ = 0as desired. Essentially the same argument works for cosx and e x ; unfortunately, it is moredifficult to show that most functions are equal to their Maclaurin series.EXAMPLE 11.54 Find a polynomial approximation for e x near x = 2 accurate to±0.005.From Taylor’s theorem:e x =N∑n=0e 2n! (x−2)n +e z(N +1)! (x−2)N+1 ,since f (n) (x) = e x for all n. We are interested in x near 2, and we need to keep |(x−2) N+1 |in check, so we may as well specify that |x−2| ≤ 1, so x ∈ [1,3]. Alsoe z∣(N +1)! ∣ ≤ e 3(N +1)! ,so we need to find an N that makes e 3 /(N + 1)! ≤ 0.005. This time N = 5 makese 3 /(N +1)! < 0.0015, so the approximating polynomial ise x = e 2 +e 2 (x−2)+ e22 (x−2)2 + e26 (x−2)3 + e224 (x−2)4 + e2120 (x−2)5 ±0.0015.This presents an additional problem for approximation, since we also need to approximatee 2 , and any approximation we use will increase the error, but we will not pursue thiscomplication.Note well that in these examples we found polynomials of a certain accuracy only ona small interval, even though the series for sinx and e x converge for all x; this is typical.To get the same accuracy on a larger interval would require more terms.Exercises 11.11.1. Find a polynomial approximation for cosx on [0,π], accurate to ±10 −3 ⇒2. How many terms of the series for lnx centered at 1 are required so that the guaranteed erroron [1/2,3/2] is at most 10 −3 ? What if the interval is instead [1,3/2]? ⇒3. Find the first three nonzero terms in the Taylor series for tanx on [−π/4,π/4], and computethe guaranteed error term as given by Taylor’s theorem. (You may want to use Sage or asimilar aid.) ⇒
Page 6 and 7: 260 Chapter 11 Sequences and Series
Page 22 and 23: ½½º ×ÓÐÙØÓÒÚÖÒ276 Chap

Sequences and Series

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?