On the approximation of real rational functions via mixed-integer ...

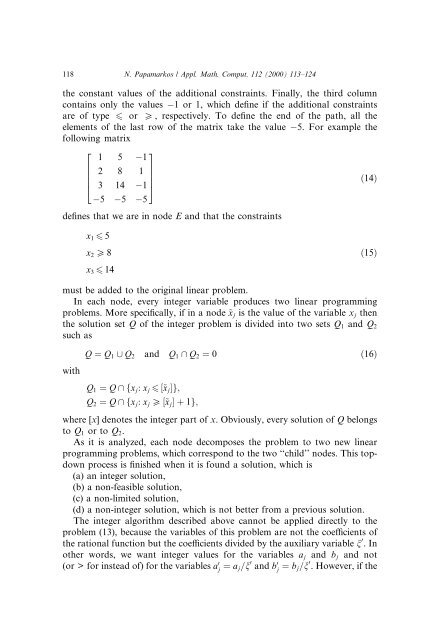
118 N. Papamarkos / Appl. Math. Comput. 112 (2000) 113±124the constant values of the additional constraints. Finally, the third columncontains only the values 1 or 1, which de®ne if the additional constraintsare of type 6 or P , respectively. To de®ne the end of the path, all theelements of the last row of the matrix take the value 5. For example thefollowing matrix231 5 12 8 167…14†4 3 14 1 55 5 5de®nes that we are in node E and that the constraintsx 1 6 5x 2 P 8x 3 6 14…15†must be added to the original linear problem.In each node, every integer variable produces two linear programmingproblems. More speci®cally, if in a node ~x j is the value of the variable x j thenthe solution set Q of the integer problem is divided into two sets Q 1 and Q 2such aswithQ ˆ Q 1 [ Q 2 and Q 1 \ Q 2 ˆ 0 …16†Q 1 ˆ Q \fx j : x j 6 ‰~x j Šg;Q 2 ˆ Q \fx j : x j P ‰~x j Š‡1g;where [x] denotes the integer part of x. Obviously, every solution of Q belongsto Q 1 or to Q 2 .As it is analyzed, each node decomposes the problem to two new linearprogramming problems, which correspond to the two ``child'' nodes. This topdownprocess is ®nished when it is found a solution, which is(a) an integer solution,(b) a non-feasible solution,(c) a non-limited solution,(d) a non-integer solution, which is not better from a previous solution.The integer algorithm described above cannot be applied directly to theproblem (13), because the variables of this problem are not the coecients ofthe rational function but the coecients divided by the auxiliary variable n 0 .Inother words, we want integer values for the variables a j and b j and not(or > for instead of) for the variables a 0 j ˆ a j=n 0 and b 0 j ˆ b j=n 0 . However, if the