Vol. 5 No 10 - Pi Mu Epsilon

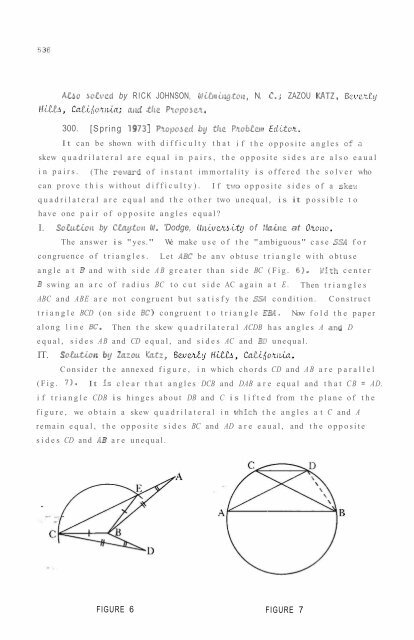
ALAO solved by RICK JOHNSON, Wibnhjton, N. C..; ZAZOU KATZ,HeC.E.s, CatC.&o,z~ua; and the Pzoposc-z.300. [Spring 19731 Ptoposcd bg the. Piobicin EdLto,~.It can be shown with difficulty that if the opposite anglesskew quadrilateral are equal in pairs, the opposite sides are also eaualin pairs. (The reward of instant immortality is offered the solver whocan prove this without difficulty). If t:!o opposite sides of a ske:.!quadrilateral are equal and the other two unequal, is it possible tohave one pair of opposite angles equal?I. SoLtLon by Ctay.tun W. 'Dodge, l.litivt,'t~.Lty of !:la-i,ne. at (h.oiio.The answer is "yes." We make use of the "ambiguous" case SSA forcongruence of triangles. Let ABC be anv obtuse triangle with obtuseangle at B and with side AB greater than side BC (Fig. 6). V!ith centerB swing an arc of radius BC to cut side AC again at E. Then trianglesABC and ABE are not congruent but satisfy the SSA condition. Constructtriangle BCD (on side BC) congruent to triangle EBA. Now fold the paperalong line BC. Then the skew quadrilateral ACDB has angles A and Dequal, sides AB and CD equal, and sides AC and ED unequal.IT. SoL-Uon by Zazou Kats, Bwe~-Cy H,(¥£.Caf-L^o~i~C.a,Consider the annexed figure, in which chords CD and AB are parallel(Fig. 7). It is clear that angles DCB and DAB are equal and that CB = AD.if triangle CDB is hinges about DB and C is lifted from the plane of thefigure, we obtain a skew quadrilateral in vhich the angles at C and Aremain equal, the opposite sides BC and AD are eaual, and the oppositesides CD and AS are unequal.A£i ~otued biy ALFRED E. NEUMAN, (In A£p V&a Fra.tfyi.iM^iy, NwVoftk, and by NOSMO KING, Raieigh, N. C.Probtm Ed.itot14 Note.Readers interested in si'ew quadrilaterals will find solutions ofthe introductory theorem on pages 1026 and 1027 of the November 1973issue of the American Mathematical Monthly~solutions to Question B-5of the William Lowell Futnam Iiathema!ical Compet'tion held on December -,1972).301. [Spring 19731 Proposed by Neat Jacob&, &ionx, N. V.(Lorre=ted). One-fifteent!. can be expressed in "decimals" ir. manvways, for example, as .0421 in base ¥;ight or as ,013 in base five.*hat 'n any base n, the "decima-" for one-fifteenth will have n:, morethan feur recurring digits.Sotu,Uon by Vonn&Uy J. John&on,Fotce. Bae., Oluo...hrwhlajot, USAF, W~Lgd-t-PaAtmc~ A^':The representat is-.n '-'f 1 "15 as a "decimaJ " f;xpressi',n to base ). ,(n = 2, 3, 4, '' ,) either terminates or has a repeating block f :enz-.1, 2, or 4. T pr:ive th:s, 't is suffi~iento show that there i s r -peating block ¥. length 4. Also it is sufficient to show tha^ 1- :2, 3, 4;-', then 15 divide- n(n4 - 1) since ". n(n4 - l)¥'lr = : .-K = f a./nz with 0 2 a . n, then- - 2is seen to represent a number in "decimal" base ti wi" . :length 4 digits; andTcomplete the proof that 15 divid--p = 3 and for p = 5, p divide? e'"ther i ?f~cientto show forp = 3, 5 thatifa- -. .is a multiple of p: thu~., l4 - 1 = : ' -215.A hhoiue.d by RICHARD A. GIBES, - -' -FIGURE 6 FIGURE 7ado; BOB PRIELIPP, The. Uiztvml A -A&? .hot\}~~i noted the. ~tt-L'spti~."& . .