Rearrangement of series. The theorem of Levy-Steiniz. - José Bonet ...
Rearrangement of series. The theorem of Levy-Steiniz. - José Bonet ...
Rearrangement of series. The theorem of Levy-Steiniz. - José Bonet ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
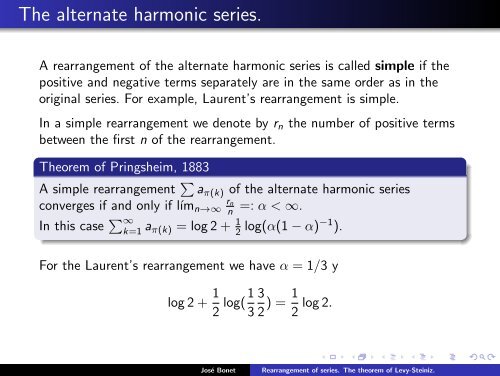
<strong>The</strong> alternate harmonic <strong>series</strong>.<br />
A rearrangement <strong>of</strong> the alternate harmonic <strong>series</strong> is called simple if the<br />
positive and negative terms separately are in the same order as in the<br />
original <strong>series</strong>. For example, Laurent’s rearrangement is simple.<br />
In a simple rearrangement we denote by rn the number <strong>of</strong> positive terms<br />
between the first n <strong>of</strong> the rearrangement.<br />
<strong>The</strong>orem <strong>of</strong> Pringsheim, 1883<br />
A simple rearrangement � aπ(k) <strong>of</strong> the alternate harmonic <strong>series</strong><br />
converges if and only if límn→∞ rn<br />
n =: α < ∞.<br />
In this case �∞ k=1 aπ(k) = log 2 + 1<br />
2 log(α(1 − α)−1 ).<br />
For the Laurent’s rearrangement we have α = 1/3 y<br />
log 2 + 1<br />
2 log(1<br />
3 1<br />
) = log 2.<br />
3 2 2<br />
<strong>José</strong> <strong>Bonet</strong> <strong>Rearrangement</strong> <strong>of</strong> <strong>series</strong>. <strong>The</strong> <strong>theorem</strong> <strong>of</strong> <strong>Levy</strong>-<strong>Steiniz</strong>.