Boîte à outils : La méthode du pivot de Gauss. 1 Vocabulaire ...

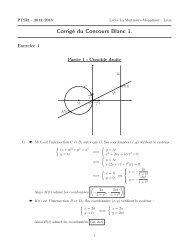
PTSI2 – 2012/2013
Lycée La Martinière-Monplaisir – Lyon
Boîte à outils : La méthode du pivot de Gauss.
C’est une méthode de résolution des systèmes linéaires qui marche à tous les coups.
On notera K = R ou C.
1 Vocabulaire : systèmes linéaires
Voici des exemples de systèmes linéaires :
⎧
⎪⎨
{
x + y = 0
x − y = 1
;
⎪⎩
x + 2y − z = −2
2x − y + z = 0
y + 3z = 5
;
{
x + y − z + t = 0
x = 3z − 4t = 1
;
⎧
⎪⎨
⎪⎩
x + y = 1
x − y = 0
x + 3y = 5
Définition : Soient n et p des entiers naturels non nuls.
• On appelle système linéaire à n équations et p inconnues tout système de la
forme :
⎧
a 1,1 x 1 + a 1,2 x 2 + . . . + a 1,p x p = b 1
⎪⎨ a 2,1 x 1 + a 2,2 x 2 + . . . + a 2,p x p = b 2
(S) :
.
.
.
.
⎪⎩
a n,1 x 1 + a n,2 x 2 + . . . + a n,p x p = b n
où les (a i,j ) 1≤i≤n, 1≤j≤p sont les coefficients (éléments de K = R ou C),
le n-uplet (b 1 , b 2 , . . . , b n ) ∈ K n est le second membre,
les x 1 , x 2 , . . . , x p ∈ K forment le p-uplet des inconnues.
• Par définition, une solution de (S) est un p-uplet (x 1 , x 2 , . . . , x p ) ∈ K p qui
vérifie toutes les équations. Résoudre (S), c’est trouver toutes les solutions.
• Lorsque tous les b i sont nuls, le système est dit homogène. Si (S) n’est pas
homogène, on peut définir le système homogène associé à (S), c’est le système
(H) obtenu en remplaçant tous les b i par des 0.
Remarques :
• Lorsqu’un système admet au moins une solution, il est dit compatible ; s’il n’a aucune solution, il
est dit incompatible.
• Lorsqu’on a un système homogène, (0, . . . , 0) est toujours solution : il est donc compatible.
2 Systèmes triangulaires ou échelonnés
Ce sont des cas particuliers où l’on sait très vite donner l’ensemble des solutions, en "remontant".
⎧
⎪⎨
Exemple 1 Résoudre (S) :
⎪⎩
x + y − z = 2
3y + z = 0
z = 6
1

Exemple 2 Résoudre (S) :
{
x − y + 3z = 2
y − 2z = 5
{
x + y + z + t = 0
Exemple 3 Résoudre (S) :
y − z = i
2

Comment se ramener à un système triangulaire ou échelonné ?
3 Opérations élémentaires sur les lignes
Ce sont les 3 seules opérations autorisées sur les systèmes, car elles seules permettent de garder un
système équivalent au système de départ.
En notant L i la i-ème ligne/équation du système :
• Multiplication de L i par un α ∈ K non nul :
L i ← αL i
• Échange de L i et L j :
L i ↔ L j
• Ajout à L i de la ligne L j multipliée par un β ∈ K (avec i ≠ j) :
L i ← L i + βL j
L
A priori il faut les faire une à la fois : par exemple si on écrit 1 ← L 1 + L 3
, on ne sais pas bien
L 2 ← L 2 − 3L 1
quelle ligne L 1 on utilise pour modifier L 2 : la nouvelle ou l’ancienne ? Il faut mettre deux étapes car
cela peut mener à un système faux.
Par contre, on peut faire L 2 ← L 2 + L 1
L 3 ← L 3 − 3L 1
4 Méthode du pivot de Gauss
par exemple, car il n’y a pas d’ambiguïté.
Elle dit quelles opérations élémentaires effectuer pour obtenir à coup sûr un système triangulaire ou
échelonné !
blablablablablablaMéthodeblablablablablabla
On suppose a 1,1 ≠ 0 : c’est notre premier "pivot".
Si ce n’est pas le cas, on échange L 1 avec une ligne
dont le 1er coefficient est non nul.
• Étape 1 : Pour chaque ligne i ≥ 2, on fait
"disparaître" la première inconnue en faisant
L i ← L i − a i,1
L 1 .
a 1,1
On obtient :
blablablablablablaExempleblablablablablabla
⎧
⎪⎨ 2x + 2y + z = 1
(S) : x + 2y − z = 1
⎪⎩ 2x + y + 2z = −1
⎧
a 1,1 x 1 + a 1,2 x 2 + . . . + a 1,p x p = b 1
⎪⎨ a 2,1 x 1 + a 2,2 x 2 + . . . + a 2,p x p = b 2
.
.
.
.
⎪⎩
a n,1 x 1 + a n,2 x 2 + . . . + a n,p x p = b n
• Étape 2 : On fait la même chose avec le "soussystème"
où il n’y a plus de x 1 ...
etc jusqu’à obtenir un système triangulaire ou
échelonné.
3

Exercice 1. Résoudre les systèmes suivants :
(S 1 ) :
⎧
⎪⎨
⎪⎩
y + z = 1
x + z = 2
x + y = 3
; (S 2 ) :
⎧
⎪⎨
⎪⎩
2x + y + 2z = 0
x + y − z = 1
3x + 2y + z = 1
; (S 3 ) :
⎧
⎪⎨
⎪⎩
x + y + 3z + t = −2
x + 2y + z + 2t = −1
2x + y − z − t = 8
−x + 2y − z + 4t = −7
Exercice 2. Résoudre les systèmes suivants, selon les paramètres :
⎧
x − y + 2z + t = m
⎪⎨
−2x + 3y + z − 4t = m + 1
(S 1 ) :
, où m est un paramètre réel ;
−3x + 5y + 5z − 2t = m + 2
⎪⎩
−x + 2y − 4z − 38t = 1
⎧
⎪⎨ mx + y + z = 1
(S 2 ) : x + y + (2m − 1)z = 1 , où m est un paramètre réel ;
⎪⎩ x + my + z = 3(m + 1)
(S 3 ) :
(S 4 ) :
⎧
⎪⎨
⎪⎩
⎧
⎪⎨
⎪⎩
2x + y − z = 2
x − y + z = 4
3x + 3y − z = 4a
(2 − a)x + 2y − 2z = −2b
(1 − λ)x + y − 2z = 0
−x + (2 − λ)y − z = 0
−x + y − λz = 0
, où a et b sont des paramètres réels ;
, où λ est un paramètre réel.
4