differentiation

322 DIFFERENTIAL CALCULUS
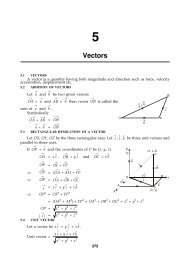
x 2 + y 2 = 25 is the equation of a circle, centre at
the origin and radius 5, as shown in Fig. 30.1. At
x = 4, the two gradients are shown.
x 2 + y 2 = 25
y
5
3
Gradient
= − 4 3
−5 0 4 5 x
Rearranging gives:
8x + 2y 3 = (10y − 6xy 2 ) dy
dx
dy
and
dx =
(b) When x = 1 and y = 2,
8x + 2y3
10y − 6xy 2 =
4x + y3
y(5 − 3xy)
dy 4(1) + (2)3
=
dx 2[5 − (3)(1)(2)] = 12
−2 = −6
−3
−5
Gradient
= 4 3
Problem 9. Find the gradients of the tangents
drawn to the circle x 2 + y 2 − 2x − 2y = 3 at
x = 2.
Figure 30.1
Above, x 2 + y 2 = 25 was differentiated implicitly;
actually, the equation could be transposed to
y = √ (25 − x 2 ) and differentiated using the function
of a function rule. This gives
dy
dx = 1 2 (25 − x2 ) −1
x
2 (−2x) =−√ (25 − x 2 )
and when x = 4,
obtained above.
dy
dx =− 4
√
(25 − 4 2 ) =±4 3
Problem 8.
(a) Find dy in terms of x and y given
dx
4x 2 + 2xy 3 − 5y 2 = 0.
(b) Evaluate dy when x = 1 and y = 2.
dx
(a) Differentiating each term in turn with respect to
x gives:
d
dx (4x2 ) + d dx (2xy3 ) − d dx (5y2 ) = d dx (0)
[ (
i.e. 8x + (2x) 3y 2 dy ) ]
+ (y 3 )(2)
dx
− 10y dy
dx = 0
i.e. 8x + 6xy 2 dy
dx + 2y3 − 10y dy
dx = 0
as
The gradient of the tangent is given by dy
dx
Differentiating each term in turn with respect to x
gives:
d
dx (x2 ) + d dx (y2 ) − d dx (2x) − d dx (2y) = d dx (3)
i.e.
2x + 2y dy
dx − 2 − 2 dy
dx = 0
Hence (2y − 2) dy
dx = 2 − 2x,
dy
from which
dx = 2 − 2x
2y − 2 = 1 − x
y − 1
The value of y when x = 2 is determined from the
original equation
Hence (2) 2 + y 2 − 2(2) − 2y = 3
i.e. 4 + y 2 − 4 − 2y = 3
or y 2 − 2y − 3 = 0
Factorising gives: (y + 1)(y − 3) = 0, from which
y =−1ory = 3
When x = 2 and y =−1,
dy
dx = 1 − x
y − 1 = 1 − 2
−1 − 1 = −1
−2 = 1 2
When x = 2 and y = 3,
dy
dx = 1 − 2
3 − 1 = −1
2
Hence the gradients of the tangents are ± 1 2