Atomic units; Molecular Hamiltonian; Born-Oppenheimer ...
Atomic units; Molecular Hamiltonian; Born-Oppenheimer ...
Atomic units; Molecular Hamiltonian; Born-Oppenheimer ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
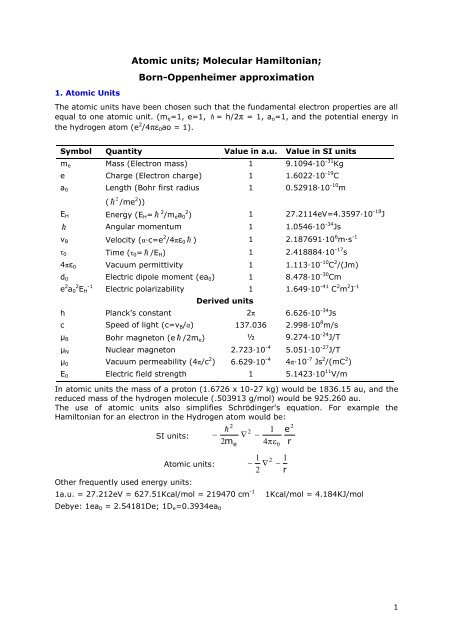
1. <strong>Atomic</strong> Units<br />
<strong>Atomic</strong> <strong>units</strong>; <strong>Molecular</strong> <strong>Hamiltonian</strong>;<br />
<strong>Born</strong>-<strong>Oppenheimer</strong> approximation<br />
The atomic <strong>units</strong> have been chosen such that the fundamental electron properties are all<br />
equal to one atomic unit. (me=1, e=1, = h/2π = 1, ao=1, and the potential energy in<br />
the hydrogen atom (e 2 /4πε0ao = 1).<br />
Symbol Quantity Value in a.u. Value in SI <strong>units</strong><br />
me Mass (Electron mass) 1 9.1094·10 -31 Kg<br />
e Charge (Electron charge) 1 1.6022·10 -19 C<br />
a0<br />
Length (Bohr first radius<br />
( 2<br />
/me 2 ))<br />
In atomic <strong>units</strong> the mass of a proton (1.6726 x 10-27 kg) would be 1836.15 au, and the<br />
reduced mass of the hydrogen molecule (.503913 g/mol) would be 925.260 au.<br />
The use of atomic <strong>units</strong> also simplifies Schrödinger's equation. For example the<br />
<strong>Hamiltonian</strong> for an electron in the Hydrogen atom would be:<br />
SI <strong>units</strong>:<br />
<strong>Atomic</strong> <strong>units</strong>:<br />
Other frequently used energy <strong>units</strong>:<br />
2<br />
2 1<br />
<br />
2 4<br />
<br />
m e<br />
0<br />
2<br />
e<br />
r<br />
1 2 1<br />
<br />
2 r<br />
1a.u. = 27.212eV = 627.51Kcal/mol = 219470 cm -1 1Kcal/mol = 4.184KJ/mol<br />
Debye: 1ea0 = 2.54181De; 1De=0.3934ea0<br />
1 0.52918·10 -10 m<br />
EH Energy (EH= 2 /mea0 2 ) 1 27.2114eV=4.3597·10 -18 J<br />
Angular momentum 1 1.0546·10 -34 Js<br />
vB Velocity (α·c=e 2 /4πε0 ) 1 2.187691·10 6 m·s -1<br />
τ0 Time (τ0= /EH) 1 2.418884·10 -17 s<br />
4πε0 Vacuum permittivity 1 1.113·10 -10 C 2 /(Jm)<br />
d0 Electric dipole moment (ea0) 1 8.478·10 -30 Cm<br />
e 2 a0 2 EH -1 Electric polarizability 1 1.649·10 -41 C 2 m 2 J -1<br />
Derived <strong>units</strong><br />
h Planck’s constant 2π 6.626·10 -34 Js<br />
c Speed of light (c=vB/α) 137.036 2.998·10 8 m/s<br />
μB Bohr magneton (e /2me) ½ 9.274·10 -24 J/T<br />
μN Nuclear magneton 2.723·10 -4<br />
5.051·10 -27 J/T<br />
μ0 Vacuum permeability (4π/c 2 ) 6.629·10 -4 4π·10 -7 Js 2 /(mC 2 )<br />
E0 Electric field strength 1 5.1423·10 11 V/m<br />
1
Energy conversion factors<br />
Hartree(a.u.) KJ/mol Kcal/mol eV cm -1<br />
Hartree(a.u.) 1 2625.5 627.51 27.212 219470<br />
KJ/mol 0.00038088 1 0.23901 0.010364 83.593<br />
Kcal/mol 0.0015936 4.184 1 0.043363 349.75<br />
eV 0.036749 96.485 23.061 1 8065.5<br />
cm-1 4.5563E-06 0.011963 0.0028591 0.00012398 1<br />
Other fundamental constants:<br />
Boltzmann’s constant: k=1.38066·10 -23 J/K<br />
Avogadro’s number: NA=6.02205·10 23 mol -1<br />
Rydberg constant: R∞=1.097373·10 7 m -1<br />
Compton wavelength of electron: λC=2.426309·10 -12 m<br />
Stefan-Boltzmann constant: σ=5.67032·10 8 W/(m 2 K 4 )<br />
Electric field unit:<br />
Field=X+a means that an electric field of a*10 -4 a.u. is applied along the X direction.<br />
1.a.u.=Hartree/(charge*bohr) = 27.2114*1.6 10 -19 J/(1.6 10 -19 C * 5.29177 10 -11 m)<br />
1 a.u. = 5.1423 10 11 V/m<br />
1 a.u. = 51.423 V/Å<br />
Ef(V/Å)=51.423Ef(a.u.)<br />
Field=X+1000 means a field of 0.1a.u. (5.1423V/Å) is applied along the X direction<br />
2. Basic concepts<br />
- structure of many-electron operators (e.g. <strong>Hamiltonian</strong>)<br />
- form of many-electron wave-functions<br />
(Slater determinants, and linear combination of them)<br />
- Hartree-Fock (HF) approximation<br />
- more sophisticated approaches which use the HF method as a starting point<br />
(correlated post-Hartree Fock methods)<br />
Aproximations made in the framework of the Hartree-Fock-Roothaan-Hall theory<br />
2
<strong>Molecular</strong> <strong>Hamiltonian</strong><br />
The non-relativistic time-independent Schrödinger equation:<br />
H|Ψ>=E|Ψ><br />
H – <strong>Hamiltonian</strong> operator for a system of nuclei and electrons<br />
N<br />
<br />
riA<br />
| riA<br />
| |<br />
ri<br />
RA<br />
|<br />
<br />
rij<br />
| rij<br />
| |<br />
ri<br />
rj<br />
|<br />
<br />
RAB<br />
|<br />
RAB<br />
| |<br />
RA<br />
RB<br />
|<br />
1 2 1 2 ZA<br />
1 ZAZB<br />
i<br />
A<br />
<br />
M <br />
<br />
A A<br />
r <br />
2 2<br />
i i<br />
A iA r<br />
i j i ij R<br />
1<br />
1<br />
1 1<br />
1 A<br />
<br />
<br />
1 B<br />
A<br />
AB<br />
T<br />
e<br />
M<br />
T<br />
N<br />
N<br />
M<br />
V<br />
eN<br />
N1<br />
V<br />
N<br />
ee<br />
M1<br />
H <br />
<br />
<br />
2<br />
i<br />
A molecular coordinate system<br />
2<br />
<br />
<br />
<br />
<br />
xi<br />
2<br />
2<br />
<br />
<br />
y<br />
2<br />
i<br />
2<br />
<br />
<br />
z<br />
2<br />
i<br />
<br />
<br />
<br />
<br />
- the major challenge in solving the time-independent Schrodinger<br />
equation<br />
MA - the ratio of the mass of nucleus A to the mass of an electron<br />
ZA – the atomic number of nucleus A<br />
Te – the operator for the kinetic energy of the electrons<br />
TN – the operator for the kinetic energy of the nuclei<br />
VeN – the operator for the Coulomb attraction between electrons and nuclei<br />
Vee – the operator for the repulsion between electrons<br />
VNN – the operator for the repulsion between nuclei<br />
(1)– represents the general problem<br />
to be separated in two parts: electronic and nuclear problems<br />
Exact solution for systems containing more than one electron is unknown!<br />
approximations, approximations<br />
i, j – electrons (N)<br />
A, B – nuclei (M)<br />
M<br />
V<br />
NN<br />
(1)<br />
3
<strong>Born</strong>-<strong>Oppenheimer</strong> Approximation<br />
Ψ=Ψ(x1,…,xN, X1,…,XM)<br />
The term VeN in the <strong>Hamiltonian</strong> prevents any wave-function Ψ(x,X) solution of the time<br />
independent Schrödinger equation from being written as a product of an electronic<br />
wavefunction and a nuclear wavefunction.<br />
Thus, we need approximations!<br />
The nuclei are much heavier than electrons (mproton=1836me)<br />
they move much more slowly<br />
the nuclei can be considered frozen in a single arrangement<br />
(molecular conformation)<br />
the electrons can respond almost instantaneously to any change<br />
in the nuclear position<br />
The electrons in a molecule are moving in the field of fixed nuclei. Neglecting the spin of<br />
nuclei, the total wavefunction is written as:<br />
On this basis:<br />
► 2-nd term in (1) can be neglected<br />
► 5-th term in (1) is a constant<br />
Electronic <strong>Hamiltonian</strong>:<br />
Ψ=Ψe(x,{R})ΨN(R)<br />
– separable form<br />
- describes the motion of N electrons in the field of M fixed point charges<br />
N<br />
i1<br />
N<br />
M<br />
i1<br />
A 1<br />
iA<br />
N1<br />
N<br />
1 2 ZA<br />
1<br />
He(<br />
R ) <br />
i<br />
<br />
2<br />
r <br />
(2)<br />
r<br />
i1<br />
ji<br />
He(R) means that He depends on the nuclei positions<br />
Electronic Schrödinger equation:<br />
ij<br />
He(R)Ψe(x;R)=Ee(R)Ψe(x;R) (3)<br />
Ψe=Ψe(x;R) (4)<br />
Ee = Ee(R) (5)<br />
(4) - is the electronic wave-function which describes the motion of the electrons<br />
- describes electronic states for fixed nuclear coordinates {R}<br />
- explicitly depends on the electronic coordinates<br />
- parametrically depends on the nuclear coordinates because H is a function of<br />
the positions R of the nuclei<br />
Another approximation: for each value of the nuclear positions, the electronic system is<br />
in the electronic ground state, corresponding to the lowest Ee(R).<br />
4
parametric dependence<br />
- the nuclear coordinates do not appear explicitly in Ψe.<br />
- from the point of view of the electrons, the nuclear degrees of freedom are fixed<br />
- a different electronic wave-function is obtained for each nuclear configuration<br />
The total energy:<br />
M 1 M<br />
pot<br />
ZAZB<br />
Etot ( R ) Ee<br />
<br />
(6)<br />
R<br />
A 1 B A<br />
Equations (2) – (6) ≡ electronic problem<br />
The electronic energy is not a constant but depends on the nuclear geometry (molecular<br />
conformation). This geometry dependent electronic energy plays the role of the<br />
potential energy in the Schrodinger equation for the nuclear motion and it is generally<br />
termed potential energy surface (PES).<br />
If the electronic problem is solved<br />
► we can solve for the motion of the nuclei using the electronic energy E(R) as<br />
the potential energy in Schrödinger equation for the nuclear motion.<br />
Since the electrons move much faster than the nuclei<br />
► we can replace the electronic coordinates by their average values (averaged<br />
over the electronic wave-function)<br />
nuclear <strong>Hamiltonian</strong><br />
o describes the motion of the nuclei in the average field of the electrons<br />
H<br />
N<br />
<br />
<br />
<br />
M<br />
N<br />
N M N1<br />
N<br />
1 2 1 2 ZA<br />
A i<br />
M <br />
2<br />
r <br />
A A<br />
2<br />
1 i1<br />
i1<br />
A 1<br />
iA i1<br />
j<br />
i<br />
M<br />
<br />
A 1<br />
M<br />
<br />
A 1<br />
1<br />
2M<br />
1<br />
2M<br />
A<br />
A<br />
<br />
<br />
2<br />
A<br />
2<br />
A<br />
E<br />
({ R})<br />
<br />
E<br />
e<br />
pot<br />
tot<br />
Potential energy surface (PES)<br />
({ R})<br />
M1<br />
M<br />
<br />
A 1 B A<br />
Z<br />
A<br />
R<br />
Z<br />
AB<br />
B<br />
pot<br />
Etot<br />
({R})<br />
AB<br />
1<br />
r<br />
ij<br />
<br />
M1<br />
M<br />
<br />
A 1 B A<br />
Schematic illustration of a potential energy surface<br />
The equilibrium conformation of the molecule corresponds to the minimum of the<br />
potential energy curve<br />
Z<br />
A<br />
R<br />
Z<br />
AB<br />
B<br />
5
nuclear Schrödinger equation<br />
ΨN - nuclear wavefunction<br />
HN|ΨN> = E|ΨN><br />
o solution of the ro-vibrational problem for the nuclear coordinates, in the<br />
presence of an electronic potential energy surface<br />
o describes the vibration, rotation and translation of a molecule<br />
E - total energy of the molecule (in the <strong>Born</strong>-<strong>Oppenheimer</strong> approximation)<br />
- includes: - electronic energy<br />
- vibrational energy<br />
- rotational energy<br />
- translational energy<br />
Total wave-function in the <strong>Born</strong>-<strong>Oppenheimer</strong> approximation:<br />
<strong>Born</strong>-<strong>Oppenheimer</strong> approximation<br />
- usually a good approximation<br />
- bad approximation for:<br />
Ψ(x,R) = Ψe(x,{R})·ΨN(R)<br />
o excited states (high energy for the nuclear motion)<br />
o degenerate or cuasidegenerate states<br />
Requirements for the wave function<br />
We will assume the <strong>Born</strong>-<strong>Oppenheimer</strong> approximation and will only be concerned with<br />
the electronic Schrödinger equation.<br />
1. Normalization<br />
For all quantum mechanical wave-function describing stationary states, is normalized<br />
to unity.<br />
<br />
( r) (<br />
r)<br />
dr<br />
1<br />
Integration is performed over the coordinates of all N electrons.<br />
The wavefunction must also be single-valued, continuous and finite.<br />
2. Antisymmetry with respect to the permutation of two electrons<br />
*<br />
Electrons are fermions and a many electron wave-function must be antisymmetric with<br />
respect to the interchange of the coordinate x (both space and spin) of any two<br />
electrons.<br />
Ψ(x1, x2, ... , xi, ..., xj, ...,xN) = -Ψ(x1, x2, ... , xj, ..., xi, ...,xN)<br />
The Pauli exclusion principle is a direct result of this antisymmetry principle.<br />
6
3. The electronic wavefunctions must be eigenfunctions of Sz and S 2 operators,<br />
with the eigenvalues MS and S(S+1).<br />
Since the electronic <strong>Hamiltonian</strong> does not contain any spin operators, it does commute<br />
with the operators Sz and S 2 defined below, with the corresponding eigenvalues MS and<br />
S(S+1), respectively.<br />
S<br />
N<br />
2<br />
sz<br />
MS<br />
S s<br />
z i<br />
i<br />
N<br />
i<br />
2<br />
i<br />
[H,Sz]=0 [H,S 2 ]=0<br />
<br />
S(<br />
S<br />
1)<br />
We take care of spin by using spin-orbitals instead of pure spatial orbitals.<br />
α(σ) and β(σ) – spin functions (complete and orthonormal)<br />
<br />
<br />
(<br />
) (<br />
) d<br />
<br />
<br />
(<br />
) ( ) d<br />
<br />
<br />
<br />
and<br />
<br />
( ) ( ) d<br />
1<br />
1<br />
( ) (<br />
) d<br />
0<br />
0<br />
The electron is described by spatial (r) and spin (σ) coordinates:<br />
Homework<br />
x={r,σ}<br />
1. Write the Z-matrix for the tetramethylsilane<br />
(TMS=Si(CH3)4) molecule in Td symmetry.<br />
2. Use the Gaussian program and obtain the PES of the water molecule in the C2v<br />
symmetry by varying the bond length from 0.9 to 1.1 and the bond angle from 100 o to<br />
110 o . Plot the PES using a dedicated software (Mathematica, MathLab, Mathcad).<br />
3. Write a C program to calculate the<br />
coordinates of the atoms in a graphene sheet<br />
(10x10 atoms) whose structure is given<br />
below. The C-C bond length in graphene is<br />
1.42Å.<br />
The Nobel prize in physics for 2010 was awarded to Andre<br />
Geim and Konstantin Novoselov at the University of Manchester "for groundbreaking experiments regarding the<br />
two-dimensional material graphene".<br />
7