Proofs of Divergence of the Harmonic Series - Prairie State College
Proofs of Divergence of the Harmonic Series - Prairie State College
Proofs of Divergence of the Harmonic Series - Prairie State College
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
More <strong>Pro<strong>of</strong>s</strong> <strong>of</strong> <strong>Divergence</strong> <strong>of</strong> <strong>the</strong> <strong>Harmonic</strong> <strong>Series</strong><br />
Steven J. Kifowit<br />
<strong>Prairie</strong> <strong>State</strong> <strong>College</strong><br />
In an earlier article, Kifowit and Stamps [13] summarized a number <strong>of</strong> elementary pro<strong>of</strong>s <strong>of</strong><br />
divergence <strong>of</strong> <strong>the</strong> harmonic series:<br />
∞<br />
n=1<br />
1 1 1 1 1<br />
= 1+ + + + +··· = ∞.<br />
n 2 3 4 5<br />
For a variety <strong>of</strong> reasons, some very nice pro<strong>of</strong>s never made it into <strong>the</strong> final draft <strong>of</strong> that article.<br />
With this in mind, <strong>the</strong> collection <strong>of</strong> divergence pro<strong>of</strong>s continues here. This informal note is a<br />
work in progress 1 . On occasion more pro<strong>of</strong>s will be added. Accessibility to first-year calculus<br />
students is a common thread that will continue (usually) to connect <strong>the</strong> pro<strong>of</strong>s.<br />
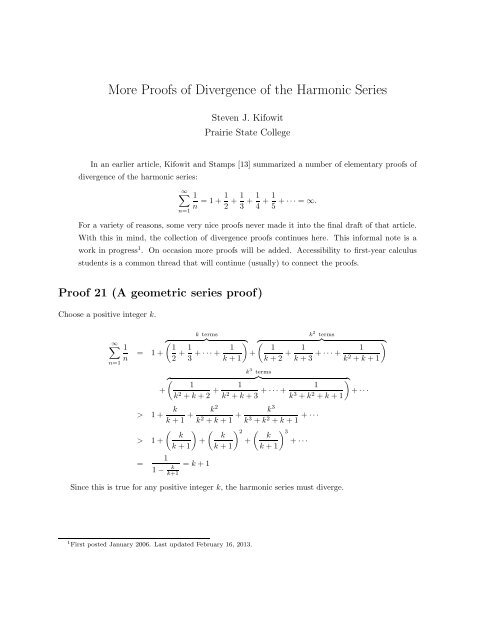
Pro<strong>of</strong> 21 (A geometric series pro<strong>of</strong>)<br />
Choose a positive integer k.<br />
∞<br />
n=1<br />
1<br />
n<br />
= 1+<br />
k terms<br />
<br />
<br />
1 1 1<br />
+ +···+<br />
2 3 k +1<br />
<br />
+<br />
k 2 terms<br />
<br />
1 1<br />
+<br />
k +2 k +3 +···+<br />
1<br />
k2 <br />
+k +1<br />
k 3 terms<br />
<br />
1<br />
+<br />
k2 +k +2 +<br />
1<br />
k2 +k +3 +···+<br />
1<br />
k3 +k2 <br />
+···<br />
+k +1<br />
k 2<br />
> 1+ k<br />
k +1 +<br />
k2 +k +1 +<br />
k3 +k2 +k +1 +···<br />
2 3 k k k<br />
> 1+ + + +···<br />
k +1 k +1 k +1<br />
=<br />
1<br />
1− k<br />
k+1<br />
= k +1<br />
Since this is true for any positive integer k, <strong>the</strong> harmonic series must diverge.<br />
1 First posted January 2006. Last updated February 16, 2013.<br />
k 3
Pro<strong>of</strong> 22<br />
The following pro<strong>of</strong> was given by Fearnehough [8] and later by Havil [9]. After substituting u = e x , this<br />
pro<strong>of</strong> is equivalent to Pro<strong>of</strong> 10 <strong>of</strong> [13].<br />
0<br />
ex dx =<br />
1−e x<br />
−∞<br />
=<br />
=<br />
=<br />
0<br />
−∞<br />
0<br />
−∞<br />
0<br />
e x (1−e x ) −1 dx<br />
e x (1+e x +e 2x +e 3x +···)dx<br />
(e<br />
−∞<br />
x +e 2x +e 3x +···)dx<br />
<br />
e x + 1<br />
2 e2x + 1<br />
3 e3x 0 +···<br />
= 1+ 1 1<br />
+<br />
2 3 +···<br />
= [−ln(1−e x )] 0<br />
−∞ = ∞<br />
Pro<strong>of</strong> 23 (A telescoping series pro<strong>of</strong>)<br />
This pro<strong>of</strong> was given by Bradley [2]. We begin with <strong>the</strong> inequality x ≥ ln(1+x), which holds for all x > −1.<br />
From this it follows that<br />
for any positive integer k. Now we have<br />
Hn =<br />
≥<br />
k=1<br />
k=1<br />
<br />
1<br />
≥ ln 1+<br />
k 1<br />
<br />
= ln(k +1)−ln(k)<br />
k<br />
n 1<br />
k<br />
n<br />
<br />
ln 1+ 1<br />
<br />
k<br />
=<br />
n<br />
<br />
k +1<br />
ln<br />
k<br />
k=1<br />
= [ln(n+1)−ln(n)]+[ln(n)−ln(n−1)]+···+[(ln(2)−ln(1)]<br />
= ln(n+1).<br />
Therefore {Hn} is unbounded, and <strong>the</strong> harmonic series diverges.<br />
Pro<strong>of</strong> 24 (A limit comparison pro<strong>of</strong>)<br />
In <strong>the</strong> last pro<strong>of</strong> <strong>the</strong> harmonic series was directly compared to <strong>the</strong> divergent series<br />
<strong>of</strong> <strong>the</strong> inequality x ≥ ln(1+x) can be avoided by using limit comparison. Since<br />
ln<br />
lim<br />
x→∞<br />
1+ 1<br />
<br />
x −<br />
= lim<br />
x→∞<br />
1<br />
x2 <br />
1 = 1,<br />
−<br />
<strong>the</strong> harmonic series diverges by limit comparison.<br />
1<br />
x<br />
2<br />
1+ 1<br />
x<br />
x 2<br />
−∞<br />
∞<br />
k=1<br />
<br />
ln 1+ 1<br />
<br />
. The use<br />
k
Pro<strong>of</strong> 25<br />
In an interesting pro<strong>of</strong> <strong>of</strong> <strong>the</strong> Egyptian fraction <strong>the</strong>orem, Owings [18] showed that no number appears more<br />
than once in any single row <strong>of</strong> <strong>the</strong> following tree.<br />
1/5<br />
.<br />
1/4<br />
1/20<br />
.<br />
1/3<br />
1/13<br />
.<br />
1/12<br />
1/156<br />
.<br />
1/2<br />
1/8<br />
.<br />
1/7<br />
1/56<br />
.<br />
1/6<br />
1/43<br />
.<br />
1/42<br />
1/1806<br />
The elements <strong>of</strong> each row have a sum <strong>of</strong> 1/2, and <strong>the</strong>re are infinitely rows with no elements in common. (For<br />
example, one could, starting with row 1, find <strong>the</strong> maximum denominator in <strong>the</strong> row, and <strong>the</strong>n jump to that<br />
row.) It follows that <strong>the</strong> harmonic series diverges.<br />
Pro<strong>of</strong> 26<br />
This pro<strong>of</strong> is actually a pair <strong>of</strong> very similar pro<strong>of</strong>s. They are closely related to a number <strong>of</strong> o<strong>the</strong>r pro<strong>of</strong>s, but<br />
most notably to Pro<strong>of</strong> 4 <strong>of</strong> [13]. In <strong>the</strong>se pro<strong>of</strong>s Hn denotes <strong>the</strong> nth partial sum <strong>of</strong> <strong>the</strong> harmonic series:<br />
Pro<strong>of</strong> (A): First notice that<br />
Hn = 1+ 1 1<br />
+<br />
2 3<br />
so that when all is said and done, we have<br />
1<br />
+···+ , n = 1,2,3,....<br />
n<br />
Hn +H2n = 2Hn + 1 1 1<br />
+ +···+<br />
n+1 n+2 2n ≥ 2Hn + n<br />
2n ,<br />
Hn +H2n ≥ 2Hn + 1<br />
2 .<br />
Now suppose <strong>the</strong> harmonic series converges with sum S.<br />
The contradiction 2S ≥ 2S + 1<br />
2<br />
2S = lim<br />
n→∞ Hn + lim<br />
n→∞ H2n<br />
= lim<br />
n→∞ (Hn +H2n)<br />
≥ lim<br />
n→∞<br />
<br />
= 2S + 1<br />
2<br />
concludes <strong>the</strong> pro<strong>of</strong>.<br />
Pro<strong>of</strong> (B): This pro<strong>of</strong> was given by Ward [20].<br />
2Hn + 1<br />
<br />
2<br />
H2n −Hn = 1 1 1 n 1<br />
+ +···+ ≥ =<br />
n+1 n+2 2n 2n 2<br />
3<br />
.
Suppose <strong>the</strong> harmonic series converges.<br />
The contradiction 0 ≥ 1<br />
2<br />
Pro<strong>of</strong> 27<br />
concludes <strong>the</strong> pro<strong>of</strong>.<br />
0 = lim<br />
n→∞ H2n − lim<br />
n→∞ Hn<br />
= lim<br />
n→∞ (H2n −Hn)<br />
1 1<br />
≥ lim =<br />
n→∞ 2 2<br />
This proposition follows immediately from <strong>the</strong> harmonic mean/arithmetic mean inequality, but an alternate<br />
pro<strong>of</strong> is given here.<br />
Proposition: For any natural number k,<br />
Pro<strong>of</strong>:<br />
exp<br />
<br />
1 1 1 1<br />
+ + +···+<br />
k k +1 k +2 3k<br />
1 1 1<br />
+ +···+<br />
k k +1 3k<br />
> 1.<br />
= e 1/k ·e 1/(k+1) ·e 1/(k+2) ···e 1/(3k)<br />
><br />
=<br />
<br />
1+ 1<br />
<br />
· 1+<br />
k<br />
1<br />
<br />
· 1+<br />
k +1<br />
1<br />
<br />
··· 1+<br />
k +2<br />
1<br />
<br />
3k<br />
<br />
k +1 k +2 k +3 3k +1<br />
· · ···<br />
k k +1 k +2 3k<br />
= 3k +1<br />
> 3.<br />
k<br />
(In this proposition <strong>the</strong> denominator 3k could be replaced by ⌊ek⌋, but even this choice is<br />
not optimal. See [1] and [11].)<br />
Based on this proposition, we have <strong>the</strong> following result:<br />
∞<br />
<br />
1 1 1 1 1 1 1<br />
= 1+ +···+ + +···+ + +···+ +···<br />
n 2 6 7 21 22 66<br />
n=1<br />
> 1+1+1+1+···<br />
Pro<strong>of</strong> 28 (A Fibonacci number pro<strong>of</strong>)<br />
The following pro<strong>of</strong> was given by Kifowit and Stamps [12] and by Chen and Kennedy [3].<br />
The Fibonacci numbers are defined recursively as follows:<br />
f0 = 1, f1 = 1; fn+1 = fn +fn−1, n = 1,2,3....<br />
For example, <strong>the</strong> first ten are given by 1, 1, 2, 3, 5, 8, 13, 21, 34, 55. The sequence <strong>of</strong> Fibonacci numbers<br />
makes an appearance in a number <strong>of</strong> modern calculus textbooks (for instance, see [14] or [19]). Often <strong>the</strong><br />
limit<br />
fn+1<br />
lim = φ =<br />
n→∞ fn<br />
1+√5 2<br />
4
is proved or presented as an exercise. This limit plays an important role in this divergence pro<strong>of</strong>.<br />
First notice that<br />
<br />
fn−1 fn+1 −fn<br />
lim = lim = lim 1−<br />
n→∞ fn+1 n→∞ fn+1 n→∞<br />
fn<br />
<br />
= 1−<br />
fn+1<br />
1<br />
≈ 0.381966.<br />
φ<br />
Now we have<br />
∞<br />
n=1<br />
1<br />
n<br />
1 1 1 1 1<br />
= 1+ + + + +<br />
2 3 4 5 6 +···<br />
= 1+ 1 1<br />
+<br />
2 3 +<br />
<br />
1 1 1 1 1<br />
+ + + +<br />
4 5 6 7 8<br />
<br />
1 1 1 1<br />
+ +···+ + +···+ +···<br />
9 13 14 21<br />
≥ 1+ 1 1<br />
+<br />
2 3<br />
∞<br />
= 1+<br />
fn−1<br />
fn+1<br />
n=1<br />
2 3 5 8<br />
+ + + +<br />
5 8 13 21 +···<br />
fn−1<br />
Since lim = 0, this last series diverges. It follows that <strong>the</strong> harmonic series diverges.<br />
n→∞ fn+1<br />
Pro<strong>of</strong> 29<br />
This pro<strong>of</strong> is essentially <strong>the</strong> same as Pro<strong>of</strong> 2 <strong>of</strong> [13]. First notice that since <strong>the</strong> sequence<br />
11<br />
10<br />
increases and converges to 10/9, <strong>the</strong> sequence<br />
10<br />
11<br />
, 111<br />
100<br />
, 100<br />
111<br />
, 1111<br />
1000<br />
, 1000<br />
1111<br />
decreases and converges to 9/10. With this in mind, we have<br />
∞<br />
n=1<br />
1<br />
n<br />
= 1+<br />
+<br />
10 terms<br />
<br />
<br />
1 1 1<br />
+ +···+<br />
2 3 11<br />
1000 terms<br />
11111<br />
, , ...<br />
10000<br />
10000<br />
, , ...<br />
11111<br />
<br />
+<br />
<br />
1 1 1<br />
+ +···+ +···<br />
112 113 1111<br />
> 1+ 10 100 1000<br />
+ +<br />
11 1111 1111 +···<br />
> 1+ 9 9 9<br />
+ +<br />
10 10 10 +···<br />
5<br />
100 terms<br />
<br />
1 1 1<br />
+ +···+<br />
12 13 111
Pro<strong>of</strong> 30<br />
The following visual pro<strong>of</strong>s show that by carefully rearranging terms, <strong>the</strong> harmonic series can be made<br />
greater than itself.<br />
Pro<strong>of</strong> (A): This pro<strong>of</strong> without words was posted on The Everything Seminar (<strong>Harmonic</strong> Digression,<br />
http://cornellmath.wordpress.com/2007/07/12/harmonic-digression/).<br />
: :<br />
. ..<br />
Figure 1: Pro<strong>of</strong> 30(A)<br />
Pro<strong>of</strong> (B): This visual pro<strong>of</strong> leaves less to <strong>the</strong> imagination than Pro<strong>of</strong> (A). It is due to Jim Belk and<br />
was posted on The Everything Seminar as a follow-up to <strong>the</strong> previous pro<strong>of</strong>. Belk’s pro<strong>of</strong> is a visualization<br />
<strong>of</strong> Johann Bernoulli’s pro<strong>of</strong> (see Pro<strong>of</strong> 13 <strong>of</strong> [13]).<br />
: :<br />
.. .<br />
Figure 2: Pro<strong>of</strong> 30(B)<br />
Pro<strong>of</strong>(C): Thispro<strong>of</strong>isavisualrepresentation<strong>of</strong><strong>Pro<strong>of</strong>s</strong>6and7<strong>of</strong>[13]. Withsomeminormodifications,<br />
6<br />
· · ·<br />
· · ·
a similar visual pro<strong>of</strong> could be used to show that one-half <strong>of</strong> <strong>the</strong> harmonic series ( 1 1 1<br />
2 + 4 + 6 +···) is strictly<br />
less than its remaining half (in <strong>the</strong> spirit <strong>of</strong> Pro<strong>of</strong> 8 <strong>of</strong> [13]).<br />
Pro<strong>of</strong> 31<br />
1<br />
1<br />
1/2<br />
1/3<br />
1/2<br />
1/4 1/5 1/6 1/7 1/8 · · ·<br />
Figure 3: Pro<strong>of</strong> 30(C)<br />
1/3 1/4 · · ·<br />
Here is ano<strong>the</strong>r pro<strong>of</strong> in which 1/k and 1/xdx are compared. Unlike its related pro<strong>of</strong>s (e.g. Pro<strong>of</strong> 9 <strong>of</strong><br />
[13]), this one focuses on arc length.<br />
The graph shown here is that <strong>of</strong> <strong>the</strong> polar function r(θ) = π/θ on [π,∞).<br />
The total arc length is unbounded:<br />
<br />
∞<br />
Arc Length = r2 2 dr<br />
+ dθ ≥<br />
dθ<br />
π<br />
∞<br />
π<br />
y<br />
r(θ) = π<br />
θ<br />
√ r 2 dθ =<br />
7<br />
∞<br />
π<br />
x<br />
rdθ =<br />
∞<br />
π<br />
π<br />
θ<br />
<br />
<br />
dθ = π lnθ<br />
∞<br />
π<br />
= ∞
Now let <strong>the</strong> polar function ρ be defined by<br />
⎧<br />
1,<br />
1/2,<br />
⎪⎨ 1/3,<br />
π ≤ θ < 2π<br />
2π ≤ θ < 3π<br />
3π ≤ θ < 4π<br />
ρ(θ) =<br />
.<br />
.<br />
⎪⎩<br />
1/n, nπ ≤ θ < (n+1)π<br />
.<br />
The graph <strong>of</strong> ρ is made up <strong>of</strong> semi-circular arcs, <strong>the</strong> nth arc having radius 1/n. The total arc length <strong>of</strong><br />
<strong>the</strong> graph <strong>of</strong> ρ is<br />
π + π<br />
<br />
π π<br />
+ + +··· = π 1+<br />
2 3 4 1 1 1<br />
+ +<br />
2 3 4 +···<br />
<br />
.<br />
The graphs <strong>of</strong> r (dashed) and ρ (solid) on [π,6π] are shown below.<br />
y<br />
By comparing <strong>the</strong> graphs <strong>of</strong> <strong>the</strong> two functions over intervals <strong>of</strong> <strong>the</strong> form [nπ,(n+1)π], we see that <strong>the</strong><br />
graph <strong>of</strong> ρ must be “longer” than <strong>the</strong> graph <strong>of</strong> r. It follows that<br />
<br />
π 1+ 1 1 1<br />
+ +<br />
2 3 4 +···<br />
<br />
must be unbounded.<br />
Pro<strong>of</strong> 32<br />
The divergence <strong>of</strong> <strong>the</strong> harmonic series follows immediately from <strong>the</strong> Cauchy Condensation Test:<br />
∞<br />
Suppose {an} is a non-increasing sequence with positive terms. Then an converges if and<br />
only if<br />
∞<br />
2 k a2k converges.<br />
k=0<br />
8<br />
.<br />
x<br />
n=1
Pro<strong>of</strong> 33<br />
This pro<strong>of</strong> is similar to Pro<strong>of</strong> 4 <strong>of</strong> [13]. Just as above, Hn denotes <strong>the</strong> nth partial sum <strong>of</strong> <strong>the</strong> harmonic series:<br />
or<br />
Consider <strong>the</strong> figure shown here:<br />
1<br />
n<br />
1<br />
n+1<br />
Referring to <strong>the</strong> figure, we see that<br />
Repeated use <strong>of</strong> this result gives<br />
1 1<br />
+···+<br />
<br />
n+1<br />
<br />
2n<br />
<br />
H2n−Hn<br />
Hn = 1+ 1 1<br />
+<br />
2 3<br />
y = 1<br />
x<br />
1<br />
n+1 <<br />
<<br />
1<br />
+···+ , n = 1,2,3,....<br />
n<br />
n n+1<br />
2n<br />
n<br />
n+1<br />
n<br />
1 1<br />
dx <<br />
x n .<br />
1 1 1<br />
dx = ln2 < +···+<br />
x<br />
<br />
n<br />
<br />
2n−1<br />
<br />
H2n −Hn < ln2 < H2n −Hn + 1 1<br />
− .<br />
<br />
n<br />
<br />
2n<br />
<br />
1/2n<br />
From this it follows that<br />
ln2− 1<br />
2n < H2n −Hn < ln2.<br />
Therefore H2n −Hn → ln2, and <strong>the</strong> sequence {Hn} must diverge.<br />
Pro<strong>of</strong> 34<br />
H2n−1−Hn−1<br />
In [7] Paul Erdős gave two remarkably clever pro<strong>of</strong>s <strong>of</strong> <strong>the</strong> divergence <strong>of</strong> <strong>the</strong> series 1/p (p prime), where<br />
<strong>the</strong> sum is taken over only <strong>the</strong> primes. O<strong>the</strong>r pro<strong>of</strong>s <strong>of</strong> this fact, such as one given by Euler (see [6]), make<br />
use <strong>of</strong> <strong>the</strong> harmonic series. Erdős’ pro<strong>of</strong>s do not, and as a consequence, <strong>the</strong>y establish <strong>the</strong> divergence <strong>of</strong> <strong>the</strong><br />
harmonic series.<br />
9
The pro<strong>of</strong>s are ra<strong>the</strong>r complicated, but accessible and well worth <strong>the</strong> effort required to follow <strong>the</strong>m<br />
through. A few elementary ideas are required before we begin:<br />
(i) ⌊x⌋ denotes <strong>the</strong> greatest integer less than or equal to x.<br />
(ii) We denote <strong>the</strong> primes, in ascending order, by p1,p2,p3,p4,....<br />
(iii) Let N be a given positive integer. For any positive integer m, <strong>the</strong>re are ⌊N/m⌋ integers between 1 and<br />
N that are divisible by m.<br />
This is <strong>the</strong> first <strong>of</strong> Erdős’ pro<strong>of</strong>s. We begin by assuming<br />
integer K such that<br />
∞<br />
1<br />
pi<br />
i=K+1<br />
< 1<br />
2 .<br />
∞<br />
1<br />
pi<br />
i=1<br />
converges. It follows that <strong>the</strong>re exists an<br />
We will call pK+1,pK+2,pK+3,... <strong>the</strong> “large primes,” and p1,p2,...,pK <strong>the</strong> “small primes.”<br />
Now let N be an integer such that N > pK. Let N1 be <strong>the</strong> number <strong>of</strong> integers between 1 and N whose<br />
divisors are all small primes, and let N2 be <strong>the</strong> number <strong>of</strong> integers between 1 and N that have at least one<br />
large prime divisor. It follows that N = N1 +N2.<br />
By <strong>the</strong> definition <strong>of</strong> N2 and using (iii) above, it follows that<br />
From this we get<br />
N2 ≤<br />
N<br />
pK+1<br />
<br />
N N<br />
+ + +··· =<br />
pK+2 pK+3<br />
N2 ≤<br />
∞<br />
i=K+1<br />
<br />
N<br />
≤<br />
pi<br />
∞<br />
N<br />
pi<br />
i=K+1<br />
where <strong>the</strong> last part <strong>of</strong> <strong>the</strong> inequality follows from <strong>the</strong> definition <strong>of</strong> K.<br />
< N<br />
2 ,<br />
∞<br />
i=K+1<br />
Now, referring back to <strong>the</strong> small primes, let x ≤ N be a positive integer with only small prime divisors.<br />
Write x = yz 2 , where y and z are positive integers, y is squarefree (i.e. has no perfect square divisors), and<br />
z ≤ √ N. The integer y must have <strong>the</strong> factorization<br />
y = p m1<br />
1 pm2<br />
2 pm3<br />
3 ···p mK<br />
K ,<br />
where each exponent mi has value 0 or 1. It follows from <strong>the</strong> multiplication principle that <strong>the</strong>re are 2 K<br />
possible choices for <strong>the</strong> integer y. Since z ≤ √ N, <strong>the</strong>re are at most √ N possible choices for <strong>the</strong> integer z.<br />
Therefore <strong>the</strong>re are at most 2 K√ N possible choices for <strong>the</strong> integer x. Recalling <strong>the</strong> definition <strong>of</strong> x, we see<br />
that we must have N1 ≤ 2 K√ N.<br />
So now we have established that<br />
N = N1 +N2 < 2 K√ N + N<br />
2 .<br />
However, by simply choosing N such that N > 22K+2 , we are lead to a contradiction:<br />
N = N1 +N2 < 2 K√ N + N<br />
2 <<br />
√<br />
N√<br />
N<br />
N + = N.<br />
2 2<br />
10<br />
N<br />
pi<br />
<br />
.
Pro<strong>of</strong> 35<br />
This is Erdős’ second pro<strong>of</strong> <strong>of</strong> <strong>the</strong> divergence <strong>of</strong> 1/p (p prime) [7]. It uses <strong>the</strong> same notation and concepts<br />
as <strong>the</strong> previous pro<strong>of</strong>.<br />
We begin by using <strong>the</strong> fact that<br />
to establish that<br />
Now assume that<br />
∞<br />
1<br />
pi<br />
i=1<br />
∞<br />
1<br />
p<br />
i=1<br />
2 i<br />
∞<br />
i=2<br />
1<br />
i(i+1) =<br />
∞<br />
i=2<br />
<br />
1 1<br />
− =<br />
i i+1<br />
1<br />
2<br />
< 1 1 1 1 1 1 3<br />
+ + + +··· = + =<br />
4 2·3 3·4 4·5 4 2 4 .<br />
converges. It follows that <strong>the</strong>re exists an integer K such that<br />
∞<br />
1<br />
pi<br />
i=K+1<br />
< 1<br />
8 .<br />
As above, we will call pK+1,pK+2,pK+3,... <strong>the</strong> “large primes,” and p1,p2,...,pK <strong>the</strong> “small primes.”<br />
Let N be a positive integer and let y ≤ N be a positive, squarefree integer with only small prime divisors.<br />
The integer y must have <strong>the</strong> factorization<br />
y = p m1<br />
1 pm2<br />
2 pm3<br />
3 ···p mK<br />
K ,<br />
where each exponent mi has value 0 or 1. It follows from <strong>the</strong> multiplication principle that <strong>the</strong>re are 2 K possi-<br />
ble choices for <strong>the</strong> integer y. Those 2 k integers must remain after we remove from <strong>the</strong> sequence 1,2,3,...,N<br />
all those integers that are not squarefree or have large prime divisors. Therefore, we must have <strong>the</strong> following<br />
inequality:<br />
2 K ≥ N −<br />
K<br />
<br />
N<br />
i=1<br />
p 2 i<br />
<br />
−<br />
∞<br />
K N N<br />
≥ N − −<br />
i=K+1<br />
pi<br />
p<br />
i=1<br />
2 i<br />
However, if we simply choose N ≥ 2 K+3 , we have a contradiction.<br />
11<br />
∞<br />
N<br />
pi<br />
i=K+1<br />
> N − 3 1 N<br />
N − N =<br />
4 8 8 .
Pro<strong>of</strong> 36<br />
Nick Lord [15] provided this “visual catalyst” for Pro<strong>of</strong> 23.<br />
Consider <strong>the</strong> graph <strong>of</strong> y = sin(e x ) for 0 ≤ x < ∞.<br />
y<br />
y = sin(e x )<br />
The graph has an x-intercept at <strong>the</strong> point where x = lnnπ for each positive integer n. This sequence <strong>of</strong><br />
x-values diverges to infinity, and <strong>the</strong> distance between each pair <strong>of</strong> intercepts is given by<br />
<br />
n+1<br />
ln(n+1)π−lnnπ = ln = ln 1+<br />
n<br />
1<br />
<br />
.<br />
n<br />
Since ln(1+ 1 1<br />
n ) < n for each n, <strong>the</strong> series <strong>of</strong> gaps between intercepts has a total length less than <strong>the</strong> harmonic<br />
series:<br />
∞<br />
n=1<br />
<br />
ln 1+ 1<br />
<br />
<<br />
n<br />
Since <strong>the</strong> sequence <strong>of</strong> x-intercepts diverges, <strong>the</strong> harmonic series must diverge.<br />
12<br />
∞<br />
n=1<br />
1<br />
n .<br />
x
Pro<strong>of</strong> 37<br />
This is ano<strong>the</strong>r visual pro<strong>of</strong> comparing <strong>the</strong> harmonic series to a divergent integral. It is similar to Pro<strong>of</strong> 9<br />
<strong>of</strong> [13].<br />
Pro<strong>of</strong> 38<br />
7<br />
6<br />
5<br />
4<br />
3<br />
2<br />
1<br />
y<br />
.<br />
1/7<br />
1/6<br />
1/5<br />
1/4<br />
1/3<br />
1/2<br />
1<br />
1/2<br />
y =<br />
1 − x<br />
x<br />
1+ 1<br />
1<br />
1 1 1<br />
+ + + +··· ><br />
2 3 4 5 0<br />
1<br />
1−x<br />
x<br />
dx = ∞<br />
Here is a matrix version <strong>of</strong> Johann Bernoulli’s pro<strong>of</strong> (Pro<strong>of</strong> 13 <strong>of</strong> [13]). We start by defining <strong>the</strong> infinite<br />
matrices M (square) and h:<br />
⎡<br />
⎤ ⎡ ⎤<br />
0 2 0 0 0 0 ··· 1<br />
⎢<br />
⎥ ⎢ ⎥<br />
⎢ 0 0 3 0 0 0 ··· ⎥ ⎢ 1/2 ⎥<br />
⎢<br />
⎥ ⎢ ⎥<br />
⎢<br />
M = ⎢ 0 0 0 4 0 0 ···<br />
⎥ ⎢<br />
⎥,<br />
h = ⎢ 1/3<br />
⎥<br />
⎢<br />
⎥ ⎢ ⎥<br />
⎢<br />
⎣<br />
0 0 0 0 5 0 ··· ⎥ ⎢<br />
⎦ ⎣<br />
1/4 ⎥<br />
⎦<br />
.<br />
. . . . . . .. .<br />
Notice that hTMh = ∞ n=1 1 1 1 1<br />
n = 1+ 2 + 3 + 4 +···. Therefore our goal is to show that hTMh = ∞.<br />
Let Jk be <strong>the</strong> infinite square matrix with ones along <strong>the</strong> superdiagonal starting at row k and with zeros<br />
13<br />
x
elsewhere. For example,<br />
⎡<br />
⎤<br />
0 0 0 0 0 0 ···<br />
⎢<br />
⎥<br />
⎢<br />
0 0 0 0 0 0 ··· ⎥<br />
⎢<br />
⎥<br />
⎢ 0 0 0 1 0 0 ··· ⎥<br />
J3 = ⎢<br />
⎥<br />
⎢ 0 0 0 0 1 0 ··· ⎥.<br />
⎥<br />
⎢<br />
⎥<br />
⎢ 0 0 0 0 0 1 ··· ⎥<br />
⎣<br />
⎦<br />
.<br />
. . . . . . ..<br />
It follows that M = J1+J1+J2+J3+J4+··· (in <strong>the</strong> sense that, for any n, <strong>the</strong> equality holds for <strong>the</strong> n×n<br />
leading, principal submatrices).<br />
By expanding h T J1h, we find that<br />
h T J1h =<br />
∞<br />
n=1<br />
From here it is easy to show (perhaps by induction) that<br />
1<br />
= 1.<br />
n(n+1)<br />
h T Jkh = 1<br />
k .<br />
Now suppose that <strong>the</strong> harmonic series converges with sum S. Then we have<br />
S = h T Mh = h T (J1 +J1 +J2 +J3 +J4 +···)h<br />
= h T J1h+h T J1h+h T J2h+h T J3h+h T J4h+···<br />
= 1+1+ 1 1 1<br />
2 + 3 + 4 +···<br />
= 1+S.<br />
The conclusion, S = 1+S, is impossible unless S = ∞.<br />
Pro<strong>of</strong> 39 (Cesàro summability)<br />
It is not difficult to prove <strong>the</strong> following result (see for example [10, pages 128–129]), which was studied in<br />
detail by <strong>the</strong> Italian ma<strong>the</strong>matician Ernesto Cesàro.<br />
Suppose that ∞ k=1ak converges with sum S and let Sn = n k=1ak. Then <strong>the</strong> sequence <strong>of</strong><br />
average partial sums, { 1 n n k=1Sk} ∞ n=1, also converges to S.<br />
In order to use this result, let<br />
and notice that<br />
1<br />
n<br />
k=1<br />
Hn = 1+ 1 1 1 1<br />
+ + +···+<br />
2 3 4 n ,<br />
n<br />
Hk = 1<br />
<br />
n n−1<br />
+<br />
n 1 2<br />
= 1<br />
n<br />
n<br />
k=1<br />
n−k +1<br />
k<br />
(A Pro<strong>of</strong> Without Words for this last fact is given in [17].)<br />
14<br />
+ n−2<br />
3<br />
<br />
1<br />
+···+<br />
n<br />
= Hn −1+ Hn<br />
n<br />
.
The divergence <strong>of</strong> <strong>the</strong> harmonic series now follows by contradiction. Assuming that <strong>the</strong> harmonic series<br />
converges with sum S, we have<br />
<br />
1<br />
S = lim<br />
n→∞ n<br />
Pro<strong>of</strong> 40<br />
n<br />
k=1<br />
Hk<br />
<br />
<br />
= lim Hn −1+<br />
n→∞<br />
Hn<br />
<br />
= S −1+0.<br />
n<br />
The following pro<strong>of</strong> was given by Augustus De Morgan [5]. Similar to several <strong>of</strong> <strong>the</strong> previous pro<strong>of</strong>s, it is<br />
included here for its historical significance. De Morgan claims to have been shown this pro<strong>of</strong> many years<br />
prior to its publication by his young student J. J. Sylvester, who himself went on to become a famous<br />
ma<strong>the</strong>matician. De Morgan’s presentation is duplicated verbatim:<br />
“It is well known that when a−b+c−d+... consists <strong>of</strong> terms diminishing without limit, <strong>the</strong> series is<br />
convergent, with a limit between a and a−b. Now<br />
1 + 1<br />
2<br />
+ 1<br />
3<br />
+ 1<br />
4<br />
+ ... is 1 − 1<br />
2<br />
+ 1<br />
3<br />
− 1<br />
4<br />
+ 1 + 1<br />
2<br />
And if it be S, we have S = a+S, where a is finite. Hence S is infinite.”<br />
Pro<strong>of</strong> 41<br />
This pro<strong>of</strong> was given by Cusumano [4]. Suppose m is an integer greater than 1.<br />
∞<br />
n=1<br />
+ ...<br />
+ ...<br />
1<br />
n =<br />
<br />
1 1 1 1<br />
+···+ + +···+<br />
1 m m+1 m2 <br />
<br />
1<br />
+<br />
m2 1<br />
+···+<br />
+1 m3 <br />
1<br />
+<br />
m3 1<br />
+···+<br />
+1 m4 <br />
+···<br />
> m<br />
m + m2 −m<br />
m 2 + m3 −m 2<br />
m 3 + m4 −m 3<br />
m 4 +···<br />
= 1+ m(m−1)<br />
mm + m2 (m−1)<br />
m 2 m + m3 (m−1)<br />
m 3 m +···<br />
= 1+ m−1<br />
m<br />
= ∞<br />
+ m−1<br />
m<br />
+ m−1<br />
m +···<br />
Notice that if <strong>the</strong> first term on <strong>the</strong> right is rewritten,<br />
<br />
1 1 1 1<br />
+···+ = 1+ +···+ ,<br />
1 m 2 m<br />
Cusumano’s pro<strong>of</strong> becomes precisely <strong>the</strong> generalization <strong>of</strong> Oresme’s classical pro<strong>of</strong> that was described after<br />
Pro<strong>of</strong> 1 <strong>of</strong> [13].<br />
15
Pro<strong>of</strong> 42 (Euler’s constant)<br />
There are a variety <strong>of</strong> pro<strong>of</strong>s <strong>of</strong> divergence <strong>of</strong> <strong>the</strong> harmonic series that in some way make use <strong>of</strong> <strong>the</strong> Euler-<br />
Mascheroni constant. This constant is <strong>of</strong>ten defined by <strong>the</strong> following limit:<br />
<br />
n<br />
1<br />
γ = lim<br />
n→∞ k −ln(n)<br />
<br />
≈ 0.5772156649.<br />
k=1<br />
Once this limit has been established (see for example [14, page 623, exercise 75]), it is clear that <strong>the</strong> partial<br />
sums, Hn = n k=1 1<br />
k<br />
, are unbounded.<br />
Here is a different pro<strong>of</strong> involving γ. Let n be a fixed positive integer. For k = 1,2,3,..., let<br />
For example,<br />
and<br />
Since<br />
we have<br />
Sn−1 = 1<br />
n−1 −<br />
Sk = 1<br />
k −<br />
n<br />
j=1<br />
1<br />
kn+j .<br />
S1 = 1− 1 1 1<br />
− −···−<br />
n+1 n+2 2n ,<br />
S2 = 1 1 1 1<br />
− − −···−<br />
2 2n+1 2n+2 3n ,<br />
1<br />
(n−1)n+1 −<br />
n<br />
kn+n <<br />
n<br />
j=1<br />
1<br />
kn+j<br />
1 1<br />
−···−<br />
(n−1)n+2 n2. < n<br />
kn+1 ,<br />
0 < 1 n<br />
−<br />
k kn+1 < Sk < 1 n 1 1<br />
− = −<br />
k kn+n k k +1 .<br />
It follows that <strong>the</strong> sequence { m<br />
k=1 Sk} ∞ m=1 is increasing, bounded above by ∞<br />
k=1<br />
<br />
1 1<br />
k − k+1<br />
<strong>the</strong>refore convergent to a positive number no greater than 1. The limit is in fact γ (see [16]).<br />
Now notice that<br />
S1 +S2 +···Sn−1 + 1<br />
n = 2Hn −H n 2.<br />
Assuming that <strong>the</strong> harmonic series converges to H and taking <strong>the</strong> limit as n → ∞, we have<br />
an obvious contradiction.<br />
Some additional pro<strong>of</strong>s<br />
γ = 2H −H = H,<br />
= 1, and<br />
Here are a couple <strong>of</strong> pro<strong>of</strong>s involving probability <strong>the</strong>ory. Because <strong>the</strong>y are too advanced for <strong>the</strong> typical<br />
calculus student, <strong>the</strong>y are not duplicated here.<br />
• 1<br />
n = ∞: A Micro-Lesson on Probability and Symmetry by Omer Adleman, Amer. Math. Monthly,<br />
November 2007, pages 809–810<br />
16
• A Pro<strong>of</strong> <strong>of</strong> <strong>Divergence</strong> <strong>of</strong> <strong>the</strong> <strong>Harmonic</strong> <strong>Series</strong> Using Probability Theory by Arnab Kumar Laha, Inter-<br />
national Journal <strong>of</strong> Ma<strong>the</strong>matical Education in Science and Technology, June 2006, pages 502–503<br />
References<br />
[1] E. R. Bobo, A sequence related to <strong>the</strong> harmonic series, <strong>College</strong> Ma<strong>the</strong>matics Journal, 26 (1995),<br />
pp. 308–310.<br />
[2] D. M. Bradley, A note on <strong>the</strong> divergence <strong>of</strong> <strong>the</strong> harmonic series, American Ma<strong>the</strong>matical Monthly,<br />
107 (2000), p. 651.<br />
[3] H. Chen and C. Kennedy, <strong>Harmonic</strong> series meets Fibonacci sequence, The <strong>College</strong> Ma<strong>the</strong>matics<br />
Journal, 43 (2012), pp. 237–243.<br />
[4] A. Cusumano, Generalization <strong>of</strong> Oresme’s standard method <strong>of</strong> showing <strong>the</strong> divergence <strong>of</strong> <strong>the</strong> harmonic<br />
series, American Ma<strong>the</strong>matical Monthly, 119 (2012), p. 210.<br />
[5] A. De Morgan, On <strong>the</strong> summation <strong>of</strong> divergent series, The Assurance Magazine, and <strong>the</strong> Journal <strong>of</strong><br />
<strong>the</strong> Institute <strong>of</strong> Actuaries, 12 (1865), pp. 245–252.<br />
[6] W. Dunham, Euler: The Master <strong>of</strong> Us All, The Ma<strong>the</strong>matical Association <strong>of</strong> America, 1999.<br />
[7] P. Erdős, Über die Reihe 1<br />
p , Ma<strong>the</strong>matica, Zutphen B 7 (1938), pp. 1–2.<br />
[8] A. Fearnehough, Ano<strong>the</strong>r method for showing <strong>the</strong> divergence <strong>of</strong> <strong>the</strong> harmonic series, The Ma<strong>the</strong>mat-<br />
ical Gazette, 75 (1991), p. 198.<br />
[9] J. Havil, Gamma: Exploring Euler’s Constant, Princeton University Press, 2003.<br />
[10] I. I. Hirschman Jr., Infinite <strong>Series</strong>, Holt, Rinehart and Winston, 1962.<br />
[11] S. J. Kifowit and D. T. Clancy, A closer look at Bobo’s sequence. To appear.<br />
[12] S. J. Kifowit and T. A. Stamps, Serious About <strong>the</strong> <strong>Harmonic</strong> <strong>Series</strong> II. Presentedat <strong>the</strong>31stAnnual<br />
Conference <strong>of</strong> <strong>the</strong> Illinois Ma<strong>the</strong>matics Association <strong>of</strong> Community <strong>College</strong>s, Monticello, IL, March 31,<br />
2006. Available at http://prairiestate.edu/skifowit/al1.pdf.<br />
[13] , The harmonic series diverges again and again, The AMATYC Review, 27 (2006), pp. 31–43.<br />
[14] R. Larson, R. P. Hostetler, and B. H. Edwards, Calculus, Houghton Mifflin Company, 8th ed.,<br />
2006.<br />
[15] N. Lord, Maths bite: seeing <strong>the</strong> divergence <strong>of</strong> <strong>the</strong> harmonic series, The Ma<strong>the</strong>matical Gazette, 87<br />
(2003), pp. 125–126.<br />
[16] J. J. Mačys, A new problem, American Ma<strong>the</strong>matical Monthly, 119 (2012), p. 82.<br />
17
[17] R. B. Nelson, <strong>Pro<strong>of</strong>s</strong> Without Words II: More Exercises in Visual Thinking, The Ma<strong>the</strong>matical As-<br />
sociation <strong>of</strong> America, 2000.<br />
[18] J. C. Owings Jr., Ano<strong>the</strong>r pro<strong>of</strong> <strong>of</strong> <strong>the</strong> Egyptian fraction <strong>the</strong>orem, American Ma<strong>the</strong>matical Monthly,<br />
75 (1968), pp. 777–778.<br />
[19] J. Stewart, Calculus, Brooks/Cole, 5th ed., 2003.<br />
[20] A. J. B. Ward, <strong>Divergence</strong> <strong>of</strong> <strong>the</strong> harmonic series, The Ma<strong>the</strong>matical Gazette, (C) (1970), p. 277.<br />
18