Math 1300 Written Homework #10 Solutions 4.2, Q. 24. Let y = at 2e ...
Math 1300 Written Homework #10 Solutions 4.2, Q. 24. Let y = at 2e ...
Math 1300 Written Homework #10 Solutions 4.2, Q. 24. Let y = at 2e ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
<strong>M<strong>at</strong>h</strong> <strong>1300</strong> <strong>Written</strong> <strong>Homework</strong> <strong>#10</strong> <strong>Solutions</strong><br />
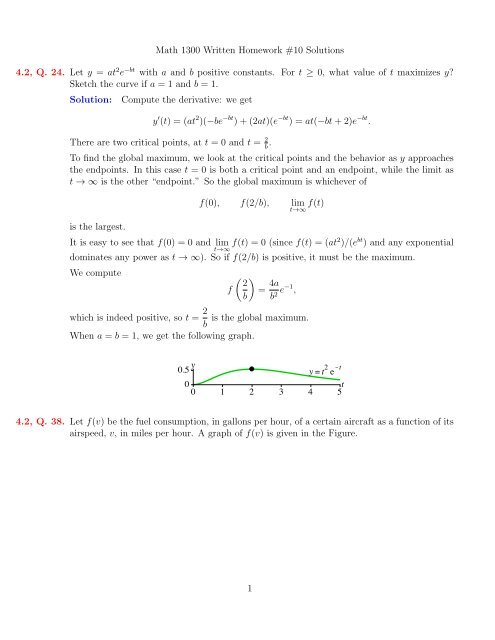
<strong>4.2</strong>, Q. <strong>24.</strong> <strong>Let</strong> y = <strong>at</strong> 2 e −bt with a and b positive constants. For t ≥ 0, wh<strong>at</strong> value of t maximizes y?<br />
Sketch the curve if a = 1 and b = 1.<br />
Solution: Compute the deriv<strong>at</strong>ive: we get<br />
y ′ (t) = (<strong>at</strong> 2 )(−be −bt ) + (2<strong>at</strong>)(e −bt ) = <strong>at</strong>(−bt + 2)e −bt .<br />
There are two critical points, <strong>at</strong> t = 0 and t = 2<br />
b .<br />
To find the global maximum, we look <strong>at</strong> the critical points and the behavior as y approaches<br />
the endpoints. In this case t = 0 is both a critical point and an endpoint, while the limit as<br />
t → ∞ is the other “endpoint.” So the global maximum is whichever of<br />
is the largest.<br />
f(0), f(2/b), lim<br />
t→∞ f(t)<br />
It is easy to see th<strong>at</strong> f(0) = 0 and lim<br />
t→∞ f(t) = 0 (since f(t) = (<strong>at</strong> 2 )/(e bt ) and any exponential<br />
domin<strong>at</strong>es any power as t → ∞). So if f(2/b) is positive, it must be the maximum.<br />
We compute<br />
f<br />
<br />
2<br />
=<br />
b<br />
4a<br />
b2 e−1 ,<br />
which is indeed positive, so t = 2<br />
is the global maximum.<br />
b<br />
When a = b = 1, we get the following graph.<br />
<strong>4.2</strong>, Q. 38. <strong>Let</strong> f(v) be the fuel consumption, in gallons per hour, of a certain aircraft as a function of its<br />
airspeed, v, in miles per hour. A graph of f(v) is given in the Figure.<br />
1
a. <strong>Let</strong> g(v) be the fuel consumption of the same aircraft, but measured in gallons per mile<br />
instead of gallons per hour. Wh<strong>at</strong> is the rel<strong>at</strong>ionship between f(v) and g(v)?<br />
Solution: Suppose v = 100 miles per hour; then from the graph, f(100) = 50 gallons<br />
per hour. So in the next one hour, the aircraft will travel about 100 miles and use up<br />
about 50 gallons of fuel. Assuming the speed and fuel consumption stay constant over<br />
th<strong>at</strong> hour, the plane is using 50 1 = gallons of fuel per mile traveled.<br />
100 2<br />
More generally,<br />
g(v) = = f(v) gallons<br />
v mile .<br />
f(v) gallons<br />
hour<br />
v miles<br />
hour<br />
Notice how the units “gallons per hour” divided by the units “miles per hour” cancels out<br />
to give “gallons per mile,” which are the units we were supposed to get. Units confirm<br />
th<strong>at</strong> we are thinking about the problem in the right way.<br />
b. For wh<strong>at</strong> value of v is f(v) minimized?<br />
Solution: We just want the critical point, where f ′ (v) = 0. This seems to occur around<br />
v = 220 miles per hour.<br />
c. For wh<strong>at</strong> value of v is g(v) minimized?<br />
Solution: g(v) will have the same general shape as f(v), with a vertical asymptote <strong>at</strong><br />
v = 0 and increasing toward infinity as v goes to infinity. So we just need the critical<br />
point of g(v).<br />
Using the Quotient Rule, we get<br />
g ′ (v) = d<br />
dv<br />
<br />
f(v)<br />
v<br />
= vf ′ (v) − f(v)<br />
v2 .<br />
The critical point we care about is wherever f(v) = vf ′ (v). The best way to see this<br />
from the graph is to notice th<strong>at</strong> geometrically, we will have<br />
f(v)<br />
v = f ′ (v)<br />
2
when the line from the origin to (v, f(v)) coincides with the tangent line <strong>at</strong> (v, f(v)).<br />
(Or in other words, when the tangent line to the graph passes through the origin.)<br />
From the graph this seems to happen when v ≈ 300 miles per hour.<br />
d. Should a pilot try to minimize f(v) or g(v)?<br />
Solution: Presumably the pilot intends to go the same speed for almost the entire trip,<br />
which means f(v) and g(v) will both be constants for the pilot. Th<strong>at</strong> means the total<br />
fuel used will be either f(v) times the number of hours the trip takes, or g(v) times the<br />
number of miles the trip takes.<br />
Now the number of hours the trip takes depends on the speed (so it’s not constant!), but<br />
the number of miles the trip takes is the same no m<strong>at</strong>ter wh<strong>at</strong> the speed is. So I would<br />
expect th<strong>at</strong> a pilot wants to minimize g(v), the fuel used per mile traveled.<br />
3
<strong>4.2</strong>, Q. 40. The Figure gives the deriv<strong>at</strong>ive of g(x) on −2 ≤ x ≤ 2.<br />
a. Write a few sentences describing the behavior of g(x) on this interval.<br />
Solution: First of all, g ′ (x) is always less than or equal to zero. Th<strong>at</strong> means the<br />
original function g(x) is always decreasing on the interval [−2, 2]. Furthermore g ′ (x) is<br />
decreasing from −2 to 0, then increases from 0 to 2. Th<strong>at</strong> means g(x) is concave down<br />
from −2 to 0 and concave up from 0 to 2.<br />
b. Does the graph of g(x) have any inflection points? If so, give the approxim<strong>at</strong>e xcoordin<strong>at</strong>es<br />
of their loc<strong>at</strong>ions. Explain your reasoning.<br />
Solution: The inflection point of g(x) is <strong>at</strong> x = 0, where it changes concavity.<br />
It is possible th<strong>at</strong> x = −2 and x = 2 would also be inflection points of the full graph, but<br />
they do not count here since the function does not actually change concavity <strong>at</strong> x = 2:<br />
g ′ (x) doesn’t even seem to exist for x > 2, and even if it did, we have no idea whether<br />
g ′ (x) decreases or increases for x > 2.<br />
c. Wh<strong>at</strong> are the global maxima and minima of g on [−2, 2]?<br />
Solution: The global maximum of g(x) occurs <strong>at</strong> x = −2, since g(x) is decreasing from<br />
−2 to 2. Similarly the global maximum occurs <strong>at</strong> x = 2.<br />
d. If g(−2) = 5, wh<strong>at</strong> do you know about g(0) and g(2)? Explain.<br />
Solution: I know th<strong>at</strong> g(0) is smaller than 5 and th<strong>at</strong> g(2) is even smaller than g(0).<br />
There are no vertical units on the graph, so there is no way of knowing just how much<br />
smaller g(0) is.<br />
The one thing you could say is th<strong>at</strong><br />
g(2) − g(0) = g(0) − g(−2),<br />
or in other words th<strong>at</strong> g(2) = 2g(0) − 5. The reason for this is basically th<strong>at</strong> g(x) is not<br />
necessarily an odd function, but since its deriv<strong>at</strong>ive is even, it has to be a vertical shift<br />
of an odd function. Th<strong>at</strong> means the secant line from −2 to 0 must have the same slope<br />
as the secant line from 0 to 2. This is a bit more sophistic<strong>at</strong>ed though.<br />
4.3, Q. 39. a. Find all critical points of f(x) = x 4 + ax 2 + b.<br />
Solution: The deriv<strong>at</strong>ive is<br />
f ′ (x) = 4x 3 + 2ax.<br />
This always exists, so to find critical points we set it to zero.<br />
4x 3 + 2ax = 0<br />
2x(2x 2 + a) = 0.<br />
4
We see th<strong>at</strong> x = 0 is always a critical point. Whether there are other critical points<br />
depends on the sign of a. If a < 0 then x = ± (−a/2) are the two other critical points<br />
(and so there are three). If a = 0 then we just get x = 0 again (so there is only one).<br />
Finally if a > 0 then we get no additional critical points (so there is just the one <strong>at</strong><br />
x = 0).<br />
b. Under wh<strong>at</strong> conditions on a and b does this function have exactly one critical point?<br />
Wh<strong>at</strong> is the one critical point, and is it a local maximum, a local minimum, or neither?<br />
Solution: It has exactly one critical point when a ≥ 0. In this case x = 0 is the one<br />
critical point. To test whether it is a local maximum or local minimum, we plug in values<br />
to the left and right. For example when x = −1 we get f ′ (−1) = −2(2 + a) which is<br />
neg<strong>at</strong>ive since a ≥ 0. Similarly f ′ (1) = 2(2 + a) > 0. So the function is going from<br />
decreasing to increasing, and thus x = 0 is a local minimum.<br />
c. Under wh<strong>at</strong> conditions on a and b does this function have exactly three critical points?<br />
Wh<strong>at</strong> are they? Which are local maxima and which are local minima?<br />
Solution: It has exactly three critical points if a < 0. They are x = 0, x = − (−a/2),<br />
and x = (−a/2). To see which are local maxima and which are local minima, we use<br />
the second deriv<strong>at</strong>ive test. We have f ′′ (x) = 12x 2 + 2a, so th<strong>at</strong><br />
f ′′ (0) = 2a, f ′′ (− (−a/2)) = −4a, f ′′ ( (−a/2)) = −4a.<br />
Now a < 0, so th<strong>at</strong> means f ′′ is neg<strong>at</strong>ive <strong>at</strong> x = 0 and positive <strong>at</strong> the two other critical<br />
points. Th<strong>at</strong> means x = 0 is a local maximum and x = ± (−a/2) are both local<br />
minima.<br />
d. Is it ever possible for this function to have two critical points? No critical points? More<br />
than three critical points? Give an explan<strong>at</strong>ion in each case.<br />
Solution: None of these things are ever possible. The deriv<strong>at</strong>ive is of the form 2x(2x 2 +<br />
a). So it either has one root or three roots, depending on whether 2x 2 + a can ever be<br />
zero. And if 2x 2 + a can be zero, it must be zero twice (since the neg<strong>at</strong>ive of any root is<br />
also a root).<br />
5
4.3, Q. 50. Consider the family<br />
y = A<br />
x + B .<br />
a. If B = 0, wh<strong>at</strong> is the effect of varying A on the graph?<br />
Solution: We have the family y = A<br />
. Varying A corresponds to rescaling the y-values<br />
x<br />
of the graph, so increasing A will stretch the graph vertically. It will still have the same<br />
basic shape (vertical asymptote <strong>at</strong> x = 0, horizontal asymptote <strong>at</strong> y = 0).<br />
b. If A = 1, wh<strong>at</strong> is the effect of varying B?<br />
Solution: We have the family y = 1<br />
, which has a vertical asymptote <strong>at</strong> x = −B.<br />
x + B<br />
Varying B moves this vertical asymptote, but does not change the shape of the graph.<br />
c. On one set of axes, graph the function for several values of A and B.<br />
Solution: Here I have graphed: A = 1, B = 1 in red, A = 2, B = 1 in blue, A = 1,<br />
B = −2 in yellow, and A = −1, B = −2 in green.<br />
4.3, Q. 54. For positive A, B, the force between two <strong>at</strong>oms is a function of the distance, r, between them:<br />
f(r) = − A B<br />
+<br />
r2 r3 r > 0<br />
a. Wh<strong>at</strong> are the zeros and asymptotes of f?<br />
Solution: It’s easier to answer this by getting a common denomin<strong>at</strong>or:<br />
f(r) =<br />
B − Ar<br />
r3 .<br />
This is zero when r = B/A, and it has a vertical asymptote when r = 0. It has a<br />
horizontal asymptote <strong>at</strong> y = 0.<br />
6
. Find the coordin<strong>at</strong>es of the critical points and inflection points of f.<br />
Solution: To take the deriv<strong>at</strong>ive, we write f(r) = −Ar −2 + Br −3 so we can use the<br />
Power Rule. We get<br />
and<br />
f ′ (r) = 2Ar −3 − 3Br −4 =<br />
f ′′ (r) = −6Ar −4 + 12Br −5 =<br />
2Ar − 3B<br />
r 4<br />
12B − 6Ar<br />
r5 .<br />
The critical points are r = 0 and r = 3B<br />
2B<br />
. The inflection points are r = 0 and r =<br />
2A A .<br />
c. Graph f.<br />
Solution: Here it is, with the zero, the minimum, and the inflection point labeled.<br />
d. Illustr<strong>at</strong>ing your answers with a sketch, describe the effect on the graph of f of:<br />
(a) Increasing B, holding A fixed<br />
(b) Increasing A, holding B fixed<br />
Solution: The fact th<strong>at</strong> our three interesting x values occur <strong>at</strong> multiples of B/A means<br />
th<strong>at</strong> increasing B moves those points to the right, and increasing A moves those points<br />
to the left. More subtly, the y-value <strong>at</strong> the minimum can be computed: plug in r = 3B<br />
2A<br />
to the formula to get f( 3B 4A3 ) = − 2A 27B2 . Thus increasing B will shrink th<strong>at</strong> minimum value<br />
toward the x-axis, while increasing A will move it away from the x-axis.<br />
4.4, Q. <strong>24.</strong> Find the minimal and maximal value of x in Figure 4.54.<br />
Solution: 0 ≤ x ≤ 10, so the minimum is 0 and the maximum 10.<br />
4.4, Q. 38. The cross-section of a tunnel is a rectangle of height h surmounted by a semicircular roof<br />
section of radius r (see figure 4.56). If the cross-sectional area is A, determine the dimensions<br />
of the cross section which minimize the perimeter.<br />
7
Solution: The perimeter P = 2r+2h+πr (the bottom, plus the 2 sides, plus the semicircular<br />
arc on top). The area A = 2rh + πr 2 /2 is fixed, so we can solve th<strong>at</strong> h = (A − πr 2 /2)/2r,<br />
getting th<strong>at</strong><br />
P = r(2 + π) + 2(A − πr 2 /2)/2r = r(2 + π/2) + A/r,<br />
where r > 0 and r < 2A/π (so h > 0). We find critical points by differenti<strong>at</strong>ing P<br />
with respect to r, yielding dP<br />
dR = 2 + π/2 − A/r2 , which exists since r > 0, and is 0 when<br />
r2 = A/(2 + π/2), so r = A/(2 + π/2). Since there is precisely 1 critical point, and the<br />
deriv<strong>at</strong>ive is neg<strong>at</strong>ive for r < A/(2 + π/2) and positive for r > A/(2 + π/2), we have<br />
th<strong>at</strong> r = A/(2 + π/2) produces the minimum perimeter, and<br />
h = (A − πA/(4 + π))/2 A/(2 + π/2) = √ A/(2 + π/2) 3/2 .<br />
4.4, Q. 40. Of all rectangles with given area, A, which has the shortest diagonals?<br />
Solution: Say the rectangle is x by y, so xy = A. Then the diagonal length we want to<br />
mimimize is x 2 + y 2 . This is the same as minimizing s = x 2 + y 2 , which is much easier to<br />
handle algebraically. Since y = A/x, we have<br />
s = x 2 + A 2 /x 2 ,<br />
where x > 0. We compute ds<br />
dx = 2x − 2A2 /x 3 , which exists everywhere, and is zero when<br />
x 4 = A 2 , so x = √ A. Since the deriv<strong>at</strong>ive is neg<strong>at</strong>ive for x < √ A and positive for x > √ A,<br />
this is a minimum. Note th<strong>at</strong> y = A/ √ A = √ A. So the square has the shortest diagonals for<br />
a given area.<br />
8