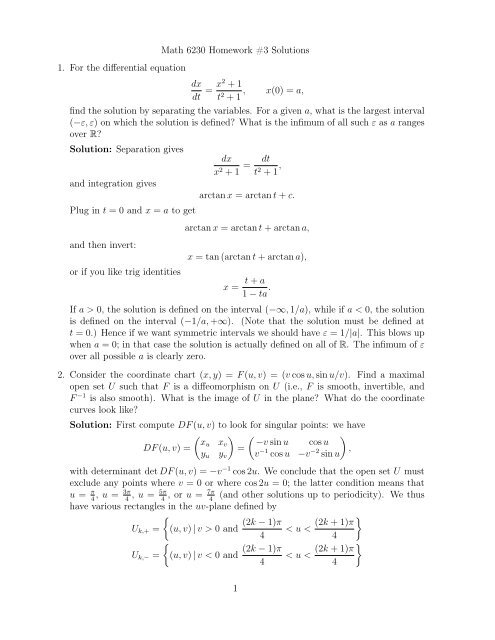
Math 6230 Homework #3 Solutions 1. For the differential equation ...

1. For the differential equation
Math 6230 Homework #3 Solutions
dx
dt = x2 + 1
t2 , x(0) = a,
+ 1
find the solution by separating the variables. For a given a, what is the largest interval
(−ε, ε) on which the solution is defined? What is the infimum of all such ε as a ranges
over R?
Solution: Separation gives
and integration gives
Plug in t = 0 and x = a to get
and then invert:
or if you like trig identities
dx
x 2 + 1
= dt
t 2 + 1 ,
arctan x = arctan t + c.
arctan x = arctan t + arctan a,
x = tan (arctan t + arctan a),
x =
t + a
1 − ta .
If a > 0, the solution is defined on the interval (−∞, 1/a), while if a < 0, the solution
is defined on the interval (−1/a, +∞). (Note that the solution must be defined at
t = 0.) Hence if we want symmetric intervals we should have ε = 1/|a|. This blows up
when a = 0; in that case the solution is actually defined on all of R. The infimum of ε
over all possible a is clearly zero.
2. Consider the coordinate chart (x, y) = F (u, v) = (v cos u, sin u/v). Find a maximal
open set U such that F is a diffeomorphism on U (i.e., F is smooth, invertible, and
F −1 is also smooth). What is the image of U in the plane? What do the coordinate
curves look like?
Solution: First compute DF (u, v) to look for singular points: we have
xu
DF (u, v) =
xv −v sin u
=
v
cos u
−1 cos u −v−2
,
sin u
yu yv
with determinant det DF (u, v) = −v−1 cos 2u. We conclude that the open set U must
exclude any points where v = 0 or where cos 2u = 0; the latter condition means that
u = π 3π 5π
7π
, u = , u = , or u = (and other solutions up to periodicity). We thus
4 4 4 4
have various rectangles in the uv-plane defined by
(2k − 1)π (2k + 1)π
Uk,+ = (u, v) | v > 0 and < u <
4
4
(2k − 1)π (2k + 1)π
Uk,− = (u, v) | v < 0 and < u <
4
4
1

with 1 ≤ k ≤ 4 being the relevant ones.
We know that F is locally a diffeomorphism on Uk,+ and Uk,−, so we just need to
prove F is bijective in order to make it a global diffeomorphism. To do this, suppose
F (u, v) = F (p, q); then v cos u = q cos p and v −1 sin u = q −1 sin p. Multiplying these
together we obtain sin 2u = sin 2p. Using the trick A = u + p and B = u − p, we get
sin (A + B) = sin (A − B), and expanding gives either sin (u − p) = 0 or cos (u + p) =
0. Thus we have the solutions u = p + nπ or u = −p + (n + 1
2 )π.
In the first case where u = p + nπ we have (−1) n v cos p = q cos p and v −1 (−1) n sin p =
q −1 sin p, which means v = (−1) n q. So either u = p up to a multiple of 2π and v = q,
or u = p + π up to a multiple of 2π and v = −q. Either way we cannot have both
points in the same set Uk,±.
In the second case where u = −p + (n + 1
2 )π, we must have cos u = (−1)n sin p and
sin u = (−1) n cos p, so that the two conditions reduce to (−1) n v sin p = q cos p. Can
two such points be in the same set? Suppose (2k − 1)π/4 < p < (2k + 1)π/4; then
(n + 1/4 − k/2)π < u < (n + 3/4 − k/2)π, and this is in the same interval as p if and
only if n + 1/2 − k = 0, which is impossible.
We conclude that F is actually one-to-one on each of the sets Uk,±.
To find the image under F of one of these sets (for example U1,+), we look at the
coordinate curves. For fixed u and positive v, the map v ↦→ (v cos u, v−1 sin u) = (x, y)
traces out a hyperbola satisfying xy = 1 sin 2u. Hence all points that can be in the
2
image of F must satisfy |xy| ≤ 1,
which severely constrains the set. When u = π/4
2
we get the hyperbola xy = 1/2 in the first quadrant, and when u = 3π/4 we get the
hyperbola xy = −1/2 in the second quadrant. For fixed v > 0 and π/4 < u < 3π/4, the
map u ↦→ (v cos u, v−1 sin u) traces out a portion of an ellipse satisfying x2 /v2 +v2y2 = 1,
between the points ( v √ 2 , 1
v √ 2 ) and (− v √ 2 , 1
v √ 2 ).
We can obtain every point in the upper half plane lying below the hyperbolas y = 1/2|x|
by just writing down formulas: it’s easy to see that we have u = 1(π
− arcsin (2xy)) in
2
order to have π
3π < u < , and using the half-angle formula for sines and cosines, we
4 4
get
1 −
v =
1 − 4x2y2 √
2x
√ =
2y
1 + 1 − 4x2y2 if x and y are both positive, and a similar formula if x < 0.
The image of coordinate curves under the map is shown below.
3. Classically, the tangent space to a surface S ⊂ R 3 at a point p ∈ S is the set of all
possible vectors γ ′ (0) such that γ(t) is a smooth curve defined on a neighborhood of
t = 0 with γ(0) = p.
If the surface is defined by the formula F (x, y, z) = xy − 3x 2 z + zyx = 0, compute the
tangent space at (1, 2, 2) and find a basis for it.
2

Figure 1: Some u-v coordinate lines in the set U1,+ = ( π 3π , )×(0, ∞), and their image under
4 4
the map. Blue curves are portions of ellipses, and red curves are portions of hyperbolas.
Solution: Suppose γ(t) = (x(t), y(t), z(t)) is a curve lying in the surface S; then for
all time we have
x(t)y(t) − 3x(t) 2 z(t) + z(t)y(t)x(t) = 0.
We assume γ(0) = p = (1, 2, 2), so that x(0) = 1, y(0) = 2, and z(0) = 2. Differentiating
the constraint equation we get
x ′ (t)y(t)+x(t)y ′ (t)−6x(t)x ′ (t)z(t)−3x(t) 2 z ′ (t)+z ′ (t)y(t)x(t)+z(t)y ′ (t)x(t)+z(t)y(t)x ′ (t) = 0.
If x ′ (0) = a, y ′ (0) = b, and z ′ (0) = c, then plugging in t = 0 gets us
or −6a + 3b − c = 0.
2a + b − 12a − 3c + 2c + 2b + 4a = 0,
We obtain the same answer in the language of vector calculus by computing the gradient:
∇F |(x,y,z) = (Fx, Fy, Fz) = (y − 6xz + zy, x + zx, −3x 2 + yx).
Thus ∇F |(1,2,2) = (−6, 3, −1), and we have ∇F |(1,2,2) · γ ′ (0) = 0. Of course, this is
precisely what the Chain Rule says.
Since (a, b, c) ∈ TpS if c = −6a + 3b, a basis is formed for example by the vectors
(1, 0, −6) and (0, 1, 3).
4. Suppose F (x, y) = x 2 y − y 3 − 2x. If G satisfies F (x, G(x)) = 0, compute G ′ (x) and
G ′′ (x) in terms of G.
Solution: Just implicit differentiation. We have x 2 G(x) − G(x) 3 − 2x = 0, so that
x 2 G ′ (x) + 2xG(x) − 3G(x) 2 G ′ (x) − 2 = 0. (1)
3

Solving for G ′ (x) gives
G ′ (x) =
2 − 2xG(x)
x2 . (2)
− 3G(x) 2
There are two ways to obtain G ′′ (x): either differentiate (1), solve for G ′′ (x), and plug
in (2), or differentiate (2) and then plug in (2). I’ll use the first method since I don’t
much care for the quotient rule.
We obtain
x 2 G ′′ (x) + 4xG ′ (x) + 2G(x) − 6G(x)G ′ (x) 2 − 3G(x) 2 G ′′ (x) = 0,
and solving for G ′′ (x) gives
Then plugging in G ′ (x) from (2) gives
G ′′ (x) =
G ′′ (x) = 6G(x)G′ (x) 2 − 2G(x) − 4xG ′ (x)
x2 − 3G(x) 2 .
6G(x)
2−2xG(x)
x2−3G(x) 2
2
2−2xG(x)
− 2G(x) − 4x x2−3G(x) 2
x 2 − 3G(x) 2
5. The images here are the lower-case and upper-case alphabet in Helvetica font.
Classify the lower-case letters by homeomorphism type. For which letters is the uppercase
version not homeomorphic to the lower-case version?
Solution: The equivalence classes for the lower-case letters are
{a, b, d, g, p, q}, {c, l, s, v, w, z}, {e}, {f, t, x}, {h, n, r, u, y}, {i, j}, {k, m}, {o}.
The only one that’s not quite obvious is that ‘k’ is homeomorphic to ‘m’, but if you
think about it carefully you’ll see the correspondence.
The lower-case letters that are homeomorphic to their upper-case versions are:
{a, c, k, l, o, s, t, v, w, x, y, z}.
4
.

6. Consider the parabolic coordinates (ϕ, U) given by formula (6.2.4) with open set U
given by the complement of the negative vertical ray. Let fpara(τ, σ) = τ 2 be defined
on the set R×(0, ∞). Is there a smooth function f : M → R such that fpara = f ◦ϕ −1 ?
Solution: Let ψ : M → R 2 be the Euclidean coordinates (x, y) on the plane. Then
the transition map is, by equation (6.2.4),
(x, y) = φ ◦ ϕ −1 (τ, σ) = στ, 1
2 (τ 2 − σ 2 ) . (3)
Now define f : U → R by f(p) = fpara◦ϕ(p) = fpara(τ, σ) if (τ, σ) = ϕ(p). The question
is whether we can complete f to all of M, and in order to determine this, we need to
compute f in another coordinate chart.
Define frect : φ[U] → R by frect(x, y) = f ◦ φ −1 (x, y). We know that φ[U] consists of
all (x, y) except the ray L = {(0, y) | y ≤ 0} (in other words, the negative vertical ray
expressed in coordinates). We compute
frect(x, y) = f ◦ φ −1 (x, y) = fpara ◦ ϕ ◦ φ −1 (x, y).
Now the function (τ, σ) = ϕ ◦ φ−1 (x, y) is just the inverse of the function (3), defined
on L, with values τ > 0 and σ ∈ R. To compute it, just solve (3) to get
⎛
(τ, σ) = ⎝ y + x2 + y2 x
,
y + x2 + y2 ⎞
⎠ .
Since fpara(τ, σ) = τ 2 , we conclude that
frect(x, y) = τ(x, y) 2 = y + x 2 + y 2
on the complement of L, i.e., as long as x = 0, or x = 0 and y > 0.
The question is now whether frect extends to all of R 2 ; if it does, then f = frect ◦ φ is
defined on all of M since φ is defined on all of M. Unfortunately frect is not smooth
in any neighborhood of the origin; we have
f(0, y) = y + |y| =
0 y ≤ 0
2y y > 0 ,
so that fy(0, 0) does not exist. Hence although there is a continuous f : M → R, it
cannot be smooth in coordinate charts.
7. Recall that a topological space M is second-countable if there is a countable collection
{Ωn : n ∈ N} of open sets such that every other open set U ⊂ M is equal to the union
of all the sets Ωn that it contains.
(a) Prove that R is second-countable. (Hint: the rationals are countable.)
Solution: Consider the set of all open intervals (a, b) such that a, b ∈ Q; this is a
subset of Q × Q, so it is a countable collection. Let U ⊂ R be any open set. Let
5

N = {(a, b) ∈ Q × Q | a < b and (a, b) ⊂ U} be an at-most-countable subset of
Q × Q, the set of all rational endpoints of open intervals contained in U. Define
V =
(a,b)∈N (a, b) to be the union of these intervals.1 Clearly V ⊂ U. We need
to show U ⊂ V .
Let x ∈ U be any point. There is an ε > 0 such that (x − ε, x + ε) ⊂ U, and
by density of the rationals, there are rationals a ∈ (x − ε, x) and b ∈ (x, x + ε).
Hence x ∈ (a, b) ⊂ (x − ε, x + ε) ⊂ U, and thus x ∈ V . We conclude that U ⊂ V ,
so U = V . Hence every open set is the union of the rational-endpoint intervals it
contains.
(b) A topological space M is Lindelöf if for every every family of open sets that
covers M, there is a countable subcover that still covers M. Prove that every
second-countable space is Lindelöf.
Solution: Let W = {Ωn | n ∈ N} be a countable basis, and let Y = {Yi | i ∈ I}
be a family of open sets that covers M for some uncountable set I.
Let C be the subset of N such that n ∈ C if Ωn ⊂ Yi for some i ∈ I; let f : C → I
be a function that picks an i for which this is true. In other words Ωn ⊂ Yf(n).
Obviously C is countable; I claim that the union Q =
n∈C Yf(n) covers M.
So let x ∈ M be any point; then x ∈ Yi for some i ∈ I. Since W is a basis, there is
some n such that x ∈ Ωn ⊂ Yi. Now perhaps i = f(n), but since Ωn is contained
in some set in Y, we know n ∈ C. Thus since x ∈ Ωn and Ωn ⊂ Yf(n), we know
x ∈ Q. Thus M = Q and we have found a countable subfamily of Y that covers
M.
8. Suppose we have a collection of bijective functions (φ, U) from a set M to R n such
that the union of all U covers M and whenever U ∩ V is nonempty, the function
φ ◦ ψ −1 : ψ[U ∩ V ] ⊂ R n → φ[U ∩ V ] ⊂ R n is a homeomorphism. Define a set Ω ⊂ M
to be open if and only if φ[Ω ∩ U] is open for every chart (φ, U). Check carefully that
this satisfies the conditions for a topology on M.
Solution: We check the three conditions.
• M and ∅ are open. M is open since φ[M ∩ U] = R n is open in itself for every
(φ, U). And ∅ is open since φ[∅ ∩ U] = ∅ is open in R n .
• If Ω and Ψ are open, then Ω ∩ Ψ is open. This comes from the formula
Now since φ is a bijection, we have
φ[(Ω ∩ Ψ) ∩ U] = φ[(Ω ∩ U) ∩ (Ω ∩ V )].
φ[(Ω ∩ U) ∩ (Ω ∩ V )] = φ[Omega ∩ U] ∩ φ[Ω ∩ V ],
and by assumption the latter two sets are open in R n . So their intersection is
open as well, as desired.
1 It is annoying that (a, b) means both an ordered pair and an open interval.
6

• If Ωi is a collection of open sets for i ∈ I for some index set I, then ∪i∈IΩi is
open. The DeMorgan law says that U ∩ (∪i∈IΩi) = ∪i∈I(U ∩ Ωi), and since φ is
a bijection we again have
φ[U ∩ (∪i∈IΩi)] = ∪i∈Iφ[U ∩ Ωi].
The latter is open since it is the union of open sets in R n .
Note that we never actually need to use the condition that φ◦ψ −1 is a homeomorphism
on its domain to get a topology on M. But if that condition is not satisfied, we may get
a strange-looking manifold. For example, let M ∼ = R (abstractly) with ψ the identity
map, and let φ be any discontinuous bijection such as
⎧
⎪⎨ x x < 0
φ(x) = 1 − x 0 ≤ x ≤ 1
⎪⎩
x x > 1.
Now for 0 < ε < 1, the set (1 − ε, 1 + ε) ⊂ M is not open in this topology since
φ(1 − ε, 1 + ε) = [0, ε) ∪ (1, 1 + ε)[0, 1) ∪ (1, 2) is not open in R. More generally there
are no “small” open sets containing the point 1 ∈ M. Thus for example the induced
topology on M is not Hausdorff: there is no open set containing 1 that does not contain
1
1 1 1
, and the sequence ( , , , . . .) converges to 1. Furthermore, in such a topology the
2 2 2 2
map φ: M → R is not even continuous!
9. Suppose we are in the same situation as the previous problem. Show that a function
f : M → R is continuous if and only if, for every chart (φ, U), the usual multivariable
function f ◦ φ −1 : φ[U] ⊂ R n → R is continuous.
Solution: Since φ is a bijection, we know that
(f ◦ φ −1 ) −1 [W ] = φ[f −1 [W ] ∩ U]
for any open set W ⊂ R. Thus we conclude that if (f ◦ φ −1 ) −1 [W ] is open for every
open W and every chart φ, then f −1 [W ] is open, and conversely. So f is continuous if
and only if f ◦ φ −1 is continuous.
10. Suppose M is a compact Hausdorff topological space and N is a Hausdorff topological
space. Let f : M → N be a continuous bijection onto a subset of N. Prove that f is a
homeomorphism onto its image.
Solution: Let P = f[M]; then g = f −1 : P → M exists. Since N is Hausdorff, so is
the subset P .
We want to prove that g is continuous. To do this, it is sufficient to show that g−1[K]
is closed in P whenever K is closed in M. Since g −1 [K] = f[K], we just have to show
that f[K] is compact in P for any closed set K in M.
Since M is compact and K is closed in M, we know K is compact. Since f is continuous,
f[K] is a compact set in P . Finally, since P is Hausdorff and f[K] is compact in P , it
must be closed in P .
7