Rational functions - Cs.ioc.ee

More documents

Recommendations

Info

$Algorithmic melody composition based on fractal ... - cs.ioc.ee$

Rational Expansion Theorem Jaan Penjam So, we have S(z) = −13 3(1 − z) + 119 122 + 15(1 + 2z) 5(1 − 3z) . All roots of Q(z) are distinct, so the equation (2) is applicable and the power series S(z) = ∑n0 snzn , where the coefficient sn = − 13 119 + 3 15 (−2)n + 122 5 3n . ✷ 4 Rational Expansion Theorem The method of partial fractions may produce huge systems of equations to be solved. The following theorem gives an alternative technique to mess around the problem. Theorem 4.1. (for Distinct Roots) If R(z) = P(z)/Q(z) the generating function for the sequence 〈rn〉, where Q(z) = (1 − ρ1z)(1 − ρ2z)···(1 − ρℓz) and the numbers (ρ1,...,ρℓ) are distinct, and if P(z) is a polynomial of degree less than ℓ, then rn = a1ρ n 1 + a2ρ n 2 + ··· + aℓρ n ℓ , where ak = −ρkP(1/ρk) Q ′ . (1/ρk) Proof. Construct the sum S(z) = a1 aℓ + ··· + 1 − ρ1z 1 − ρℓz , where the constants ak are as defined in the theorem. We show that T(z)=R(z)-S(z)=0. For that reason, it is sufficient (cf. properties of rational functions, for example in http://en.wikipedia.org/wiki/Rational_function) to confirm that limz→∞ T (z) = 0 and that T (z) is never infinite. Satisfiability of the first condition follows from the observation that both limz→∞ R(z) = 0 and limz→∞ S(z) = 0, because of degrees of numerators is less than degree of corresponding denominators. Only the points where T (z) may approach to infinity are 1/ρk. Hence, to show that limz→αk T (z) = ∞, were αk = 1/ρk, it suffers to prove that lim (z − αk)R(z) = lim (z − αk)S(z). z→αk z→αk Due to ak(z − αk) 1 − ρ jz = ak(z − 1 ρk ) 1 − ρ jz = −ak(1 − ρkz) → 0, if k = j and z → αk. ρk(1 − ρ jz) The right-hand side of this assertion is lim z→αk (z − αk)S(z) = lim (z − αk) z→αk ak(z − αk) 1 − ρkz = −ak ρk = P(1/ρk) Q ′ (1/ρk) The left-hand limit can be transformed due to L’Hospital’s Rule (see in more details on http://mathworld.wolfram.com/LHospitalsRule.html) as follows lim (z − αk)R(z) = lim (z − αk) z→αk z→αk P(z) Q(z) = P(αk) z − αk lim z→αk Q(z) P(αk) = Q ′ P(1/ρk) = (αk) Q ′ (1/ρk) Example 4.1. Consider the fraction S(z) = P1(z) Q(z) from Example 1.1 once more. To use theorem 4.1, we shall compute values P1( 1/ρ) and Q( 1/ρ) for ρ = ρ1,ρ2,ρ3 first. We have P1(z) = z2 − 3z + 28. Hence, 1 P1 = ρ 1 3 1 − 3ρ + 28ρ2 − + 28 = ρ2 ρ ρ2 4
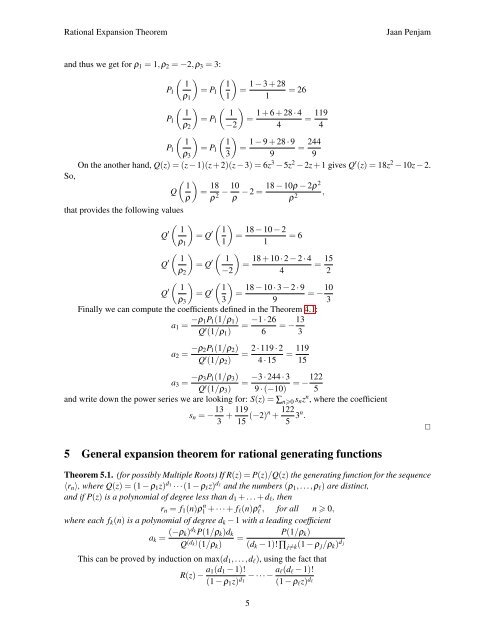
Rational Expansion Theorem Jaan Penjam and thus we get for ρ1 = 1,ρ2 = −2,ρ3 = 3: So, 1 1 P1 = P1 = ρ1 1 1 − 3 + 28 = 26 1 1 1 P1 = P1 = ρ2 −2 1 + 6 + 28 · 4 = 4 119 4 1 1 P1 = P1 = ρ3 3 1 − 9 + 28 · 9 = 9 244 9 On the another hand, Q(z) = (z − 1)(z + 2)(z − 3) = 6z3 − 5z2 − 2z + 1 gives Q ′ (z) = 18z2 − 10z − 2. Q that provides the following values 1 ρ = 18 10 − ρ2 ρ 18 − 10ρ − 2ρ2 − 2 = ρ2 , Q ′ 1 = Q ρ1 ′ 1 = 1 18 − 10 − 2 = 6 1 Q ′ 1 = Q ρ2 ′ 1 = −2 18 + 10 · 2 − 2 · 4 = 4 15 2 Q ′ 1 = Q ρ3 ′ 1 = 3 18 − 10 · 3 − 2 · 9 = − 9 10 3 Finally we can compute the coefficients defined in the Theorem 4.1: a1 = −ρ1P1(1/ρ1) Q ′ −1 · 26 = = −13 (1/ρ1) 6 3 a2 = −ρ2P1(1/ρ2) Q ′ (1/ρ2) = 2 · 119 · 2 4 · 15 = 119 15 a3 = −ρ3P1(1/ρ3) Q ′ −3 · 244 · 3 = = −122 (1/ρ3) 9 · (−10) 5 and write down the power series we are looking for: S(z) = ∑n0 snzn , where the coefficient sn = − 13 119 + 3 15 (−2)n + 122 5 3n . 5 General expansion theorem for rational generating functions Theorem 5.1. (for possibly Multiple Roots) If R(z) = P(z)/Q(z) the generating function for the sequence 〈rn〉, where Q(z) = (1 − ρ1z) d1 ···(1 − ρℓz) dℓ and the numbers (ρ1,...,ρℓ) are distinct, and if P(z) is a polynomial of degree less than d1 + ... + dℓ, then rn = f1(n)ρ n 1 + ··· + fℓ(n)ρ n ℓ , for all n 0, where each fk(n) is a polynomial of degree dk − 1 with a leading coefficient (−ρk) dkP(1/ρk)dk ak = Q (dk) P(1/ρk) = (1/ρk) (dk − 1)!∏j=k(1 − ρ j/ρk) d j This can be proved by induction on max(d1,...,dℓ), using the fact that R(z) − a1(d1 − 1)! (1 − ρ1z) d1 − ··· − aℓ(dℓ − 1)! (1 − ρℓz) dℓ 5 ✷
Page 1 and 2: 1 Motivation ITT9130 Konkreetne Mat
Page 3: Rational Expansion Theorem Jaan Pen
Page 7: Rational Expansion Theorem Jaan Pen

Rational functions - Cs.ioc.ee

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?