Mathematical marvels - CUNY Graduate Center

Expanding interactions
EXPANDERS ARE HIGHLY connected sparse
graphs widely used in computer science, in areas
ranging from parallel computation to complexity
theory and cryptography. There are several ways
of making the intuitive notions of connectivity
and sparsity precise, the simplest and most widely
used is the following. Given a subset of vertices,
its boundary is the set of edges connecting the
set to its complement. The expansion of a subset
is a ratio of the size of a boundary to the size of a
set. The expansion of a graph is a minimum over
all expansion coeffi cients of its subsets.
The expansion coeffi cient captures the notion of
being highly-connected, the bigger the expansion
coeffi cient, the more highly-connected is the
graph. Of course one can simply connect all the
vertices but in this case the number of edges
grows as a square of the number of vertices. The
problem of constructing expanders is nontrivial
because we put the second constraint: the graphs
are to be sparse, ie. the number of edges should
grow linearly with the number of vertices. The
simplest way to accomplish this is to demand
that the graphs be regular, that is, each vertex
has the same number of neighbours (say 3). A
family of regular graphs is said to form a family
of expanders if the expansion coeffi cient of all
the graphs in the family is bounded from below
by some positive constant.
“The expansion coeffi cient is a notion which is
very easy to grasp but it is diffi cult to compute
numerically or to estimate analytically, as the
number of subsets grows exponentially with
the number of vertices,” explains Alex Gamburd,
Professor of Mathematics at the Graduate
Center of the City University of New York and
the University of California, Santa Cruz. “The
starting point of most current work on expanders
is that expansion coeffi cient has a spectral
interpretation: to put it sonorously, if you hit
a graph with a hammer, you can determine
how highly-connected it is by listening to the
bass note. In more
technical terms,
high connectivity
is equivalent to
establishing a
spectral gap for
an averaging (or
Laplace) operator on
the graph”.
CAYLEY GRAPHS
OF GROUPS
Explicit construction
of expanders is
given in terms of
Cayley graphs –
globally-symmetric
graphs defi ned by simple local rules. A Cayley
graph of a group with respect to a fi xed
generating set is a graph whose vertices are
elements of the group; the neighbours of an
element are determined by multiplying it by all
the generators, which is a fi xed small number,
say 3. The simplest example is furnished by the
group of two by two matrices of determinant
one with entries in integers modulo a prime. It
is a consequence of a deep spectral gap result of
Selberg, proved in 1965, that Cayley graphs of
this group with respect to standard generators
are expanders. Here is a simple related example:
take as vertices the integers modulo a prime
and connect them if they differ by plus/minus
one or if their product has remainder 1 when
divided by this prime (in other words, they
are inverses modulo that prime), the resulting
family is a family of expanders. Figure 1
exhibits such graphs for primes 101, 499, 997.
PSEUDO-RANDOMNESS
The crucial feature underlying expansion of
graphs in Figure 1 is pseudo-randomness:
if you take a set of integers from one to
DR ALEX GAMBURD
Research by mathematicians on expander graphs, originating in computer science, turns out to have
unexpected and far-reaching applications to quasi-crystals, quantum computing, and number theory
FIGURE 1. SIMPLE RULES, COMPLEX STRUCTURES
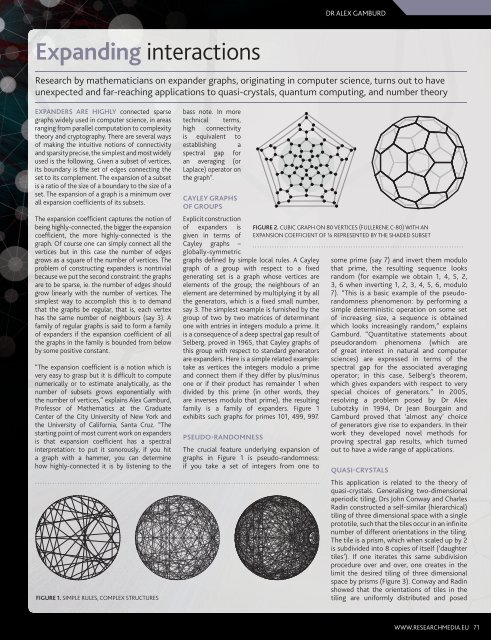
FIGURE 2. CUBIC GRAPH ON 80 VERTICES (FULLERENE C-80) WITH AN
EXPANSION COEFFICIENT OF ¼ REPRESENTED BY THE SHADED SUBSET
some prime (say 7) and invert them modulo
that prime, the resulting sequence looks
random (for example we obtain 1, 4, 5, 2,
3, 6 when inverting 1, 2, 3, 4, 5, 6, modulo
7). “This is a basic example of the pseudorandomness
phenomenon: by performing a
simple deterministic operation on some set
of increasing size, a sequence is obtained
which looks increasingly random,” explains
Gamburd. “Quantitative statements about
pseudorandom phenomena (which are
of great interest in natural and computer
sciences) are expressed in terms of the
spectral gap for the associated averaging
operator; in this case, Selberg’s theorem,
which gives expanders with respect to very
special choices of generators.” In 2005,
resolving a problem posed by Dr Alex
Lubotzky in 1994, Dr Jean Bourgain and
Gamburd proved that ‘almost any’ choice
of generators give rise to expanders. In their
work they developed novel methods for
proving spectral gap results, which turned
out to have a wide range of applications.
QUASI-CRYSTALS
This application is related to the theory of
quasi-crystals. Generalising two-dimensional
aperiodic tiling, Drs John Conway and Charles
Radin constructed a self-similar (hierarchical)
tiling of three dimensional space with a single
prototile, such that the tiles occur in an infi nite
number of different orientations in the tiling.
The tile is a prism, which when scaled up by 2
is subdivided into 8 copies of itself (‘daughter
tiles’). If one iterates this same subdivision
procedure over and over, one creates in the
limit the desired tiling of three dimensional
space by prisms (Figure 3). Conway and Radin
showed that the orientations of tiles in the
tiling are uniformly distributed and posed
WWW.RESEARCHMEDIA.EU 71