Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
<strong>Answers</strong> to <strong>Exercises</strong> 41<br />
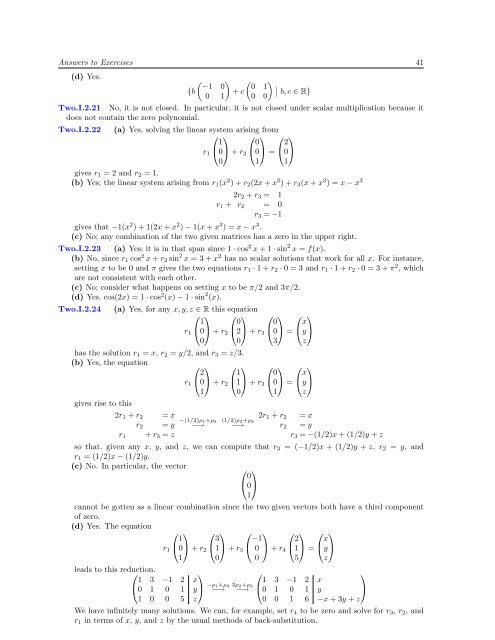
(d) Yes.<br />
{b<br />
( ) ( )<br />
−1 0 0 1 ∣∣<br />
+ c b, c ∈ R}<br />
0 1 0 0<br />
Two.I.2.21 No, it is not closed. In particular, it is not closed under scalar multiplication because it<br />
does not contain the zero polynomial.<br />
Two.I.2.22<br />
(a) Yes, solving the linear system arising from<br />
⎛<br />
r 1<br />
⎝ 1 ⎞ ⎛<br />
0⎠ + r 2<br />
⎝ 0 ⎞ ⎛<br />
0⎠ = ⎝ 2 ⎞<br />
0⎠<br />
0 1 1<br />
gives r 1 = 2 and r 2 = 1.<br />
(b) Yes; the linear system arising from r 1 (x 2 ) + r 2 (2x + x 2 ) + r 3 (x + x 3 ) = x − x 3<br />
2r 2 + r 3 = 1<br />
r 1 + r 2 = 0<br />
r 3 = −1<br />
gives that −1(x 2 ) + 1(2x + x 2 ) − 1(x + x 3 ) = x − x 3 .<br />
(c) No; any combination of the two given matrices has a zero in the upper right.<br />
Two.I.2.23 (a) Yes; it is in that span since 1 · cos 2 x + 1 · sin 2 x = f(x).<br />
(b) No, since r 1 cos 2 x + r 2 sin 2 x = 3 + x 2 has no scalar solutions that work for all x. For instance,<br />
setting x to be 0 and π gives the two equations r 1 · 1 + r 2 · 0 = 3 and r 1 · 1 + r 2 · 0 = 3 + π 2 , which<br />
are not consistent with each other.<br />
(c) No; consider what happens on setting x to be π/2 and 3π/2.<br />
(d) Yes, cos(2x) = 1 · cos 2 (x) − 1 · sin 2 (x).<br />
Two.I.2.24<br />
(a) Yes, for any x, y, z ∈ R this equation<br />
⎛<br />
r 1<br />
⎝ 1 ⎞ ⎛<br />
0⎠ + r 2<br />
⎝ 0 ⎞ ⎛<br />
2⎠ + r 3<br />
⎝ 0 ⎞ ⎛<br />
0⎠ = ⎝ x ⎞<br />
y⎠<br />
0 0 3 z<br />
has the solution r 1 = x, r 2 = y/2, and r 3 = z/3.<br />
(b) Yes, the equation<br />
⎛<br />
r 1<br />
⎝ 2 ⎞ ⎛<br />
0⎠ + r 2<br />
⎝ 1 ⎞ ⎛<br />
1⎠ + r 3<br />
⎝ 0 ⎞ ⎛<br />
0⎠ = ⎝ x ⎞<br />
y⎠<br />
1 0 1 z<br />
gives rise to this<br />
2r 1 + r 2<br />
r 1<br />
r 2<br />
= x<br />
= y<br />
+ r 3 = z<br />
−(1/2)ρ 1+ρ 3<br />
−→<br />
(1/2)ρ 2+ρ 3<br />
−→ r 2 = y<br />
2r 1 + r 2 = x<br />
r 3 = −(1/2)x + (1/2)y + z<br />
so that, given any x, y, and z, we can compute that r 3 = (−1/2)x + (1/2)y + z, r 2 = y, and<br />
r 1 = (1/2)x − (1/2)y.<br />
(c) No. In particular, the vector<br />
⎛ ⎞<br />
0<br />
⎝0⎠<br />
1<br />
cannot be gotten as a linear combination since the two given vectors both have a third component<br />
of zero.<br />
(d) Yes. The equation<br />
⎛<br />
r 1<br />
⎝ 1 ⎞ ⎛<br />
0⎠ + r 2<br />
⎝ 3 ⎞ ⎛<br />
1⎠ + r 3<br />
⎝ −1<br />
⎞ ⎛<br />
0 ⎠ + r 4<br />
⎝ 2 ⎞ ⎛<br />
1⎠ = ⎝ x ⎞<br />
y⎠<br />
1 0 0 5 z<br />
leads to this reduction.<br />
⎛<br />
⎝ 1 3 −1 2 x<br />
⎞<br />
0 1 0 1 y<br />
1 0 0 5 z<br />
⎠ −ρ1+ρ3<br />
−→<br />
3ρ2+ρ3<br />
−→<br />
⎛<br />
⎝ 1 3 −1 2 x<br />
0 1 0 1 y<br />
0 0 1 6 −x + 3y + z<br />
We have infinitely many solutions. We can, for example, set r 4 to be zero and solve for r 3 , r 2 , and<br />
r 1 in terms of x, y, and z by the usual methods of back-substitution.<br />
⎞<br />
⎠