Hochschild Cohomology and Representation-finite Algebras Ragnar ...

17
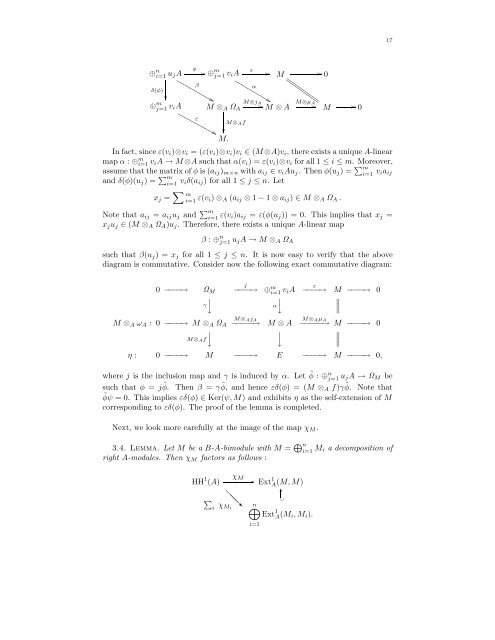
⊕ n i=1 u jA φ ⊕ m j=1 v i A ε M
0
β
α
δ(φ)
⊕ m j=1 v M⊗j A
iA M ⊗ A Ω A
M ⊗ A M⊗µA M 0
ε
M⊗ Af
M.
In fact, since ε(v i )⊗v i =(ε(v i )⊗v i )v i ∈ (M ⊗A)v i , there exists a unique A-linear
map α : ⊕ m i=1 v iA → M ⊗A such that α(v i )=ε(v i )⊗v i for all 1 ≤ i ≤ m. Moreover,
assume that the matrix of φ is (a ij ) m×n with a ij ∈ v i Au j .Thenφ(u j )= ∑ m
i=1 v ia ij
and δ(φ)(u j )= ∑ m
i=1 v iδ(a ij ) for all 1 ≤ j ≤ n. Let
x j = ∑ m
i=1 ε(v i) ⊗ A (a ij ⊗ 1 − 1 ⊗ a ij ) ∈ M ⊗ A Ω A .
Note that a ij = a ij u j and ∑ m
i=1 ε(v i)a ij = ε(φ(u j )) = 0. This implies that x j =
x j u j ∈ (M ⊗ A Ω A )u j . Therefore, there exists a unique A-linear map
β : ⊕ n j=1 u jA → M ⊗ A Ω A
such that β(u j )=x j for all 1 ≤ j ≤ n. It is now easy to verify that the above
diagram is commutative. Consider now the following exact commutative diagram:
j
0 −−−−→ Ω M −−−−→
⏐
γ↓
⊕ m i=1 v iA
⏐
α↓
ε
−−−−→ M −−−−→ 0
∥
M ⊗ A ω A : 0 −−−−→ M ⊗ A Ω A
⏐
M⊗ Af↓
M⊗ Aj A
M⊗ Aµ A
−−−−−→ M ⊗ A −−−−−→ M −−−−→ 0
⏐
↓
∥
η : 0 −−−−→ M −−−−→ E −−−−→ M −−−−→ 0,
where j is the inclusion map and γ is induced by α. Let ˜φ : ⊕ n j=1 u jA → Ω M be
such that φ = j ˜φ. Then β = γ ˜φ, and hence εδ(φ) =(M ⊗ A f)γ ˜φ. Note that
˜φψ = 0. This implies εδ(φ) ∈ Ker(ψ, M) and exhibits η as the self-extension of M
corresponding to εδ(φ). The proof of the lemma is completed.
Next, we look more carefully at the image of the map χ M .
3.4. Lemma. Let M be a B-A-bimodule with M = ⊕ n
i=1 M i a decomposition of
right A-modules. Then χ M factors as follows :
HH 1 χ M
(A) ✲ Ext
1
A (M,M)
❅ ✻
∑ ❅❅
i χ ∪
M i
❘ n⊕
Ext 1 A(M i ,M i ).
i=1