Solutions to Final Examination

EE32a Signals, Systems, and Transforms
December 15, 2001
Final Examination — Solutions
R. J. McEliece
162 Moore
Problem 1. (Graded by Muhammed) The first step is to decompose X(s) into partial
fractions:
s − 1
(s + 2)(s + 3)(s 2 + s +1) = −1
s +2 + 4/7 (3s +1)/7
+
s +3 s 2 + s +1
= X 1 (s)+X 2 (s)+X 3 (s).
Note that the poles of X(s) are at s = −2, −3, and −1/2 ± j √ 3/2.
The inverse Laplace transforms of X 1 (s), X 2 (s), and X 3 (s) are
{
x
R
x 1 (t) = 1 (t) =−e −2t u(t) Re(s) > −2
x L 1 (t) =e −2t u(−t) Re(s) < −2
{
x
R
x 2 (t) = 2 (t) =(4/7)e −3t u(t) Re(s) > −3
x L 2 (t) =−(4/7)e −3t u(−t) Re(s) > −3
(
x
R
x 3 (t) ={
3 (t) = 1 7 3e −t/2 cos( √ 3t/2) − (1/ √ 3)e −t/2 sin( √ 3t/2) ) u(t) Re(s) > −1/2
(
x L 3 (t) =− 1 7 3e −t/2 cos( √ 3t/2) − (1/ √ 3)e −t/2 sin( √ 3t/2) ) u(−t) Re(s) < −1/2
.
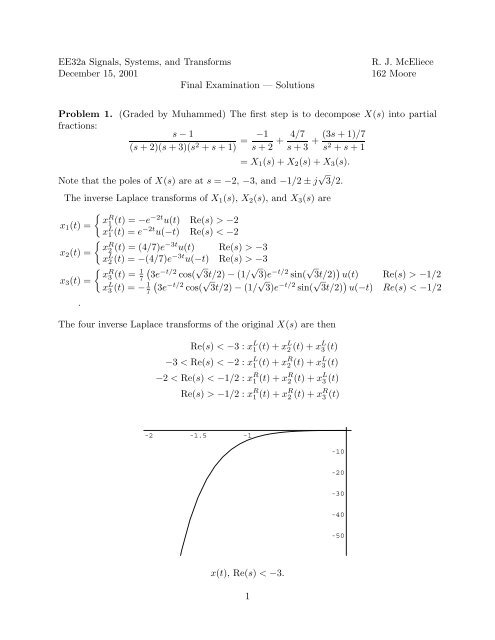
The four inverse Laplace transforms of the original X(s) are then
Re(s) < −3 :x L 1 (t)+x L 2 (t)+x L 3 (t)
−3 < Re(s) < −2 :x L 1 (t)+x R 2 (t)+x L 3 (t)
−2 < Re(s) < −1/2 :x R 1 (t)+x R 2 (t)+x L 3 (t)
Re(s) > −1/2 :x R 1 (t)+x R 2 (t)+x R 3 (t)
-2 -1.5 -1
-10
-20
-30
-40
-50
x(t), Re(s) < −3.
1

6
5
4
3
2
1
-1 -0.5 0.5 1
x(t), −3 < Re(s) < −2.
2
-8 -6 -4 2
-2
-4
-6
x(t), −2 < Re(s) < −1/2.
2

0.04
0.02
-0.02
2 4 6 8 10
-0.04
-0.06
-0.08
x(t), Re(s) > −1/2.
3

Problem 2. (Graded by all.)
(a) Use the fact that Re x(t) =(x(t)+x ∗ (t))/2, and entry 4.3.3 in Table 4.1, p. 328:
Re x(t) ←→ F X(jΩ) + X∗ (−jΩ)
.
2
(b) Using entries 4.3.3 and 4.3.5 in Table 4.1 :
x ∗ (−t) F
←→X ∗ (jΩ).
(c) Using the given decomposition and entry 4.3.5 in Table 4.1 :
Ev x(t) ←→ F X(jΩ) + X(−jΩ)
.
2
4

Problem 3. (Graded by RJM) The key to this problem is to use z-transforms. Indeed, if
g[n] ∗ g[n] =h[n], we have
G(z) 2 = H(z).
Thus if g[n] isaconvolution square root of h[n], we have
G(z) =± √ H(z).
(a) Here H(z) =1+2z −1 + z −2 =(1+z −1 ) 2 ,soG(z) =±(1 + z −1 ) 2 . Ignoring the
possible minus sign, we have
{ 1 if n =0orn =1
g[n] =
0 otherwise.
(b) Here we have
H(z) = ∑ n≥0(n +1)z −n =
1
(1 − z −1 ) 2 .
Thus G(z) =1/(1 − z −1 ), so that (again ignoring the possible minus sign)
h[n] =u[n].
(c) Here H(z) = ∑ n≥0 z−1 =1/(1 − z −1 ), so that G(z) =(1− z −1 ) −1/2 . But as we
discussed in class on Nov. 9, we can apply the binomial theorem, even when the exponent
is negative, so that
g(z) =(1− z −1 ) −1/2 = ∑ ( ) −1/2
(−1) n z −n .
n
n≥0
Thus
( ) −1/2
g[n] =(−1) n u[n].
n
This expression can be simplified considerably.:
(−1) n ( −1/2
n
Thus g[0] = 1, and for n ≥ 1,
)
=(−1) n · (− 1 2 )(− 3 2n−1
2
) ···(−
2
)
1 · 2 ···n
=( 1 2 ) · (3 4 ) ···(2n − 1
2n ).
n∏
(
g[n] = 1 − 1 )
.
2k
k=1
For example g[1] = 1/2, g[2] = 3/8, g[3] = 5/16, etc.
Notess: A few studeents used Mathematica to find the inverse z-transform of G(z), and
obtained the remarkable expression
Γ(n +1/2)
g[n] =
Γ(n +1) √ π .
I reluctantly accepted this formula. Also, some students found a recursive method for
computing the sequence of g[n]’s. I took off 2.5 points for this approach.
5

Problem 4. (Graded by Donald) This system in the serial iterconnection of two simpler
systems, with systems functions (obtained by “inspection,” perhaps)
H 1 (D) = 1 − 1 2 D
1 − 1 3 D
H 2 (D) =
1
4 + D
1+ 1 2 D ,
where I have substituted the delay operator D for the cumbersome z −1 . Thsu the overall
system function is
1
4
H(D) =H 1 (D)H 2 (D) =
+ 7 8 D − 1 2 D2
1+ 1 6 D − 1 .
6 D2
from this we can read off the difference equation. It is
(a) y[n]+ 1 6 y[n − 1] − 1 6 y[n − 2] = 1 4 x[n]+7 8 x[n − 1] − 1 x[n − 2].
2
Of course the frequency responce is just the systems function evaluated at z = e jΩ :
(b) H(e jΩ )=
1
4 + 7 8 e−jΩ − 1 2 e−2jΩ
1+ 1 6 e−jΩ − 1 6 e−2jΩ .
Finally, to obtain the impulse response, we first decompose the expression for H(z) into
partial fractions:
13
21
20
H(D) =3−
1 − 1 3 D − 10
1+ 1 2 D .
The impulse response h[n] isjust the coefficient of D n in the expansion of H(D) asapower
series in D, and so:
(c) h[n] =3δ[n] − 13
20 (1 3 )n − 21
10 (−1 2 )n ,
for n ≥ 0.
6

Problem 5. (Graded by Cedric)
(a) The easy way to do this problem is to observe that “the triangle is the convolution
of the box with itself,” i.e.,
x(t) =y(t) ∗ y(t),
where
y(t) =
{
1 if |t| ≤1/2
0 if |t| > 1/2.
Using the result of Example 4.4 (or Table 4.2), we have
Y (jω)=2
sin ω/2
,
ω
so that
X(jω)=Y (jω) 2 =4 sin2 ω/2
ω 2 .
(b)
1
-5 -4 -3 -2 --1 1 2 3 4
Sketch of x(t) ∗ ∑ +∞
k=−∞
δ(t − 4k).
(c) Here are two such g(t)’s (there are infinitely many more):
7

1
-5 -4 -3 -2 --1 0 1 2 3 4
1
-5 -4 -3 -2 --1 0 1 2 3 4
8