David Zureick-Brown - Rational points and algebraic cycles
David Zureick-Brown - Rational points and algebraic cycles
David Zureick-Brown - Rational points and algebraic cycles
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
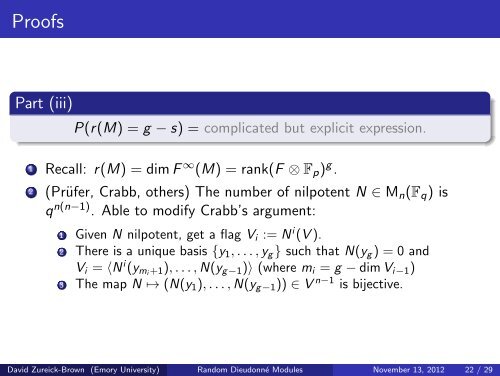
Proofs<br />
Part (iii)<br />
P(r(M) = g − s) = complicated but explicit expression.<br />
1 Recall: r(M) = dim F ∞ (M) = rank(F ⊗ F p ) g .<br />
2 (Prüfer, Crabb, others) The number of nilpotent N ∈ M n (F q ) is<br />
q n(n−1) . Able to modify Crabb’s argument:<br />
1 Given N nilpotent, get a flag V i := N i (V ).<br />
2 There is a unique basis {y 1 , . . . , y g } such that N(y g ) = 0 <strong>and</strong><br />
V i = 〈N i (y mi +1), . . . , N(y g−1 )〉 (where m i = g − dim V i−1 )<br />
3 The map N ↦→ (N(y 1 ), . . . , N(y g−1 )) ∈ V n−1 is bijective.<br />
<strong>David</strong> <strong>Zureick</strong>-<strong>Brown</strong> (Emory University) R<strong>and</strong>om Dieudonné Modules November 13, 2012 22 / 29