Vol. 10 No 6 - Pi Mu Epsilon

486 PI MU EPSILON JOURNAL
SEARCHING FOR INFINITE FAMILIES OF 2-TRANSITIVE SPACES 487
Example 4. 2 Similarity:
f(X) = 2X + 2(mod 5)
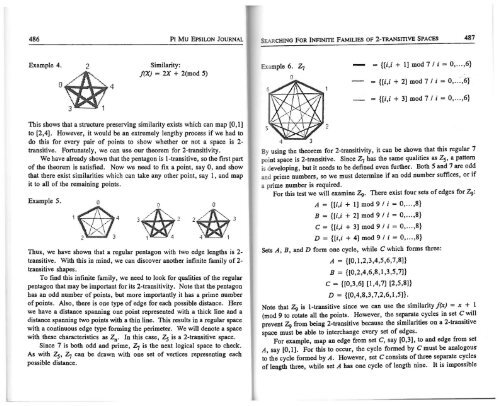
Example 6. Z,
(I
- = {[i,i + 1] mod 7 I i = 0, ... ,6}
= {[i,i + 2] mod 7 I i = 0, .. :,6}
= {[i,i + 3] mod 7 I i = 0, ... ,6}
This shows that a structure preserving similarity exists which can map [0,1]
to [2,4]. However, it would be an extremely lengthy process if we bad to
do this for every pair of points to show whether or not a space is 2-
transitive. Fortunately, we can use our theorem for 2-transitivity.
We have already shown that the pentagon is !-transitive, so the first part
of the theorem is satisfied. Now we need to fix a point, say 0, and show
that there exist similarities which can take any other point, say 1, and map
it to all of the remaining points.
Example 5. 0
0
@
- .-)
3 ~
I
1 4
Thus, we have shown that a regular pentagon with two edge lengths is 2-
transitive. With this in mind, we can discover another infinite family of 2-
transitive shapes.
To find this infinite family, we need to look for qualities of the regular
pentagon that may be important for its 2-transitivity. Note that the pentagon
has an odd number of points, but more importantly it has a prime number
of points. Also, there is one type of edge for each possible distance. Here
we have a distance spanning one point represented with a thick line and a
distance spanning two points with a thin line. This results in a regular space
with a continuous edge type forming the perimeter. We will denote a space
with these characteristics as Z 0
• In this case, z 5 is a 2-transitive space.
Since 7 is both odd and prime, Z, is the next logical space to check.
As with z 5 , Z, can be drawn with one set of vertices representing each
possible distance.
4
2
By using the theorem for 2-transitivity, it can be shown that this regular 7
point space is 2-transitive. Since Z, has the same qualities as Zs, a pattern
is developing, but it needs to be defined even further. Both 5 and 7 are odd
and prime numbers, so we must determine if an odd number suffices, or if
a prime number is required.
For this test we will examine ~·
A = {[i,i + 1] mod 9 I i = 0, ... ,8}
B = {[i,i + 2] mod 9 I i = 0, .. . ,8}
C = {[i,i + 3] mod 9 I i = 0 , .. . ,8}
D = {[i,i + 4] mod 9 I i = 0, ... ,8}
There exist four sets of edges for~:
Sets A, B, and D form one cycle, while C which forms three:
A = {[0,1,2,3,4,5,6,7,81}
B = {[0,2,4,6,8,1,3,5,7]}
c = {[0,3,6] [1 ,4,7] [2,5,81}
D = {[0,4,8,3,7,2,6,1,5]}.
Note that ~ is !-transitive since we can use the similarity/(~) = x +. 1
(mod 9 to rotate all the points. However, the separate cycles m set C w11l
prevent ~ from being 2-transitive because the similarities on a 2-transitive
space must be able to interchange every set of edges.
For example, map an edge from set C, say [0,3], to and edge from se~
A, say [0,1]. For this to occur, the cycle formed by C must be analogous
to the cycle formed by A. However, set C consists of three separate cycles
of length three, while set A has one cycle of length nine. It is impossible