1 Introduction 2 Resolvents and Green's Functions

z
.
. . . . . . .
q 4
C 4
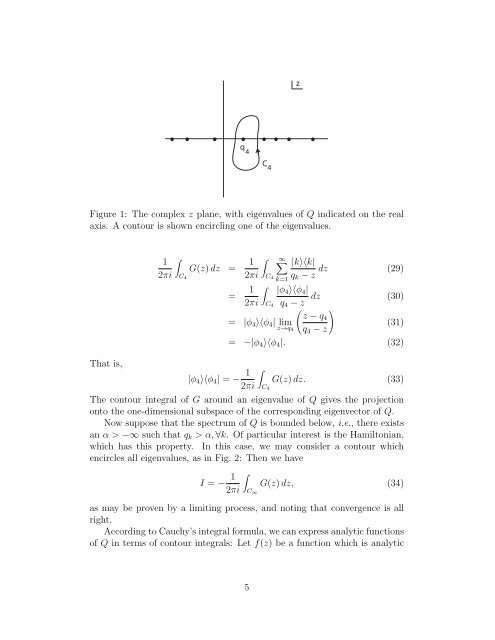
Figure 1: The complex z plane, with eigenvalues of Q indicated on the real
axis. A contour is shown encircling one of the eigenvalues.
That is,
∫
1
G(z) dz = 1 ∫
2πi C 4 2πi C 4
∫
= 1
2πi
∞ ∑
k=1
C 4
|φ 4 〉〈φ 4 |
q 4 − z
|k〉〈k|
dz (29)
q k − z
dz (30)
=
( z − q4
|φ 4 〉〈φ 4 | lim z→q4 q 4 − z
(31)
= −|φ 4 〉〈φ 4 |. (32)
|φ 4 〉〈φ 4 | = − 1 ∫
G(z) dz. (33)
2πi C 4
The contour integral of G around an eigenvalue of Q gives the projection
onto the one-dimensional subspace of the corresponding eigenvector of Q.
Now suppose that the spectrum of Q is bounded below, i.e., there exists
an α > −∞ such that q k > α, ∀k. Of particular interest is the Hamiltonian,
which has this property. In this case, we may consider a contour which
encircles all eigenvalues, as in Fig. 2: Then we have
I = − 1 ∫
G(z) dz, (34)
2πi C ∞
as may be proven by a limiting process, and noting that convergence is all
right.
According to Cauchy’s integral formula, we can express analytic functions
of Q in terms of contour integrals: Let f(z) be a function which is analytic
)
5