MIT Integration Bee 2009 Worked Solutions - TweetCube
MIT Integration Bee 2009 Worked Solutions - TweetCube
MIT Integration Bee 2009 Worked Solutions - TweetCube
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
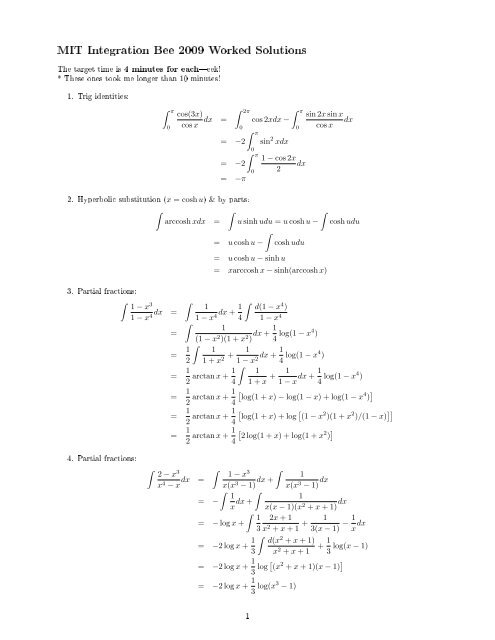
<strong>MIT</strong> <strong>Integration</strong> <strong>Bee</strong> <strong>2009</strong> <strong>Worked</strong> <strong>Solutions</strong><br />
The target time is 4 minutes for eacheek!<br />
* These ones took me longer than 10 minutes!<br />
1. Trig identities:<br />
ˆ π<br />
0<br />
ˆ<br />
cos(3x)<br />
2π<br />
cos x dx =<br />
0<br />
= −2<br />
= −2<br />
= −π<br />
ˆ π<br />
cos 2xdx −<br />
ˆ π<br />
0<br />
ˆ π<br />
0<br />
sin 2 xdx<br />
0<br />
1 − cos 2x<br />
dx<br />
2<br />
sin 2x sin x<br />
dx<br />
cos x<br />
2. Hyperbolic substitution (x = cosh u) & by parts:<br />
ˆ<br />
ˆ<br />
ˆ<br />
arccosh xdx = u sinh udu = u cosh u −<br />
ˆ<br />
= u cosh u − cosh udu<br />
cosh udu<br />
= u cosh u − sinh u<br />
= xarccosh x − sinh(arccosh x)<br />
3. Partial fractions:<br />
ˆ 1 − x<br />
3<br />
ˆ<br />
1 − x 4 dx = 1<br />
1 − x 4 dx + 1 ˆ d(1 − x 4 )<br />
4 1 − x<br />
ˆ<br />
4<br />
1<br />
=<br />
(1 − x 2 )(1 + x 2 ) dx + 1 4 log(1 − x4 )<br />
= 1 ˆ<br />
1<br />
2 1 + x 2 + 1<br />
1 − x 2 dx + 1 4 log(1 − x4 )<br />
= 1 2 arctan x + 1 ˆ<br />
1<br />
4 1 + x + 1<br />
1 − x dx + 1 4 log(1 − x4 )<br />
= 1 2 arctan x + 1 [<br />
log(1 + x) − log(1 − x) + log(1 − x 4 ) ]<br />
4<br />
= 1 2 arctan x + 1 [ [<br />
log(1 + x) + log (1 − x 2 )(1 + x 2 )/(1 − x) ]]<br />
4<br />
= 1 2 arctan x + 1 [<br />
2 log(1 + x) + log(1 + x 2 ) ]<br />
4<br />
4. Partial fractions:<br />
ˆ 2 − x<br />
3<br />
x 4 − x dx = ˆ<br />
= −<br />
1 − x 3 ˆ<br />
x(x 3 − 1) dx + ˆ ˆ 1<br />
x dx + ˆ 1<br />
= − log x +<br />
= −2 log x + 1 3<br />
1<br />
x(x 3 − 1) dx<br />
1<br />
x(x − 1)(x 2 + x + 1) dx<br />
2x + 1<br />
3 x 2 + x + 1 + 1<br />
3(x − 1) − 1 x dx<br />
ˆ d(x 2 + x + 1)<br />
x 2 + x + 1 + 1 log(x − 1)<br />
3<br />
= −2 log x + 1 3 log [ (x 2 + x + 1)(x − 1) ]<br />
= −2 log x + 1 3 log(x3 − 1)<br />
1
5. * Substitution of t = π − x (dt = −dx) which leads to sin x = cos t so arccos(sin x) = t (I realised it<br />
2<br />
was this simple only after my rst thought, wanting to substitute sin x = cos t and then doing all those<br />
motions, which worked ne except for an ambiguity in sign and an awkward limit):<br />
ˆ 1<br />
0<br />
arccos(sin x)dx = −<br />
=<br />
ˆ π/2−1<br />
π/2<br />
ˆ π/2<br />
π/2−1<br />
] π/2<br />
tdt<br />
[ t<br />
2<br />
=<br />
2<br />
π/2−1<br />
= (π − 1)/2<br />
This one is deceptively simple. I was sure it was wrong until I did a numerical integration to check!<br />
tdt<br />
6. Trig identities:<br />
ˆ π/2<br />
−π/2<br />
√<br />
1 − cos xdx = 2<br />
ˆ π/2<br />
= 2 √ 2<br />
= −4 √ 2<br />
0<br />
ˆ π/2<br />
0<br />
= 4( √ 2 − 1)<br />
√<br />
1 − (1 − 2 sin 2 x 2 )dx<br />
( x<br />
)<br />
sin dx<br />
2<br />
[ ( x<br />
)] π/2<br />
cos<br />
2<br />
0<br />
7. * Substitution (y = 7 − x) & trickery (someone likes the number 7 in this question, even the limits<br />
3 + 4 = 7!):<br />
I =<br />
ˆ 4<br />
3<br />
x 1/7<br />
ˆ 3<br />
(7 − x) 1/7 + x dx = − (7 − y) 1/7<br />
dy<br />
1/7 y 1/7 + (7 − y)<br />
1/7<br />
=<br />
=<br />
4<br />
ˆ 4<br />
3<br />
ˆ 4<br />
3<br />
= 1 − I<br />
⇐⇒ I = 1/2<br />
(7 − y) 1/7 + y 1/7 − y 1/7<br />
(7 − y) 1/7 + y 1/7 dy<br />
1 −<br />
y 1/7<br />
dy<br />
(7 − y) 1/7 + y1/7 [My thanks to Mark for that! I didn't spot the add/subtract trick and was baed.]<br />
8. Trig substitution (x = 2 sin u) & identities:<br />
ˆ 2<br />
1<br />
√<br />
4 − x2 dx = 2<br />
ˆ π/2<br />
= 4<br />
= 2<br />
π/6<br />
ˆ π/2<br />
π/6<br />
ˆ π/2<br />
π/6<br />
√<br />
4 − 4 sin 2 u cos udu<br />
cos 2 udu<br />
cos 2u + 1du<br />
= [sin 2u + 2] π/2<br />
π/6<br />
= 2π 3 − √<br />
3<br />
2<br />
2
9. * Hyperbolic substitution (x = sinh u, then w = 1/ sinh u, then z = w 2 + 1; i.e. z = coth 2 u) &<br />
identities:<br />
ˆ<br />
ˆ<br />
1<br />
x 2√ x 2 + 1 dx = cosh u<br />
sinh 2 u<br />
√sinh 2 u + 1 du<br />
ˆ<br />
1<br />
=<br />
sinh 2 u du<br />
ˆ<br />
−w<br />
= √<br />
w2 + 1 dw<br />
N.B. If you recall ´<br />
= − 1 2<br />
ˆ<br />
= − √ z<br />
= − coth u<br />
1<br />
√ z<br />
dz<br />
= − coth(arcsinh x)<br />
1<br />
sinh 2 u du = ´ cosech 2 u du = − coth u then you're done easily. Unlike me :(<br />
10. Trig identities, hyperbolics & substitution (u = cot x, then u = sinh t):<br />
ˆ<br />
ˆ<br />
2 csc 3 xdx = csc x csc 2 xdx<br />
=<br />
ˆ<br />
− csc x d cot x<br />
dx<br />
dx<br />
ˆ √<br />
= − 1 + cot 2 xd cot x<br />
ˆ √1<br />
= − + u2 du<br />
ˆ<br />
= − cosh 2 t dt<br />
= − 1 ˆ<br />
cosh 2t + 1 dt<br />
2<br />
= − (sinh 2t + 2t) /4<br />
= − (sinh t cosh t + t) /2<br />
[<br />
= − u √ ]<br />
1 + u 2 + arcsinh u /2<br />
= − [cot x csc x + arcsinh(cot x)] /2<br />
* This took under 10 minutes to get the idea out, but it took nearly 20 minutes for me to convince<br />
myself it is right! Not a terribly pleasant solution.<br />
11. Trig substitution (x = sin θ, then u = θ + π/4) & identities:<br />
ˆ 2<br />
0<br />
ˆ<br />
4<br />
0<br />
x + √ 4 − x dx = 4 2<br />
= 4<br />
π/2<br />
ˆ π/2<br />
0<br />
= 4 √<br />
2<br />
ˆ π/2<br />
= 2 √ 2<br />
= 2 √ 2<br />
−2 sin θ<br />
2 cos θ + 2 sin θ dθ<br />
sin θ<br />
cos θ + sin θ dθ<br />
0<br />
ˆ 3π/4<br />
π/4<br />
ˆ 3π/4<br />
π/4<br />
sin θ<br />
sin(θ + π/4) dθ<br />
sin(u − π/4)<br />
du<br />
sin u<br />
( π<br />
)<br />
cos − sin<br />
4<br />
= 2 [u − log(sin u)] 3π/4<br />
π/4<br />
= π<br />
( π<br />
4<br />
)<br />
cot u du<br />
3
12. By parts (twice):<br />
ˆ<br />
I =<br />
ˆ<br />
e −x cos xdx = −e −x cos x − e −x sin xdx<br />
ˆ<br />
= −e −x cos x + e −x sin x − e −x cos xdx<br />
= e −x (sin x − cos x) − I<br />
⇐⇒ I = e −x (sin x − cos x)/2<br />
4