Exterior Angle Inequality
Exterior Angle Inequality
Exterior Angle Inequality
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Department of Applied Mathematics ISU Geometry Spring 2008 1<br />
Lecture 7 – Lecture note by Jerry Yeh from Textbook Section 3.4. – April 8, 2008<br />
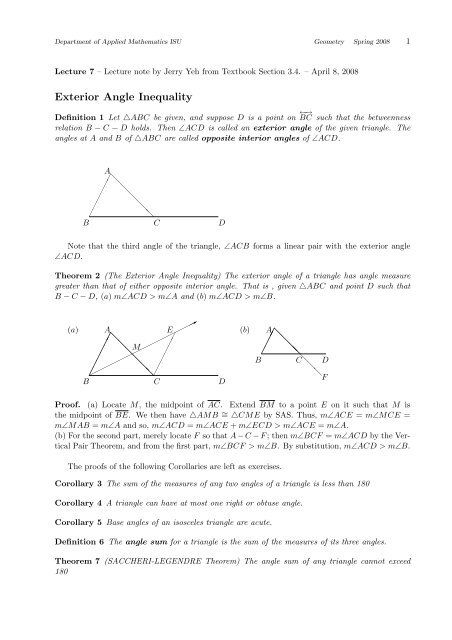
<strong>Exterior</strong> <strong>Angle</strong> <strong>Inequality</strong><br />
Definition 1 Let △ABC be given, and suppose D is a point on ←→ BC such that the betweenness<br />
relation B − C − D holds. Then ∠ACD is called an exterior angle of the given triangle. The<br />
angles at A and B of △ABC are called opposite interior angles of ∠ACD.<br />
A<br />
✁ ✁✁❅ ❅❅<br />
❅❅❅<br />
✁ ✁✁<br />
B<br />
C<br />
D<br />
Note that the third angle of the triangle, ∠ACB forms a linear pair with the exterior angle<br />
∠ACD.<br />
Theorem 2 (The <strong>Exterior</strong> <strong>Angle</strong> <strong>Inequality</strong>) The exterior angle of a triangle has angle measure<br />
greater than that of either opposite interior angle. That is , given △ABC and point D such that<br />
B − C − D, (a) m∠ACD > m∠A and (b) m∠ACD > m∠B.<br />
(a)<br />
✟✯<br />
A<br />
E<br />
❅ M ❅❅❅❅<br />
✁ ✁✁✁✁✁❅ ✟<br />
✟<br />
✟✟✟✟✟✟✟✟✟✟✟<br />
✁ ✁✁✁✁✁<br />
B<br />
C<br />
D<br />
(b)<br />
A<br />
✁ ✁✁✁❅ ❅<br />
❅❅<br />
B C❅ D ❅❅<br />
F<br />
Proof. (a) Locate M, the midpoint of AC. Extend BM to a point E on it such that M is<br />
the midpoint of BE. We then have △AMB ∼ = △CME by SAS. Thus, m∠ACE = m∠MCE =<br />
m∠MAB = m∠A and so, m∠ACD = m∠ACE + m∠ECD > m∠ACE = m∠A.<br />
(b) For the second part, merely locate F so that A − C − F ; then m∠BCF = m∠ACD by the Vertical<br />
Pair Theorem, and from the first part, m∠BCF > m∠B. By substitution, m∠ACD > m∠B.<br />
The proofs of the following Corollaries are left as exercises.<br />
Corollary 3 The sum of the measures of any two angles of a triangle is less than 180<br />
Corollary 4 A triangle can have at most one right or obtuse angle.<br />
Corollary 5 Base angles of an isosceles triangle are acute.<br />
Definition 6 The angle sum for a triangle is the sum of the measures of its three angles.<br />
Theorem 7 (SACCHERI-LEGENDRE Theorem) The angle sum of any triangle cannot exceed<br />
180
Department of Applied Mathematics ISU Geometry Spring 2008 2<br />
Proof. Begin with △ABC and the construction used in the proof of the exterior <strong>Angle</strong> <strong>Inequality</strong> :<br />
Find the midpoint M of AC and find E so that B−M −E and BM = ME; connect C and E to form<br />
△BEC. As in the proof of Theorem reftheorem:EXTERIOR, SAS gives us △AMB ∼ = △CME<br />
and hence<br />
m∠ABE = m∠BEC and m∠BAC = m∠ACE<br />
Thus, in the triangle △BCE,<br />
m∠EBC + m∠BCE + m∠BEC = m∠EBC + m∠BCA + m∠ACE + m∠ABE<br />
= m∠EBC + m∠BCA + m∠BAC + m∠ABE<br />
= m∠ABC + m∠BCA + m∠BAC<br />
That is, the angle sum of △ABC is equal to the angle sum of △BCE.<br />
We can then repeat the construction on △BEC : locate the midpoint N of CE and extend BN<br />
its own length to point F such that B −N −F . Continue this process. Here is what happens. First,<br />
the angle sums of all the new triangles we constructed in the process (△BCE, △BCF, △BCG, · · · )<br />
remain constant. Also, the construction allows us to use SAS to show that m∠E = ∠ABE, m∠F =<br />
m∠EBF, m∠G = m∠F BG · · · . We will call these angle measures θ 1 , θ 2 , θ 3 , · · · .<br />
Now, let’s suppose that, contrary to what we are trying to prove, the angle sum of △ABC is<br />
greater than 180. Hence there is some positive constant t such that<br />
m∠A + m∠ABC + m∠BCA = 180 + t<br />
Then all the other triangles we constructed will also have angle sum = 180 + t. Now, observe<br />
that<br />
θ 1 < m∠ABC, θ 1 + θ 2 < m∠ABC, θ 1 + θ 2 + θ 3 < m∠ABC.<br />
In fact, for any n,<br />
θ 1 + θ 2 + θ 3 + · · · + θ n < m∠ABC.<br />
This means ultimately that for at least one n taken large enough, θ n < t. (for if this were not true,<br />
then θ n ≥ t for any n, we would have<br />
m∠ABC > θ 1 + θ 2 + θ 3 + · · · + θ n ≥ nt for any n.<br />
This is impossible.)<br />
So suppose, for convenience, that the triangle for which this occurs is labeled BW C and that<br />
m∠W < t. The angle sum of △BW C = 180 + t, so it follows that<br />
180 + t = m∠W BC + m∠BCW + m∠W < m∠W BC + m∠BCW + t<br />
or, canceling t from both sides,<br />
a contradiction to Corollary 3.<br />
180 < m∠W BC + m∠BCW,