Differential Equations on the two dimensional torus 1 Constant ...

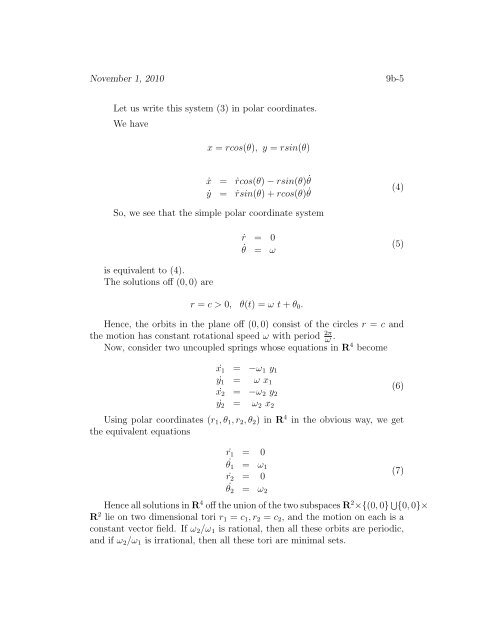
November 1, 2010 9b-5Let us write this system (3) in polar coordinates.We havex = rcos(θ), y = rsin(θ)ẋ = ṙcos(θ) − rsin(θ) ˙θẏ = ṙsin(θ) + rcos(θ) ˙θ(4)So, we see that the simple polar coordinate systemṙ = 0˙θ = ω(5)is equivalent to (4).The solutions off (0, 0) arer = c > 0, θ(t) = ω t + θ 0 .Hence, the orbits in the plane off (0, 0) consist of the circles r = c andthe motion has constant rotational speed ω with period 2π ω .Now, consider two uncoupled springs whose equations in R 4 becomex˙1 = −ω 1 y 1y˙1 = ω x 1(6)x˙2 = −ω 2 y 2y˙2 = ω 2 x 2Using polar coordinates (r 1 , θ 1 , r 2 , θ 2 ) in R 4 in the obvious way, we getthe equivalent equationsr˙1 = 0θ˙1 = ω 1(7)r˙2 = 0θ˙2 = ω 2Hence all solutions in R 4 off the union of the two subspaces R 2 ×{(0, 0} ⋃ {0, 0}×R 2 lie on two dimensional tori r 1 = c 1 , r 2 = c 2 , and the motion on each is aconstant vector field. If ω 2 /ω 1 is rational, then all these orbits are periodic,and if ω 2 /ω 1 is irrational, then all these tori are minimal sets.