Robustness of Discrete-Time Direct Adaptive Controllers - Centre ...

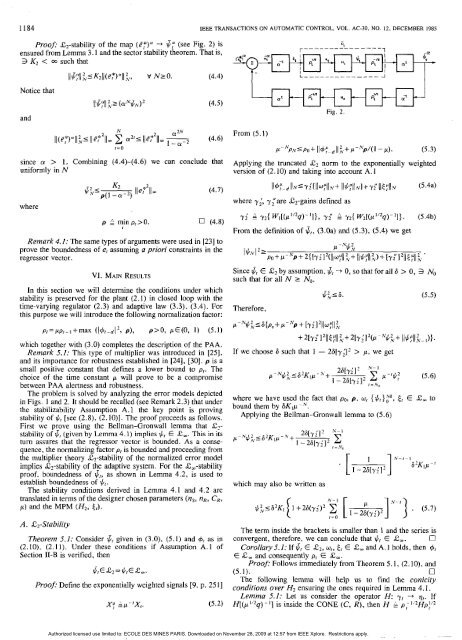
1 I84 IEEE TRAl(e,*)"Proof: &stability of the map --* $; (see Fig. 2) isensured from Lemma 3.1 and the sector stability theorem. That is,3 K2 < 03 such thatlllt~l,t...K*ll(et)~ll;~, v NrO. (4.4)VSACTIONS ON AUTOMATIC CONTROL, VOL. AC-30. NO. 12. DECEMBER 1985Notice that\~$~]~+(O1~~$:v)~ (4.5)Fig. 2.andsince CY > 1. Combining (4.4)-(4.6) we can conclude thatuniformly in Nwhere(4.7)p minp,>O. ti (4.8)Remark 4. I: The same types of arguments were used in [23] toprove the boundedness of e, assuming a priori constraints in theregressor vector.IVI. MAIN RESULTSIn this section we will determine the conditions under whichstability is preserved for the plant (2.1) in closed loop with thetime-varying regulator (2.3) and adaptive law (3.3). (3.4). Forthis purpose we will introduce the following normalization factor:Pr=w-l+max (I+r-d12, P), P>O, PE(O, 1) (5.1)which together with (3.0) completes the description of the PAA.Remark 5.1: This type of multiplier was introduced in [25].and its importance for robustness established in [24], [30]. p is asmall positive constant that defines a lower bound to p,. Thechoice of the time constant ,u will prove to be a compromisebetween PAA alertness and robustness.The problem is solved by analyzing the error models depictedin Figs. 1 and 2. It should be recalled (see Remark 2.3) that underthe stabilizability Assumption A.l the key point is provingstability of $[ [see (2.8). (2. IO)]. The proof proceeds as follows.First we prove using the Bellman-Gronwall lemma that &-stability of $I (given by Lemma 4.1) implies 3, E 2,. This in itsturn assures that the regressor vector is bounded. As a consequence,the normalizing factor p, is bounded and proceeding fromthe multiplier theory &-stability of the normalized error modelimplies &-stability of the adaptive system. For the &,-stabilityproof, boundedness of $,, as shown in Lemma 4.2, is used toestablish boundedness of $,.The stability conditions derived in Lema 4.1 and 4.2 aretranslated in terms of the designer chosen parameters (os, n,, C,,p) and the MPM (Hz, t,).A. &-StabilityTheorem 5. I: Consider $, given in (3.0), (5.1) and 41 as in(2.10). (2.1 1). Under these conditions if Assumption A.l ofSection 11-B is verified, then$IE.e2=)$r€Oe.r.Proof: Define the exponentially weighted signals [9. p. 25 1 Jwhere we have used the fact that PO, p , w, { $,}zo, {, Ebound them by 6K1p -.v.Applying the Bellman-Gronwall lemma to (5.6)which may also be written as,=oto. (5.7)The term inside the brackets is smaller than 1 and the series isconvergent, therefore, we can conclude that $, E C,. 0Corollary 5. I: If $, E 22, a,, 4, E Sm and A. 1 holds, then 4,E C , and consequently p, E 2,.Proof: Follows immediately from Theorem 5.1 ~ (2. lo), and(5.1). 0The following lemma will help us to find the coniciryconditions over Hz ensuring the ones required in Lemma 4.1.Lemma 5.1: Let us consider the operator H: y, + q,. Ifti[(p''2q) - I ] is inside the CONE (C, R), then H 2 p;I'*Hp:/'Authorized licensed use limited to: ECOLE DES MINES PARIS. Downloaded on November 28, 2009 at 12:57 from IEEE Xplore. Restrictions apply.