A multi-state HLL approximate Riemann solver for ideal ...

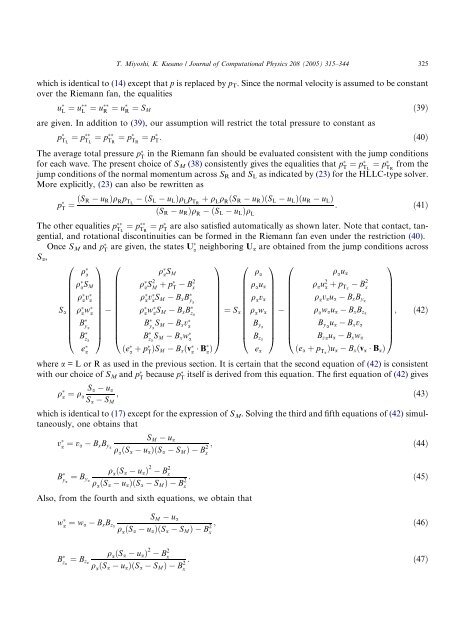
which is identical to (14) except that p is replaced by p T . Since the normal velocity is assumed to be constantover the Riemann fan, the equalitiesu L ¼ u L¼ u R ¼ u R ¼ S Mð39Þare given. In addition to (39), our assumption will restrict the total pressure to constant asp T L¼ p T L¼ p T R¼ p T R¼ p T : ð40ÞThe average total pressure p Tin the Riemann fan should be evaluated consistent with the jump conditionsfor each wave. The present choice of S M (38) consistently gives the equalities that p T ¼ p T L¼ p T Rfrom thejump conditions of the normal momentum across S R and S L as indicated by (23) for the HLLC-type solver.More explicitly, (23) can also be rewritten asp T ¼ ðS R u R Þq R p TLðS L u L Þq L p TRþ q L q R ðS R u R ÞðS L u L Þðu R u L Þ: ð41ÞðS R u R Þq R ðS L u L Þq LThe other equalities p T L¼ p T R¼ p Tare also satisfied automatically as shown later. Note that contact, tangential,and rotational discontinuities can be formed in the Riemann fan even under the restriction (40).Once S M and p T are given, the states U a neighboring U a are obtained from the jump conditions acrossS a ,0 1 0q aq a S 1 0 1 01Mq aq a u aq a S Mq a S2 M þ p TB 2 xq q a v aq a S aq v a S a u aq a u 2 a þ p T aB 2 xM B x B y aq a v aq a v a u a B x B yaa w aq a w a S M B x B z a¼ S aq a w aq a w a u a B x B za; ð42ÞB y aB y aS M B x v aB yaB y au a B x v aB@ B C Bz aA @ B z aS M B x w C B C BCa A @ B za A @ B za u a B x w a Aðe a þ p T ÞS M B x ðv a B a Þðe a þ p TaÞu a B x ðv a B a Þe awhere a = L or R as used in the previous section. It is certain that the second equation of (42) is consistentwith our choice of S M and p T because p Titself is derived from this equation. The first equation of (42) givesS au aT. Miyoshi, K. Kusano / Journal of Computational Physics 208 (2005) 315–344 325q a ¼ q a ; ð43ÞS a S Mwhich is identical to (17) except for the expression of S M . Solving the third and fifth equations of (42) simultaneously,one obtains thatv a ¼ v S M u aa B x B ya ; ð44Þq a ðS a u a ÞðS a S M Þ B 2 xe aB qy a¼ B a ðS a u a Þ 2 B 2 xya : ð45Þq a ðS a u a ÞðS a S M Þ B 2 xAlso, from the fourth and sixth equations, we obtain thatw a ¼ w S M u aa B x B za ; ð46Þq a ðS a u a ÞðS a S M Þ B 2 xB qz a¼ B a ðS a u a Þ 2 B 2 xza : ð47Þq a ðS a u a ÞðS a S M Þ B 2 x