8.4 Vector and Parametric Equations of a Plane A ... - La Citadelle

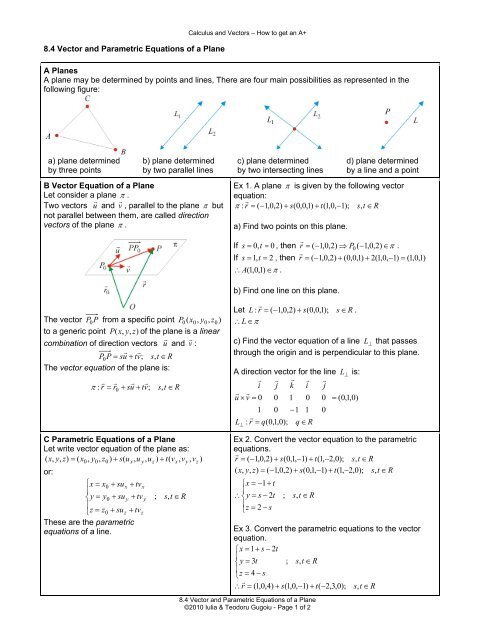
8.4 Vector and Parametric Equations of a PlaneCalculus and Vectors – How to get an A+A PlanesA plane may be determined by points and lines, There are four main possibilities as represented in thefollowing figure:a) plane determinedby three pointsb) plane determinedby two parallel linesB Vector Equation of a PlaneLet consider a plane π .Two vectors u r and v r , parallel to the plane π butnot parallel between them, are called directionvectors of the plane π .c) plane determinedby two intersecting linesd) plane determinedby a line and a pointEx 1. A plane π is given by the following vectorequation:π : r = ( −1,0,2)+ s(0,0,1)+ t(1,0,−1);s,t ∈ Ra) Find two points on this plane.If s = 0 , t = 0 , then r = ( −1,0,2)⇒ P0( −1,0,2)∈π.If s = 1 , t = 2 , then r = ( −1,0,2)+ (0,0,1) + 2(1,0, −1)= (1,0,1 )∴ A (1,0,1 )∈π .b) Find one line on this plane.The vector P 0 P from a specific point P 0( x0,y0,z0)to a generic point P( x,y,z)of the plane is a linearcombination of direction vectors u r and v r :r rP0P = su + tv;s,t ∈ RThe vector equation of the plane is:r r r rπ= + su + tv;s,t ∈ R: 0C Parametric Equations of a PlaneLet write vector equation of the plane as:( x , y,z)= ( x0,y0,z0) + s(ux, u y , uz) + t(vx, v y , vz)or:⎧x= x0+ sux+ tvx⎪⎨y= y0+ su y + tv y⎪⎩z= z0+ suz+ tvz; s,t ∈ RThese are the parametricequations of a line.Let L : r = ( −1,0,2)+ s(0,0,1); s ∈ R .∴L ∈πc) Find the vector equation of a line L ⊥ that passesthrough the origin and is perpendicular to this plane.A direction vector for the line L ⊥ is:r r r r ri j k i jr ru × v = 0 0 1 0 0 = (0,1,0)1 0 −11 0L⊥ : r = q(0,1,0); q ∈ REx 2. Convert the vector equation to the parametricequations.r= ( −1,0,2)+ s(0,1,−1)+ t(1,−2,0);s,t ∈ R( x,y,z)= ( −1,0,2)+ s(0,1,−1)+ t(1,−2,0);s,t ∈ R⎧x= −1+t⎪∴⎨y= s − 2t; s,t ∈ R⎪⎩z= 2 − sEx 3. Convert the parametric equations to the vectorequation.⎧x= 1+s − 2t⎪⎨y= 3t; s,t ∈ R⎪⎩z= 4 − sr∴ = (1,0,4) + s(1,0,−1)+ t(−2,3,0);s,t ∈ R8.4 Vector and Parametric Equations of a Plane©2010 Iulia & Teodoru Gugoiu - Page 1 of 2

Calculus and Vectors – How to get an A+Ex 4. (Plane determined by three points)Find the vector equation of the plane π thatpasses through the points A ( 0,1, −1), B ( 2, −1,0), andC (0,0,1) .Ex 5. (Plane determined by two parallel and distinctlines)Find the vector and parametric equations of the plane πthat contains the following parallel and distinct lines:rL1:= (1,2,1) + s(0,−1,−2);s ∈ RrL := (3,4,0) + t(0,1,2);t ∈ R2Let r 0 = OA = (0,1, −1), u r = AB = ( 2, −2,1), andv r = AC = ( 0, −1,2). Then:π : r = (0,1, −1)+ s(2,−2,1)+ t(0,−1,2);s,t ∈ REx 6. (Plane determined by two intersecting lines)Find the vector equation of the plane πdetermined by the following intersecting lines.rL1:= (0,0,1) + s(−1,0,0);s ∈ RrL := ( −3,0,1)+ t(0,0,2);t ∈ R2r rr r rLet 0 = 02 = (3,4,0), u = 01 −02 = ( −2,−2,1), andr rv = u 2 = (0,1,2) . Then:∴ π : r = (3,4,0) + s(−2,−2,1)+ t(0,1,2);s,t ∈ Rand⎧x= 3 − 2s⎪∴ π : ⎨y= 4 − 2s+ t ; s,t ∈ R⎪⎩z= s + 2tEx 7. (Plane determined by a line and an external point)Find the vector equation of the plane π that passesthrough the origin and contains the lineL : r = (0,1,2) + t(−1,0,3); t ∈ R .Let first find the point of intersection.⎧−s = −3⎪⎨0= 0 ⇒ s = −3and t = 0⎪⎩1= 1+2tP0 = L1∩ L2⇒ P0= ( −3,0,1)Let r r r0 = OP0= ( −3,0,1) , u = u 2 = (0,0,2), andr rv = u1 = ( −1,0,0). Then:∴ π : r = ( −3,0,1)+ s(0,0,2)+ t(−1,0,0);s,t ∈ RLet r 0 = (0,0,0), u r = (−1,0,3 ) , andv r = ( 0,1,2) − (0,0,0) = (0,1,2) . Then the vector equation ofthe plane π is:∴ π : r = s(−1,0,3)+ t(0,1,2);s,t ∈ RReading: Nelson Textbook, Pages 453-458Homework: Nelson Textbook: Page 459 #1, 2, 4, 6b, 7, 9, 10, 158.4 Vector and Parametric Equations of a Plane©2010 Iulia & Teodoru Gugoiu - Page 2 of 2