Chapter 11 Gravity

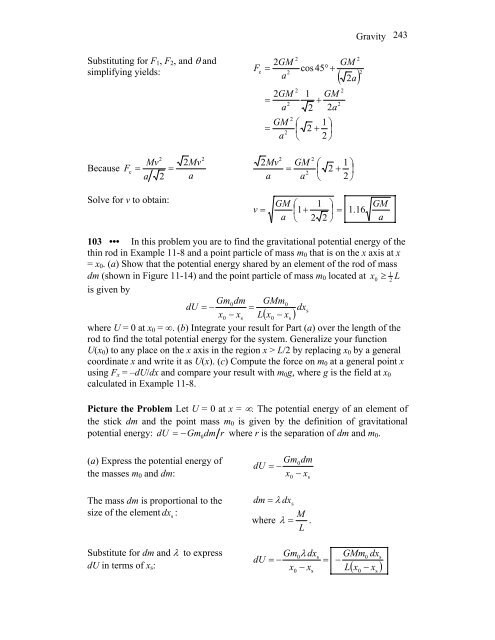
Substituting for F1, F2, and θ and
2
2GM
GM
F = cos45°
+
simplifying yields: c 2
a
( 2a)
Because
2GM
= 2
a
GM
= 2
a
2
2
⎛
⎜
⎝
1 GM
+
2 2a
1 ⎞
2 + ⎟
2 ⎠
2
2
Mv 2Mv
Fc
a 2 a
= = 2
2
2Mv
GM ⎛ 1 ⎞
= ⎜ 2 +
2 ⎟
a a ⎝ 2 ⎠
Solve for v to obtain:
GM ⎛ 1 ⎞
v = ⎜1
+ ⎟ = 1.
16
a ⎝ 2 2 ⎠
2
2
Gravity
2
2
GM
a
103 ••• In this problem you are to find the gravitational potential energy of the
thin rod in Example 11-8 and a point particle of mass m0 that is on the x axis at x
= x0. (a) Show that the potential energy shared by an element of the rod of mass
1
dm (shown in Figure 11-14) and the point particle of mass m0 located at x0 ≥ 2 L
is given by
Gm0dm
GMm0
dU = − = dxs
x0
− xs
L(
x0
− xs
)
where U = 0 at x0 = ∞. (b) Integrate your result for Part (a) over the length of the
rod to find the total potential energy for the system. Generalize your function
U(x0) to any place on the x axis in the region x > L/2 by replacing x0 by a general
coordinate x and write it as U(x). (c) Compute the force on m0 at a general point x
using Fx = –dU/dx and compare your result with m0g, where g is the field at x0
calculated in Example 11-8.
Picture the Problem Let U = 0 at x = ∞. The potential energy of an element of
the stick dm and the point mass m0 is given by the definition of gravitational
potential energy: = −Gm
dm r where r is the separation of dm and m0.
dU 0
(a) Express the potential energy of
Gm0dm
dU = −
x − x
the masses m0 and dm: 0 s
The mass dm is proportional to the
size of the element dxs
:
Substitute for dm and λ to express
dm = λ dxs
M
where λ = .
L
Gm0λ
dx
dU = −
dU in terms of xs: x − x L(
x − x )
0
s
s
=
GMm
−
0
0
dx
s
s
243