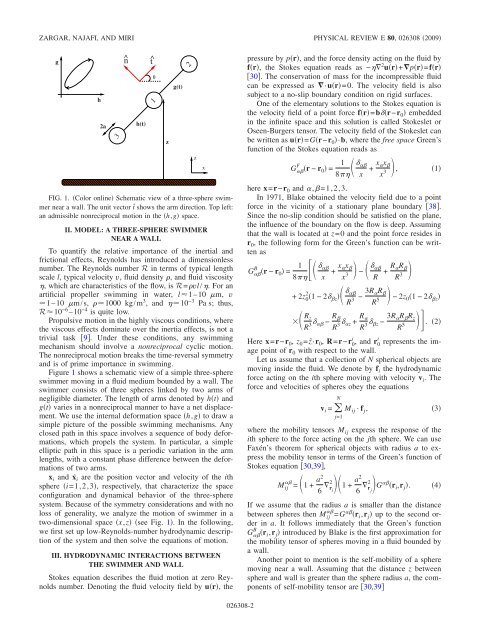
Three-sphere low-Reynolds-number swimmer near a wall

ZARGAR, NAJAFI, AND MIRIg^n ^th2a3II. MODEL: A THREE-SPHERE SWIMMERNEAR A WALLTo quantify the relative importance of the inertial andfrictional effects, Reynolds has introduced a dimensionlessnumber. The Reynolds number R in terms of typical lengthscale l, typical velocity v, fluid density , and fluid viscosity, which are characteristics of the flow, is R=vl/. For anartificial propeller swimming in water, l1–10 m, v1–10 m/s, 1000 kg/m 3 , and 10 −3 Pa s; thus,R10 −6 –10 −4 is quite low.Propulsive motion in the highly viscous conditions, wherethe viscous effects dominate over the inertia effects, is not atrivial task 9. Under these conditions, any swimmingmechanism should involve a nonreciprocal cyclic motion.The nonreciprocal motion breaks the time-reversal symmetryand is of prime importance in swimming.Figure 1 shows a schematic view of a simple three-sphereswimmer moving in a fluid medium bounded by a wall. Theswimmer consists of three spheres linked by two arms ofnegligible diameter. The length of arms denoted by ht andgt varies in a nonreciprocal manner to have a net displacement.We use the internal deformation space h,g to draw asimple picture of the possible swimming mechanisms. Anyclosed path in this space involves a sequence of body deformations,which propels the system. In particular, a simpleelliptic path in this space is a periodic variation in the armlengths, with a constant phase difference between the deformationsof two arms.x i and ẋ i are the position vector and velocity of the ithsphere i=1,2,3, respectively, that characterize the spaceconfiguration and dynamical behavior of the three-spheresystem. Because of the symmetry considerations and with noloss of generality, we analyze the motion of swimmer in atwo-dimensional space x,z see Fig. 1. In the following,we first set up low-Reynolds-number hydrodynamic descriptionof the system and then solve the equations of motion.III. HYDRODYNAMIC INTERACTIONS BETWEENTHE SWIMMER AND WALLStokes equation describes the fluid motion at zero Reynoldsnumber. Denoting the fluid velocity field by ur, theh(t)θg(t)1 2FIG. 1. Color online Schematic view of a three-sphere swimmernear a wall. The unit vector tˆ shows the arm direction. Top left:an admissible nonreciprocal motion in the h,g space.zzxpressure by pr, and the force density acting on the fluid byfr, the Stokes equation reads as − 2 ur+pr=fr30. The conservation of mass for the incompressible fluidcan be expressed as ·ur=0. The velocity field is alsosubject to a no-slip boundary condition on rigid surfaces.One of the elementary solutions to the Stokes equation isthe velocity field of a point force fr=br−r 0 embeddedin the infinite space and this solution is called Stokeslet orOseen-Burgers tensor. The velocity field of the Stokeslet canbe written as ur=Gr−r 0 ·b, where the free space Green’sfunction of the Stokes equation reads asG F r − r 0 = 18 x+ x x x 3 , 1here x=r−r 0 and ,=1,2,3.In 1971, Blake obtained the velocity field due to a pointforce in the vicinity of a stationary plane boundary 38.Since the no-slip condition should be satisfied on the plane,the influence of the boundary on the flow is deep. Assumingthat the wall is located at z=0 and the point force resides inr 0 , the following form for the Green’s function can be writtenasG B r − r 0 = 18 xPHYSICAL REVIEW E 80, 026308 2009+2z 0 2 1−2 z + x x x 3 − R + R R R 3R 3 − 3R R R 5 −2z 0 1−2 z R zR 3 − R R 3 z + R R 3 z − 3R R R zR 5 . 2Here x=r−r 0 , z 0 =ẑ·r 0 , R=r−r i i0 , and r 0 represents the imagepoint of r 0 with respect to the wall.Let us assume that a collection of N spherical objects aremoving inside the fluid. We denote by f i the hydrodynamicforce acting on the ith sphere moving with velocity v i . Theforce and velocities of spheres obey the equationsNv i = M ij · f j ,3j=1where the mobility tensors M ij express the response of theith sphere to the force acting on the jth sphere. We can useFaxén’s theorem for spherical objects with radius a to expressthe mobility tensor in terms of the Green’s function ofStokes equation 30,39,M ij =1+ a26 r21+ a2i 6 r2G rji ,r j . 4If we assume that the radius a is smaller than the distancebetween spheres then M ij =G r i ,r j up to the second orderin a. It follows immediately that the Green’s functionG B r i ,r j introduced by Blake is the first approximation forthe mobility tensor of spheres moving in a fluid bounded bya wall.Another point to mention is the self-mobility of a spheremoving near a wall. Assuming that the distance z betweensphere and wall is greater than the sphere radius a, the componentsof self-mobility tensor are 30,39026308-2