2. Radiation Field Basics I Specific Intensity Ω = dddd cos d tA I E

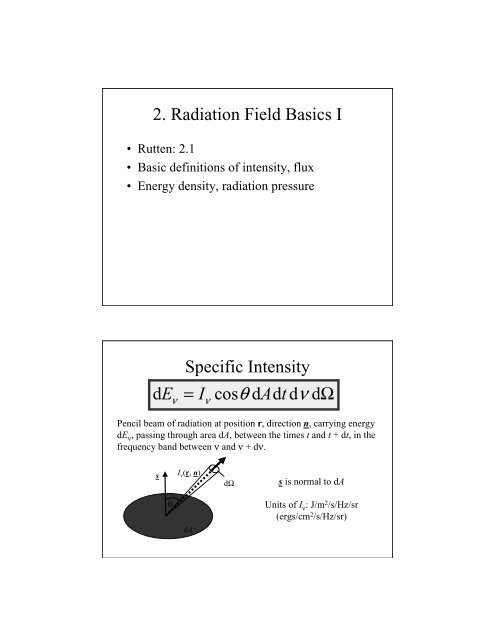
2. Radiation Field Basics I• Rutten: 2.1• Basic definitions of intensity, flux• Energy density, radiation pressuredEνSpecific Intensity= I cosθdAdtdνdΩνPencil beam of radiation at position r, direction n, carrying energydE ν , passing through area dA, between the times t and t + dt, in thefrequency band between ν and ν + dν.sI ν (r, n)dΩs is normal to dAθdAUnits of I ν : J/m 2 /s/Hz/sr(ergs/cm 2 /s/Hz/sr)

ss’dEνp θ θ’ p’rdAdA’No sources or sinks of radiation. Pencil beam of radiation passingthrough dA at p and dA’ at p’. Radiant energy passing through bothareas is the same:' '= I cosθdAdtdνdΩ = dE= I cosθ'dA'dtdνdΩ'νSolid angle dΩ subtended by dA’ at p, dΩ’ subtended by dA at p’:dΩ = dA'cos' / r; d2θ Ω ='dAcosθ/ rν2νTherefore:'Iν= I νSpecific intensity independent of distance when no sources or sinks.Solar Limb DarkeningθAssume plane parallel atmosphereMeasure I at different positions on solar disk => get I(θ)

JJν=14IMean Intensity1dΩ =4π2πνπ 0 0πIνsinθdθdφJ ν is a very important quantity. It determines level populationsand ionization state throughout atmosphere. Like I ν , it is afunction of position. For a plane parallel atmosphere (no φdependence) with µ = cos θ:ν11 1( z)= Iν( z,θ )2πsinθdθ= Iν( z,µ )dµ4π2 −1We’ll later use moments of the radiation field. J ν is the 0 th momentMoment operator M operating on f :1( ) 1M n [ f ] = f µ n dµ2 −1R *θ *I *rWhat is J ν at distance r from a star with uniform specific intensityI * across its surface?I = I * for 0 < θ < θ * (µ * < µ < 1)I = 0 for θ > θ * (µ < µ * )JJ=12= I*1µ12I d=I (1 −µ 12 *µ* w is the dilution factor*2 2( 1−1−R*/ r ) = w I*)At large r, w = R 2 /4r 2

¢¢££££¢¢¡¢£¢¡£££££FluxThe flux enables us to calculate the total energy, E, passing througha surface in a given time, i.e., integrated over all directions. Theenergy transport can be positive or negative.E = dEdΩ = dνdtI ( r,n,t)nˆ• dAdΩνMonochromatic Flux, ν : The net flow of radiant energy per secondthrough an area dA in time dt in frequency range dν.νν=IνcosθdΩ =2π0π0IνcosθsinθdθdφThis is used for specifying the energetics of radiation through stellarinteriors, atmospheres, ISM, etc. In principle, flux is a vector.In stellar atmospheres, the outward radial direction is always impliedpositive, so thatν( z)==≡2π02π0+νπ / 20π / 20( z)−I cosθsinθdθdφ+I cosθsinθdθdφ−ννν( z)2π02π0πνπ / 2πνπ / 2I cosθsinθdθdφI ( π −θ)cosθsinθdθdφWith both the outward flux, ν + , and the inward flux, ν − , positive.Isotropic radiation has ν + = ν− = πI ν and ν = 0. Axisymmetry:ν( z)= 2π01= 2πI=£+νπν0I cosθsinθdθdφν( z)−µ dµ− 2πν( z)−10I µ dµν

The flux emitted by a star per unit area of its surface isν = ν + = πI ν∗ where I ν∗ is the intensity, averaged over the apparentstellar disk, received by an observer. This equality is why that fluxis often written as πF = , so that F = I ∗ , with F called theAstrophysical Flux.This explains the often confusing factors of π that are floating aboutin definitions of flux:= Monochromatic Flux or just the Flux; F = Astrophysical FluxThey are related by πF = .In terms of moments of the radiation field, the first moment isdefined as the Eddington Flux, H ν . For plane parallel geometry:Hν≡4π1 ν 1IνcosθdΩ = =1−1ν=4πF42Iνµ dµFlux = energy/second per areaLuminosity = energy/secondStellar LuminosityLν=νA*= 4π R2*π IνAssume I ν = B ν and integrate:L =L2νdν= 4πR* π Bνdν= 4π R2*σ T4

Unresolved SourcesRelate energy observed to ν at stellar surface:Energy received per detector area, from anulus: df ν= Iνdωdω = solid angle of anulusAnulus area (r = R * sin θ):θdS = 2πr dr= 2πµ dµR *rdSfνdω = dS /D 2Integrate over ω:2R *2= 2π( R*/ D)I(R*, µ , ν ) µ ¤dµ02= ( R / D)R , ν )1 2α*Rα¢¡* = angular diameter=*,ν )4Unresolved => measure fluxInverse square law. Know α * , get absolute flux at star*£¡1*Energy DensityThe energy flow in a beam of radiation isdVds = c dtnθdAdEν= I cosθdAdtdνdΩThe flow has velocity c (photons) andtravels a distance ds in time dt = ds/cthrough volume dV = dA ds cos θ. Thus,each beam carries dE ν = (1/c) I ν dΩ dV.If multiple beams pass through a smallvolume ∆V, integration over ∆V and overall beam directions gives the radiantenergy E ν dν contained in ∆V acrossbandwidth dν as:1νd ν ¥ ¥ E = IνdVdΩdνc ∆VΩν

For sufficiently small ∆V, the intensity is homogeneous, so thetwo integrations (V, Ω) are independent. The energy density isu1= dΩcνI νRadiation PressureEach photon has momentum p = hν/c. Component of momentumnormal to a solid wall per time per area isdpν=1 dEνcosθc dAdtRe-write in terms of I ν and integrating over solid angle gives:n p = hν/cθdAhν/c cos θds = c dtp1= cos 2θ dΩcνI νIsotropic radiation has p ν = u ν /3.Radiation pressure is analogous togas pressure, being the pressure ofthe photon gas.