Change in Variables; Cylindrical, Spherical Coordinates

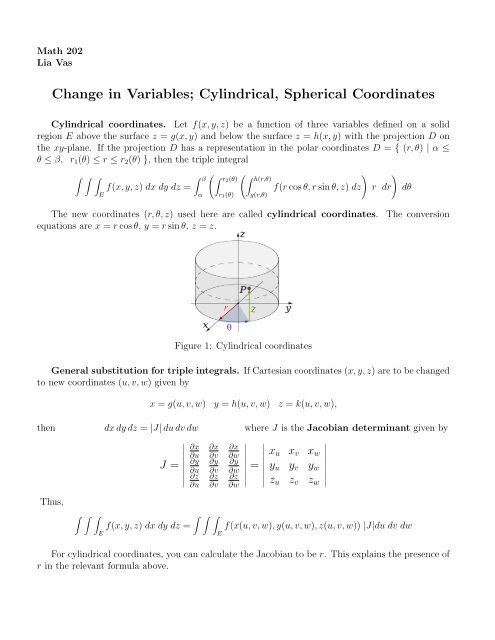
Math 202Lia VasChange in Variables; Cylindrical, Spherical CoordinatesCylindrical coordinates. Let f(x, y, z) be a function of three variables defined on a solidregion E above the surface z = g(x, y) and below the surface z = h(x, y) with the projection D onthe xy-plane. If the projection D has a representation in the polar coordinates D = { (r, θ) | α ≤θ ≤ β, r 1 (θ) ≤ r ≤ r 2 (θ) }, then the triple integral∫ ∫ ∫Ef(x, y, z) dx dy dz =∫ ( β ∫ (r2 (θ) ∫ h(r,θ)αr 1 (θ)g(r,θ)f(r cos θ, r sin θ, z) dz)r dr)dθThe new coordinates (r, θ, z) used here are called cylindrical coordinates.equations are x = r cos θ, y = r sin θ, z = z.The conversionFigure 1: Cylindrical coordinatesGeneral substitution for triple integrals. If Cartesian coordinates (x, y, z) are to be changedto new coordinates (u, v, w) given byx = g(u, v, w) y = h(u, v, w) z = k(u, v, w),then dx dy dz = |J| du dv dw where J is the Jacobian determinant given byThus,∫ ∫ ∫EJ =∂x∂u∂y∂u∣∂z∂u∂x∂v∂y∂v∂z∂v∫ ∫ ∫f(x, y, z) dx dy dz =E∂x∂w∂y∂w∂z∣∂w=∣∣x u x v x ∣∣∣∣∣∣∣∣wy u y v y wz u z v z wf(x(u, v, w), y(u, v, w), z(u, v, w)) |J|du dv dwFor cylindrical coordinates, you can calculate the Jacobian to be r. This explains the presence ofr in the relevant formula above.

General substitution for double integrals. For double integrals, if Cartesian coordinates(x, y) are to be changed to new coordinates (u, v) given by x = g(u, v) y = h(u, v), then dxdy =|J|dudv where the Jacobian determinant J is given by∣ ∂x ∂x ∣Then∫ ∫DJ =∣∂u∂y∂u∂v∂y∂v∫ ∫f(x, y) dx dy =D∣ ∣ ∣∣∣∣ ∣ = x u x ∣∣∣∣ v.y u y vf(x(u, v), y(u, v)) |J|du dvCheck that the polar coordinates x = r cos θ and y = r sin θ have the Jacobian determinant J = r.This is the reason why the substitution with polar coordinates yields∫ ∫∫ ∫f(x, y)dxdy = f(r cos θ, r sin θ) r dr dθ.DSpherical coordinates. Besides cylindrical coordinates, another frequently used coordinates fortriple integrals are spherical coordinates. Spherical coordinates are mostly used for the integralsover a solid whose definition involves spheres.If P = (x, y, z) is a point in space and O denotes the origin, let• r denote the length of the vector −→ OP = 〈x, y, z〉, i.e. the distance of the point P = (x, y, z)from the origin O. Thus,x 2 + y 2 + z 2 = r 2 ;• θ be the angle between the projection of vector −→ OP = 〈x, y, z〉 on the xy-plane and the vector−→ i (positive x axis); and• φ be the angle between the vector −→ OP and the vector −→ k (positive z-axis).With this notation, spherical coordinates are (r, θ, φ). The conversion equations arex = r cos θ sin φ y = r sin θ sin φ z = r cos φThe Jacobian determinant for the spherical coordinates is r 2 sin φ. Thus,dx dy dz = r 2 sin φ dr dφ dθ.Practice problems.Figure 2: Spherical coordinates

1. Evaluate the triple integrala) ∫ ∫ ∫ E√x2 + y 2 dx dy dz where E is the region that lies inside the cylinder x 2 + y 2 = 16and between the planes z = −5 and z = 4.b) ∫ ∫ ∫ E 2 dx dy dz where E is the solid that lies between the cylinders x2 +y 2 = 1 x 2 +y 2 = 4and between the xy-plane and the plane z = x + 2.c) ∫ ∫ ∫ E (x2 + y 2 + z 2 ) dx dy dz where E is the unit ball x 2 + y 2 + z 2 ≤ 1.d) ∫ ∫ ∫ E z dx dy dz where E is the region between the spheres x2 + y 2 + z 2 = 1 andx 2 + y 2 + z 2 = 4 in the first octant.2. Find the volume of the solid enclosed by the paraboloids z = x 2 + y 2 and z = 36 − 3x 2 − 3y 2 .3. Find the volume of the solid enclosed by the paraboloids z = x 2 + y 2 and z = 18 − x 2 − y 2 .4. Use the given transformation to evaluate the integral.a) ∫ ∫ D (3x+4y) dx dy where D is the region bounded by the lines y = x, y = x−2, y = −2x,and y = 3 − 2x. The substitution x = 1/3(u + v), y = 1/3(v − 2u) transforms the regionto a rectangle 0 ≤ u ≤ 2 and 0 ≤ v ≤ 3.b) ∫ ∫ D xy dx dy where D is the region in the first quadrant bounded by the curves y = x,y = 3x, y = 1/x, and y = 3/x. The substitution x = u/v, y = v transforms the regioninto a region with bounds 1 ≤ u ≤ 3 and √ u ≤ v ≤ √ 3u.c) ∫ ∫ D xy dx dy where D is the region in the first quadrant bounded by the curves y = x,y = 3x, y = 1/x, and y = 3/x. The substitution x = √ u, y = √ uv transforms the regionvinto a square 1 ≤ u ≤ 3 and 1 ≤ v ≤ 3.5. Find the volume of the ellipsoid x 2 /4 + y 2 /9 + z 2 /25 = 1 by using the transformation x = 2u,y = 3v z = 5w.Solutions.1. a) Use cylindrical coordinates. The interior of the circle x 2 +y 2 = 16 can be described by 0 ≤ θ ≤2π and 0 ≤ r ≤ 4. The bounds for z are given by z = −5 and z = 4. ∫ ∫ ∫ √E x2 + y 2 dx dy dz =∫ 2π ∫ 4 ∫ 4√0 0 −5 r2 r dr dθ dz = ∫ 2π0 dθ ∫ 40 r2 dr ∫ 4−5 dz = 2π 64 (4 + 5) = 384π.3b) The region between the circles x 2 + y 2 = 1 x 2 + y 2 = 4 has 0 ≤ θ ≤ 2π and 1 ≤ r ≤ 2.The bounds for z are xy-plane z = 0 and the plane z = x + 2 which in polar coordinateshas the equation z = r cos θ + 2. Thus, using the cylindrical coordinates, ∫ ∫ ∫ E 2 dx dy dz =∫ 2π ∫ 2 ∫ r cos θ+20 1 0 2r dr dθ dz = ∫ 2π0 dθ ∫ 21 2r dr (r cos θ + 2) = ∫ 2π0 dθ (2 r3 cos θ + 3 2r2 )| 2 1 =∫ 2π0 dθ ( 14 cos θ + 6) = 12π.3c) Using spherical coordinates, 0 ≤ θ ≤ 2π, 0 ≤ φ ≤ π, and 0 ≤ r ≤ 1. ∫ ∫ ∫ E x2 + y 2 +z 2 dx dy dz = ∫ 2π02π(2) 1 = 4π.5 5∫ π0∫ 10 r2 r 2 sin φdr dθ dφ = ∫ 2π0 dθ ∫ π0 sin φdφ ∫ 10 r4 dr = 2π(− cos φ)| π 0 r5 5 |1 0 =d) Use spherical coordinates. Since the region is in the first octant, 0 ≤ θ ≤ π and 0 ≤ φ ≤ π.2 2The bounds for r are determined by the radii of the spheres, so 1 ≤ r ≤ 2. ∫ ∫ ∫ E z dx dy dz =∫ π/2 ∫ π/2 ∫ 20 0 1 r cos φ r2 sin φdr dθ dφ = ∫ π/20 dθ ∫ π/20 cos φ sin φdφ ∫ 21 r3 dr = π 1 r 42 2 4 |2 1 = 15π16

2. Use cylindrical coordinates. The paraboloids have the equations z = x 2 + y 2 = r 2 and z =36 − 3x 2 − 3y 2 = 36 − 3r 2 . The first is the lower z-bound and the second is the upper. Thebounds for θ are 0 ≤ θ ≤ 2π. The paraboloids intersect in a circle. The projection of the circlein xy-plane determines the r-bounds. The intersection is when 36 − 3r 2 = r 2 ⇒ 36 = 4r 2 ⇒9 = r 2 ⇒ r = 3 (negative solution is not relevant). Thus, the r-bounds are 0 ≤ r ≤ 3.The volume is V = ∫ ∫ ∫ dxdydz = ∫ 2π02π(18r 2 − r 4 )| 3 0 = 2π(162 − 81) = 162π.∫ 30∫ 36−3r 2r 2r dr dθ dz = ∫ 2π0 dθ ∫ 30 r dr(36 − 3r2 − r 2 ) =3. Very similar to the previous problem. The z-bounds are x 2 +y 2 = r 2 ≤ z ≤ 18−x 2 −y 2 = 18−r 2 .The bounds for θ are 0 ≤ θ ≤ 2π. The intersection of paraboloids is when 18 − r 2 = r 2 ⇒18 = 2r 2 ⇒ 9 = r 2 ⇒ r = 3 (negative solution is not relevant). Thus, the r-bounds are0 ≤ r ≤ 3. The volume is V = ∫ ∫ ∫ dxdydz = ∫ 2π0r 2 − r 2 ) = 2π(9r 2 − r4 2 )|3 0 = 2π(81 − 81) = 81π.2∣ x4. a) Calculate the Jacobian J = u x ∣∣∣∣ v=∣ y u y v ∣∫ 2 ∫ 30 0 (u + v + 4(v − 2u)) 1du dv = 1 3 3 31(6 + 9 + 12 − 16) = 11 3 3∣ xb) The Jacobian is J = u x ∣∣∣∣ v=∣ y u y v ∣∫ 31 udu ln v|√ √3uu= ∫ 31 udu ln √ 3 = ln √ 3 u2c) The Jacobian is J =∫ 3 ∫ 31 1√ uv√ uv1du dv = ∫ 3 ∫ 32v 1 1∫ 301/3 1/3−2/3 1/3∫ 18−r 2r 2∫ 2 v20 (uv + + 4v2 − 8uv2 6 3 )|3 0 du = 1 31/v −u/v 20 1r dr dθ dz = ∫ 2π0 dθ ∫ 30 r dr(18 −∣ = 1 + 2 = 1. ∫ ∫ 9 9 3 D (3x + 4y) dx dy =∫ 20 (3u + 9 + 6 − 8u) du =2∣ = 1. ∫ ∫ v D xy dx dy = ∫ 312 |3 1 = 4 ln √ 3 = 2 ln 3 = 2.197∣ x u x ∣∣∣∣ 1v=2 √ − √ uuv 2 √ √ √v ∣ y u y 3vv ∣2 √ uu 2 √ ∣vu2vdu dv =u24 |3 1 ln v| 3 1 = 2 ln 3 = 2.197∫ √ 3u√ uuv 1 du dv =v v= 1 + 1 = 1 . ∫ ∫ 4v 4v 2v D xy dx dy =5. The substitution x = 2u, y = 3v and z = 5w converts the ellipsoid into a sphere of radius2 0 01. The Jacobian of the substitution is J =0 3 0= 30. Thus, the volume is equal to∣ 0 0 5 ∣V = ∫ ∫ ∫ dx dy dz = ∫ ∫ ∫ 30 du dv dw. Use the spherical coordinates now. The bounds are0 ≤ θ ≤ 2π, 0 ≤ φ ≤ π, and 0 ≤ r ≤ 1 and the Jacobian is r 2 sin φ. Thus, the volumeis V = ∫ ∫ ∫ 30 r 2 sin φ dr dφ dθ = 30 ∫ 2π0 dθ ∫ π0 sin φ dφ ∫ 10 r2 dr = 30 2π (− cos φ)| π 0 r3 3 |1 0 =120π 1 = 40π.3