DISCRETE MATHEMATICS THROUGH GUIDED DISCOVERY ...

elements in common. For example, the sets {1, 3, 12} and {6, 4, 8, 2} are
disjoint, but {1, 3, 12} and {3, 5, 7} are not disjoint sets. Three or more sets
are said to be mutually disjoint if no two of the sets have any elements
in common. To solve Problem 9(c), the set of all possible choices of three
pints of ice cream can be broken into three mutually disjoint sets: Three
pints of different flavors; three pints with two different flavors; three pints
of the same flavor. The total number of choices is the sum of the sizes of
these three mutually disjoint sets.
•10. (a) What can you say about the size of the union of a finite number
of mutually disjoint finite sets?
(b) What can you say about the size of the union of m mutually
disjoint sets, each of the same size n? This is a fundamental
principle of “counting” or enumerative combinatorics.
(c) Find at least one of the previous problems that can be solved
by the counting principle in part (b). Explain how to solve your
chosen problem using that principle.
The problems you’ve just completed contain among them kernels of the
fundamentals of enumerative combinatorics. For example, in your solution
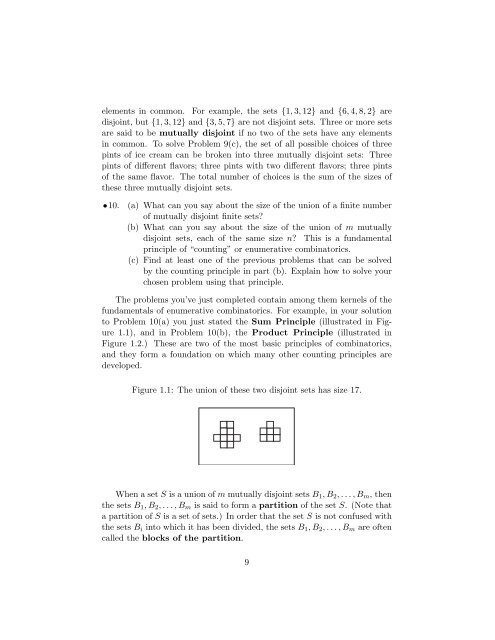
to Problem 10(a) you just stated the Sum Principle (illustrated in Figure
1.1), and in Problem 10(b), the Product Principle (illustrated in
Figure 1.2.) These are two of the most basic principles of combinatorics,
and they form a foundation on which many other counting principles are
developed.
Figure 1.1: The union of these two disjoint sets has size 17.
When a set S is a union of m mutually disjoint sets B1, B2, . . . , Bm, then
the sets B1, B2, . . . , Bm is said to form a partition of the set S. (Note that
a partition of S is a set of sets.) In order that the set S is not confused with
the sets Bi into which it has been divided, the sets B1, B2, . . . , Bm are often
called the blocks of the partition.
9