Chapter 3 Linear transformations

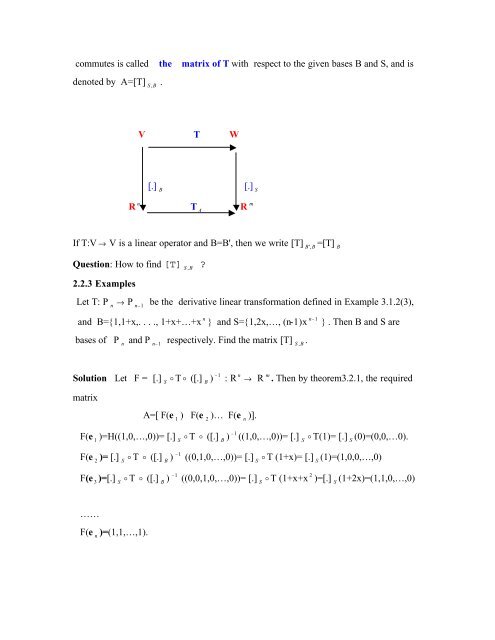
commutes is called the matrix of T with respect to the given bases B and S, and isdenoted by A=[T]S, B.V T W[.] B[.] SR n T AR mIf T:V → V is a linear operator and B=B', then we write [T]Question: How to find [T] S, B?2.2.3 ExamplesB ',B=[T] BLet T: P n→ P n −1be the derivative linear transformation defined in Example 3.1.2(3),and B={1,1+x,. . . ., 1+x+…+x n } and S={1,2x,…, (n-1)x n −1bases of P nand P n −1respectively. Find the matrix [T]S, B.} . Then B and S areSolution Let F = [.] So T o ([.] B) − 1: R n → R m . Then by theorem3.2.1, the requiredmatrixA=[ F(e 1) F(e 2)… F(e n)].F(e 1)=H((1,0,…,0))= [.] So T o ([.] B) − 1 ((1,0,…,0))= [.] So T(1)= [.] S(0)=(0,0,…0).F(e 2)= [.] So T o ([.] B) − 1F(e 3)=[.] So T o ([.] B) − 1((0,1,0,…,0))= [.] So T (1+x)= [.] S(1)=(1,0,0,…,0)((0,0,1,0,…,0))= [.] So T (1+x+x 2 )=[.] S(1+2x)=(1,1,0,…,0)……F(e n)=(1,1,…,1).