Learning Session Three – Tiling patterns - teacher

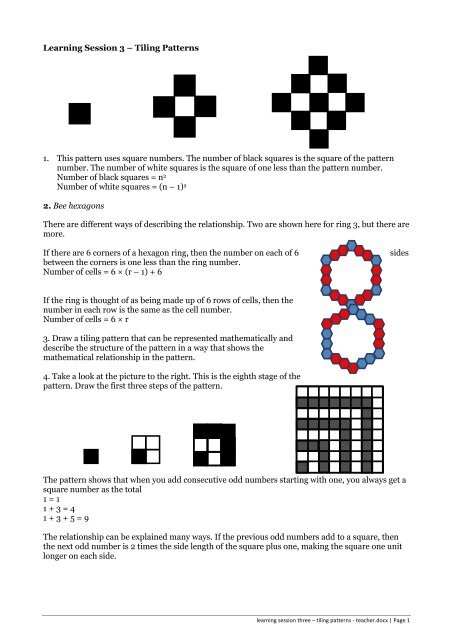
Learning Session 3 – Tiling Patterns1. This pattern uses square numbers. The number of black squares is the square of the patternnumber. The number of white squares is the square of one less than the pattern number.Number of black squares = n 2Number of white squares = (n – 1) 22. Bee hexagonsThere are different ways of describing the relationship. Two are shown here for ring 3, but there aremore.If there are 6 corners of a hexagon ring, then the number on each of 6between the corners is one less than the ring number.Number of cells = 6 × (r – 1) + 6sidesIf the ring is thought of as being made up of 6 rows of cells, then thenumber in each row is the same as the cell number.Number of cells = 6 × r3. Draw a tiling pattern that can be represented mathematically anddescribe the structure of the pattern in a way that shows themathematical relationship in the pattern.4. Take a look at the picture to the right. This is the eighth stage of thepattern. Draw the first three steps of the pattern.The pattern shows that when you add consecutive odd numbers starting with one, you always get asquare number as the total1 = 11 + 3 = 41 + 3 + 5 = 9The relationship can be explained many ways. If the previous odd numbers add to a square, thenthe next odd number is 2 times the side length of the square plus one, making the square one unitlonger on each side.learning session three – tiling patterns - teacher.docx | Page 1

If each odd number is thought of as twice a counting number plus one (2n + 1), then the square ismade up of a diagonal row of single squares plus twice a counting number each time you add a newlayer.Hyperlink 3. 4Hint: Try starting at the bottom left corner and add up the numbers in each stripe as you move tothe top right.What sort of numbers do the stripes add to?1 = 11 + 3 = 41 + 3 + 5 = 9…3. Students could debate this, and give evidence supporting their reasoning. In fact,mathematically, 0.999…. does equal 1, because the fractions extend into infinity, so you cannotdefine a point where 0.999… is not equal to one.4. If you look at the L shape of 3 quarters of the square, 1 of the 3 quarters is unshaded, or onethird of the area. If we look at the remaining quarter, and look at the L shape of 3 sixteenths, 1of the 3 sixteenths is unshaded, or one third of the area, and so on and so on. No matter howsmall the final L shape is, one third of it will be unshaded, so the relationship is: 1/4 + 1/16+ 1/64 + ... = 1/3Hyperlink 3.6Hint: Look at the square divided into quarters. Ignore the top right quarter. What fractionof the L shape is un-shaded? Now look at the top right quarter, divide it into quarters andjust look at the L shape. What fraction is un-shaded?learning session three – tiling patterns - teacher.docx | Page 2

5. 1/2 + 1/4 + 1/8 + 1/16 + ... = 1Hyperlink 3.7Hint: Divide the square into half and colour one half, then divide the uncoloured half again andcolour half of it, repeat as many times as you can.6. 1/3 + 1/9 + 1/27 + 1/81 + ... = 1/2learning session three – tiling patterns - teacher.docx | Page 3