Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
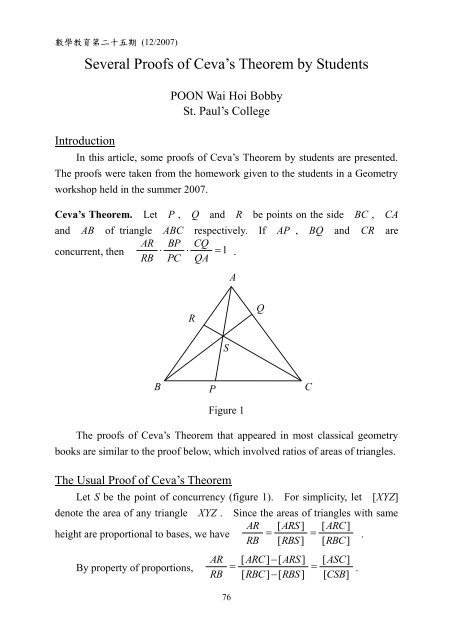
數 學 教 育 第 二 十 五 期 (12/2007)<strong>Several</strong> <strong>Pro<strong>of</strong>s</strong> <strong>of</strong> Ceva’s <strong>Theorem</strong> <strong>by</strong> <strong>Students</strong>IntroductionPOON Wai Hoi Bob<strong>by</strong>St. Paul’s CollegeIn this article, some pro<strong>of</strong>s <strong>of</strong> Ceva’s <strong>Theorem</strong> <strong>by</strong> students are presented.The pro<strong>of</strong>s were taken from the homework given to the students in a Geometryworkshop held in the summer 2007.Ceva’s <strong>Theorem</strong>.Let P , Q and R be points on the side BC , CAand AB <strong>of</strong> triangle ABC respectively. If AP , BQ and CR areAR BP CQconcurrent, then 1.RB PC QAARQSBPFigure 1CThe pro<strong>of</strong>s <strong>of</strong> Ceva’s <strong>Theorem</strong> that appeared in most classical geometrybooks are similar to the pro<strong>of</strong> below, which involved ratios <strong>of</strong> areas <strong>of</strong> triangles.The Usual Pro<strong>of</strong> <strong>of</strong> Ceva’s <strong>Theorem</strong>Let S be the point <strong>of</strong> concurrency (figure 1). For simplicity, let [XYZ]denote the area <strong>of</strong> any triangle XYZ . Since the areas <strong>of</strong> triangles with sameAR [ ARS ] [ ARC]height are proportional to bases, we have .RB [ RBS ] [ RBC]By property <strong>of</strong> proportions,ARRB76[ ARC][ARS ] [ ASC ] .[ RBC][RBS ] [ CSB]
EduMath 25 (12/2007)Similarly,BP [ BSA] andPC [ ASC]CQ [ CSB] .QA [ BSA]AR BP CQ [ ASC ] [ BSA][ CSB]So, 1RB PC QA [ CSB][ ASC][ BSA].Six Different <strong>Pro<strong>of</strong>s</strong> <strong>of</strong> Ceva’s <strong>Theorem</strong> <strong>by</strong> the <strong>Students</strong>Pro<strong>of</strong> 1. (<strong>by</strong> Monzon Abel, Chan Chi Fai, Chan Ka Lun, Chan Wing YanJoyce, Hui Chiu Kit, Hui Man Wa, Wendy Chow, Kan Ka Yin, Lam Ching Yan,Lam Hiu Yue, Leung Ka Hei, Lo Ching Hoi, Mok Tung Hoi, Ning Man Chit, SoTsz Chung, Tan Pak Chiu, Nicole Tin, Tsang Ting Ho, Wong Cheuk Kwan,Wong Ching, Wong See Wai, Yu Lik Hang and Anglea Wu)YAXRQSBPFigure 2CLet X and Y be points on BQ produced and CR producedrespectively such that XAY is a straight line parallel to BC (figure 2).As ΔARY ~ ΔBRC and ΔAQX ~ ΔCQB , we haveAR YA CQ BC and .RB BC QA AXAs ΔASX ~ ΔPSB and ΔASY ~ ΔPSC , we haveBP PS PCBP AX , and so .AX SA YAPC YAAR BP CQ YA AX BCHence 1RB PC QA BC YA AX.77
數 學 教 育 第 二 十 五 期 (12/2007)Pro<strong>of</strong> 2. (<strong>by</strong> Kwan Chin Wai, Tai Wai Ming)XAYRQSBPCFigure 3Let X and Y be points on BQ produced and CR producedrespectively such that BY and CX are both parallel to PA (figure 3).As ΔARS ~ ΔBRY and ΔAQS ~ ΔCQX , we haveAR SA CQ CX and .RB BY QA SAAs AP // XC and ΔBYS ~ ΔXCS , we haveAR BP CQ SA BY CXHence 1RB PC QA BY CX SAPro<strong>of</strong> 3. (<strong>by</strong> Kan Ka Wing)ABPPC.BS BY .SX CXRQSXBPCYFigure 478
EduMath 25 (12/2007)Let X and Y be points on the line BC such that AX // BQ andAY // RC (figure 4).As ΔCQB ~ ΔCAX and ΔBRC ~ ΔBAY , we haveCQ BC AR CY and .QA BX RB BCAs ΔBPS ~ ΔXPA and ΔPSC ~ ΔPAY , we haveBP PS PCBP XB , and so .XB SA CYPC CYAR BP CQ CY BX BCHence 1RB PC QA BC CY BX.Pro<strong>of</strong> 4. (<strong>by</strong> Wong Wai Lung)ARQISJBXPYCFigure 5Let X and Y be points on BC such that XR and YQ areboth parallel to PA . Let I be the point <strong>of</strong> intersection <strong>of</strong> BQ andXR , and J be the point <strong>of</strong> intersection <strong>of</strong> CR and YQ (figure 5).By the intercept theorem, we haveAR PX andRB XBCQ CY .QA YPAs XR // PA and ΔBIR ~ ΔBSA ,we havePBXBSB AS .IB RIAs YQ // PA and ΔCJQ~ ΔCSA , we haveCYCPCJ QJ .CS AS79
數 學 教 育 第 二 十 五 期 (12/2007)ASRIHenceQJASARRBRSSJBPPCQJRICQQARSSJ1PXXB.BPPCCYYPBPXBCYPCPXYPThe last equality follows as ΔRIS~ ΔJQS .Pro<strong>of</strong> 5. (<strong>by</strong> Hui Shun On)AXRSQYBPFigure 6CLet X be the point on AB such that PXis parallel to CR . LetY be the point on AC such that PY is parallel to BQ (figure 6).By the intercept theorem, we haveAR AS AQ RX PC , andRX SP QY RB BCCQ BC .YQ BPHenceAQQYPCBCARRBBPPCBPPCYQQACQQABCBP1ARRX.RXRBBPPCYQQACQYQPro<strong>of</strong> 6. (<strong>by</strong> Leung Yeung Yeung)Let X and Y be points on AP produced such that BX isparallel to RC and CY is parallel to QB (figure 7).By the intercept theorem, we haveAR AS andRB SXCQ SY .QA AS80
AEduMath 25 (12/2007)RQSBXPCYFigure 7As ΔBPS ~ ΔCPY and ΔBSX ~ ΔCYS , we haveBP SB SX .PC CY SYAR BP CQ AS SX SYHence 1RB PC QA SX SY AS.RemarksThe “Usual Pro<strong>of</strong>” is adopted from Geometry Revisited <strong>by</strong> Coxeter andGreitzer (1967). Some <strong>of</strong> the students’ pro<strong>of</strong>s appear also in some geometrytextbooks. For example, pro<strong>of</strong>s similar to “Pro<strong>of</strong> 1” and “Pro<strong>of</strong> 2” are alsopresented in Posamentier’s book Advanced Euclidean Geometry (2002).One common point <strong>of</strong> all the pro<strong>of</strong>s <strong>by</strong> the students is the use <strong>of</strong> auxiliaryparallel lines on the triangle to relate ratios <strong>of</strong> segments to other ratios <strong>of</strong>segments. During the workshop, I encouraged the students to first assumesome numerical values on the ratios AR : RB and CQ : QA and try tocalculate the remaining ratio BP : PC with the help <strong>of</strong> adding auxiliary81
數 學 教 育 第 二 十 五 期 (12/2007)parallel lines. By this concrete computation, students were able to understandthe relationships between ratios <strong>of</strong> the line segments on the triangle. Thepro<strong>of</strong>s then should be followed <strong>by</strong> generalizing AR : RB and CQ : QA toany arbitrary ratios.The aim <strong>of</strong> this homework was to encourage students’ participation inproving geometric theorems rather than memorizing pro<strong>of</strong>s. I hoped that thestudents could develop some generic problem solving skills for future geometricproblems.ReferenceCoxeter, H.S.M., Greitzer, S. L. (1967). Geometry Revisited. Mathematical Association <strong>of</strong>America.Posamentier, Alfred S. (2002).Advanced Euclidean Geometry. Key College Publishing.Email: whp@stpauls.edu.hk82