The St. Louis Gateway Arch

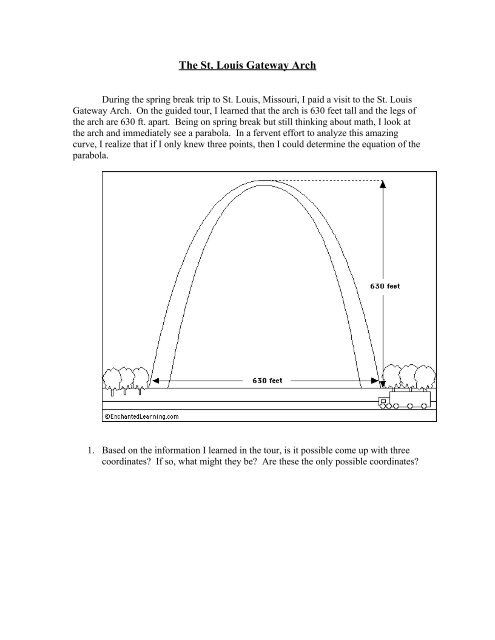
The St. Louis Gateway ArchDuring the spring break trip to St. Louis, Missouri, I paid a visit to the St. LouisGateway Arch. On the guided tour, I learned that the arch is 630 feet tall and the legs ofthe arch are 630 ft. apart. Being on spring break but still thinking about math, I look atthe arch and immediately see a parabola. In a fervent effort to analyze this amazingcurve, I realize that if I only knew three points, then I could determine the equation of theparabola.1. Based on the information I learned in the tour, is it possible come up with threecoordinates? If so, what might they be? Are these the only possible coordinates?

2. Suppose you orient a coordinate grid with the bottom of one leg of the arch at theorigin and the rest of the arch lying in the first quadrant so that the other leg isalso on the x-axis. Sketch the arch in the first quadrant below and label as manycoordinates as you can on the arch.

23. Using the general form of a quadratic function, y = a ⋅ x + b ⋅ x + c and the threecoordinates labeled above, write a system of three equations to solve for the threeunknowns, a,b and c.HINT: You will get c directly from one of the equations. Substitute this valueinto the other two. Use one of the other equations to find an equation for b interms of a, and substitute this value for b into the last equation and solve for a.You now have numerical values for a and c use these to solve for b.

4. Using the values for a,b and c from question 3 write the quadratic equation for theGateway Arch and sketch it.

5. Is there another way to orient the coordinate axes that might make finding thequadratic equation easier? Choose another orientation and find the quadraticequations using the new coordinate system. How are the two quadratic equationsrelated?

6. The formula actually used in constructing the St. Louis Arch is displayed on theinside of the arch. It is the formula for a catenary curve, which is the shape afree hanging chain takes when held at both ends. Mathematically, the functionthat models such a curve is hyberbolic cosine. The formula used for the St. LouisArch is y = 68.8⋅ cosh(.01⋅x −1). Use your graphing calculator to graph thisfunction. (If you have trouble find the cosh function, use the catalog feature ofyour calculator.) Is this graph close to the graph of either of the quadraticfunctions you found? Can you transform either of the functions (the quadratic orthe hyberbolic cosine) in a way that preserves size (using only reflections andtranslations) to show that they model close to the same physical structure?December 20, 2004. Ensuring Teacher Quality: Algebra II, produced by the Charles A. Dana Center at The Universityof Texas at Austin for the Texas Higher Education Coordinating Board.

Solutions1. Based on the information I learned in the tour, is it possible come up with threecoordinates? If so, what might they be? Are these the only possible coordinates?The students can orient the coordinate system in many different ways andget different sets of coordinates. Example:(0, 0), (315, 630) and (630, 0) or(-315, 0), (0, 630) and (315, 630)2. Suppose you orient a coordinate grid with the bottom of one leg of the arch at theorigin and the rest of the arch lying in the first quadrant so that the other leg isalso on the x-axis. Sketch the arch in the first quadrant below and label as manycoordinates as you can on the arch.

23. Using the general form of a quadratic function, y = a ⋅ x + b ⋅ x + c and the threecoordinates labeled above, write a system of three equations to solve for the threeunknowns, a,b and c.HINT: You will get c directly from one of the equations. Substitute this valueinto the other two. Use one of the other equations to find an equation for b interms of a, and substitute this value for b into the last equation and solve for a.You now have numerical values for a and c use these to solve for b.This creates an easy solution:Eqn 1: c = 0Eqn 2: 315 2 ⋅ a + 315⋅b+ c = 630Eqn 3: 630 2 ⋅ a + 630 ⋅b+ c = 0Substitute c into Eqn 2 and 3,31563022⋅ a + 315⋅b= 630⋅ a + 630 ⋅b= 0From 2 write b in terms of a,3152⋅ a + 315⋅b= 6302630 − 315 ⋅ ab == 2 − 315⋅a315Substitute in 3 and solve for a630 2 ⋅ a + 630 ⋅ (2 − 315⋅a)= 0630 ⋅ (630 − 315) ⋅ a = −2⋅ 630− 2a = = −.00635315Solve for bb = 2 − 315⋅ab = 2 − 315⋅( −.00635)= 4Answer:a = -.00635b = 4c = 0

4. Using the values for a,b and c from question 3 write the quadratic equation for theGateway Arch and sketch it.2y = −.00635⋅x + 4 ⋅ x

5. Is there another way to orient the coordinate axes that might make finding thequadratic equation easier? Choose another orientation and find the quadraticequations using the new coordinate system. How are the two quadratic equationsrelated?One possibility is to center the legs around the y-axis and get the followingthree coordinates, (-315, 0), (0, 630) and (315, 0). By using knowledge abouttransformation of the parent function y=x 2 we get the following solution:y = −a⋅ x2 + 630Plug in any value for y and x gives,20 = −a⋅315+ 630630a =2315= .00635Leads to,y = −.00635⋅x2 +630The two equations differ only in translation.

6. The formula actually used in constructing the St. Louis Arch is displayed on theinside of the arch. It is the formula for a catenary curve, which is the shape afree hanging chain takes when held at both ends. Mathematically, the functionthat models such a curve is hyberbolic cosine. The formula used for the St. LouisArch is y = 68.8⋅ cosh(.01⋅x −1). Use your graphing calculator to graph thisfunction. (If you have trouble find the cosh function, use the catalog feature ofyour calculator.) Is this graph close to the graph of either of the quadraticfunctions you found? Can you transform either of the functions (the quadratic orthe hyberbolic cosine) in a way that preserves size (using only reflections andtranslations) to show that they model close to the same physical structure?The catenary curve described by the formula is a reflection and a translationof the curve modeled with the quadratic. In order to see this, it can bemanipulated by the parameters that will not change the size of our arch. Thecatenary curve can be transformed to y = −68 .8⋅cosh⋅(.01⋅x − 3.15) + 700 .This graph and the first quadratic model created are very close together.Some discrepancies occur because of where the tour guide’s measurementsare taken. We are told that the legs are 630 feet apart. This measurement istaken from the outside edge of both legs. A graphic of the actual arch withthe published catenary curve overlaid shows the catenary curve does notfollow the outer edge of the arch, but instead represents an internalstructure.Still, the two curves are very close.