Population Growth Exponential vs Logistic Growth - The Burns ...

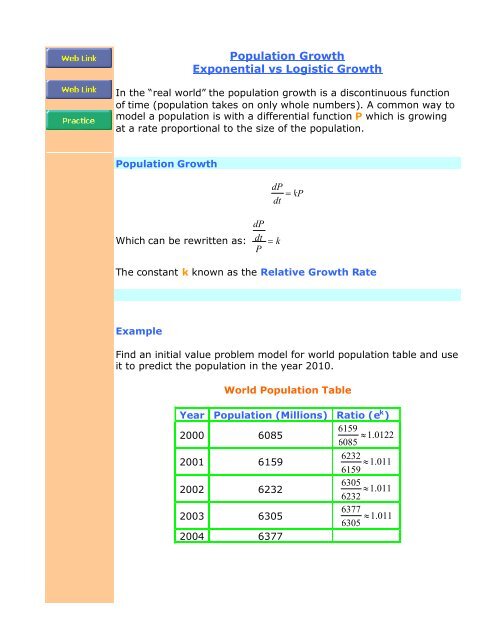
Population GrowthExponential vs Logistic GrowthIn the “real world” the population growth is a discontinuous functionof time (population takes on only whole numbers). A common way tomodel a population is with a differential function P which is growingat a rate proportional to the size of the population.Population GrowthdP kPdt Which can be rewritten as:dPdt kP The constant k known as the Relative Growth RateExampleFind an initial value problem model for world population table and useit to predict the population in the year 2010.World Population TableYear Population (Millions) Ratio (e k )2000 608561596085 1.01222001 61592002 62322003 63052004 63776232 1.0116159 6305 1.0116232 63776305 1.011

Solution:If we let t=0 represent 2000, then t=10 represent 2010.From the tablee k is approximated by the value 1.011Now since dPdt kP , we have k being about 0.0109.dPDifferential Equation: 0.0109PdtInitial Condition:P0 6085Solving this gives usP 6085eP 106085e6785.80.0109 t0.109The world’s population is expected to be about 6.8 billion people by2010.The problem with exponential growth models is that they assumeunlimited growth. This unlimited growth assumption is only valid forsmall periods of time. The environment will place a constraint on thegrowth and a more realistic model is used. The Logistic GrowthModel.Logistic GrowthdPdtP P k1MdP k P M P or dt MWhere M is a maximum population or carrying capacity that theenvironment is capable of sustaining.

ExampleA farm is known to support no more than 100 cows. The farmcurrently has 10 cows. Model the population with a logistic differentialequation with k 0.1 . And find how long it takes for the farm to reach25 cows.Solution:dP k P M P dt M0.1 P 100P1000.001P100 PWe knowP0 101 dP0.001P 100P dt1 1 1 dP0.001 ; separation technique100 P 100 P dt1 1 dP 0.1P 100 P dtln P ln 100 P 0.1t CPln 0.1tC100 P100 Pln 0.1t CP100 P0.1tCeP100 PC 0.1t e eP100 10.1t AeP100P 0.1t1 AeThis is the general solution to the differential equation. When t=0 weknow P=10.10010 1 Ae1A10A 90

100This gives us the Logistic growth Model: P 0.1t1 9 e Now to determine when P=2510025 1 9e0.1t19 e 40.1t9e3e0.1te0.1t133ln 3t 0.1110.1tIt would take about 11 years to reach 25 cows.The growth curve is represented below.