Calculation of the Maxwell stress tensor and the Poisson-Boltzmann ...

Recommendations

Info

084904-4 Lu et al. J. Chem. Phys. 123, 084904 2005r i /4r 2 N v J. J is the Jacobian from the coordinatetransformation, and here simply doubles the element area,From the coordinate transformation from r to ,, wefindx i − y i = A i ,20FIG. 2. A flat triangle in the three-dimensional space is mapped to a rightisosceles triangle on the plane. The singular point y, its surroundingcircular patch e , and their images in the transformed triangle are alsoshown.singular integral part, i.e., the last term on the right-hand sideof Eq. 16, can be treated following a similar procedure.For our specific case, because we use a flat triangularelement and linear shape functions, the derivation of hypersingularintegral formulation can be more simple andstraightforward. Here we give the detailed derivation. Let usfirst consider the case that the singular point y locates in theelement, and suppose in the limiting process the patch e iscircular and of radius centered at point y see Fig. 2. Inthis case, we can then show as following that part of theintegral the circular patch-related part is solved analyticallyand the remainder numerically, which was also discussed byAllison. 21 In the implementation of the boundary elementintegral, the surface triangles in the physical threedimensionalspace are mapped to an isosceles triangle on the parametric plane, as shown in Fig. 2, where the integrationon each element is actually performed.The values of the coordinates, the potential, and its normalderivative at any position in the element are obtained bylinear interpolation from the corresponding values on threenodes, respectively. For example, for a point , in parametricspace, its coordinates in the original space is x=N 1 ,x 1 +N 2 ,x 2 +N 3 ,x 3 , where N i i=1,2,3 arethe shape functions, and x 1 ,x 2 ,x 3 are the original coordinatesof the three nodes of the element. Here, N 1 =1−−, N 2=, and N 3 =.Now, we use polar coordinate , in the parametricspace , to perform the integral. Denoting Eq. 16 as12 v ,i =−v o ,i − I + I W ,17we find that the hypersingular part I and the strong singularpart I W areI = lim→0 +s e −e V i y,xN v n Jdd + vy b iy, 18Jdd I W = lim W i y,xN v ,→0 +s e −e 19where v and v n ,=1,2,3, denote the value v and vx/nat node , respectively, and the doubly appeared superscriptsor subscripts implicitly mean a summation, i.e., N v n= N v n . Note that r/n=0 on the flat surface, the hypersingularintegrand in Eq. 18 is thus reduced ton i /4r 3 N v n J, and the strong singular integral is given bywherer = A,A i = x 2 i − x 1 i cos + x 3 i − x 1 i sin ,21223A = A i 21/2, 23i=1andN = N o + N 1 , = 1,2,3. 24Here, A, A i , N 0 , and N 1 have the same definitions as in Ref.16. N 0 is a constant that depends on the position of the singularpoint, namely, the position where we want to calculatethe derivative of the potential. The contour of the neighborhoode given by the expression =r now in the parameterplane is expressed in polar coordinates by = A i .25By using the reversion of the above expression, we thenobtain the expression in of the equation in polar coordinatesof the contour e the image of e , see Fig. 2 = /A i .26Now, using the above formulas expansion Eqs.20–26 and performing the hypersingular and strong singularintegrals, we obtainI = lim→0 +02 A−3Jn i N 1lnˆ −ln +lnA4− A−3 Jn i N 04 1ˆ + 1Ad + vy b iy , 27I W = lim→0 +02 A−3A i JN 1ˆ4+ A−3 A i JN 0lnˆ −ln4+lnAd .28Note that A=A+, A i =−A i +, and N 1 =−N 1 +, the integral of the terms involving ln in bothEqs. 27 and 28 is equal to zero. Because the final value Iin Eq. 27 is finite, in the limiting process, the integral resultof the term involving 1/ must be canceled by the termvyb i y/. Then, the singular integrals are transformedwithout any approximation into regular integrals one dimensionin our case, they areI =012F −1 lnˆA − F −2 ˆd,29Downloaded 02 Sep 2005 to 132.239.16.167. Redistribution subject to AIP license or copyright, see http://jcp.aip.org/jcp/copyright.jsp
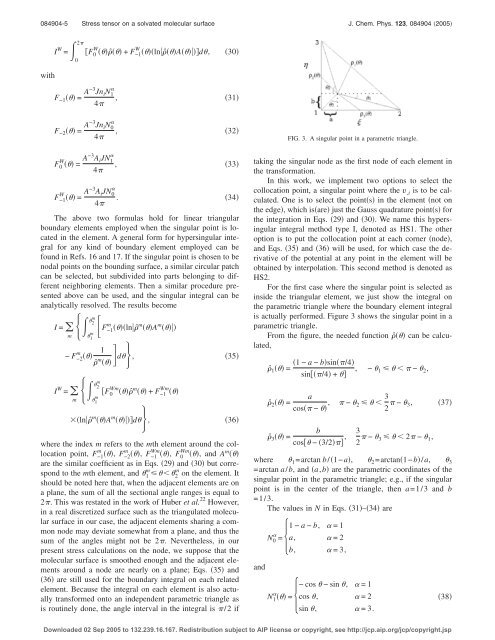
084904-5 Stress tensor on a solvated molecular surface J. Chem. Phys. 123, 084904 2005I W =02F W 0 ˆ + F W −1 lnˆAd,30withF −1 = A−3 Jn i N 1, 314F −2 = A−3 Jn i N 0, 324F W 0 = A−3 A i JN 1, 334F W −1 = A−3 A i JN 0. 344The above two formulas hold for linear triangularboundary elements employed when the singular point is locatedin the element. A general form for hypersingular integralfor any kind of boundary element employed can befound in Refs. 16 and 17. If the singular point is chosen to benodal points on the bounding surface, a similar circular patchcan be selected, but subdivided into parts belonging to differentneighboring elements. Then a similar procedure presentedabove can be used, and the singular integral can beanalytically resolved. The results become 2I = mF m −1 lnˆ mA m mm 1− F m −2 1ˆ md ,mI W 2= F Wm 0 ˆ m + F Wm −1 mm 1lnˆ mA m d ,3536where the index m refers to the mth element around the collocationpoint, F m −1 , F m −2 , F Wm −1 , F Wm 0 , and A m are the similar coefficient as in Eqs. 29 and 30 but correspondto the mth element, and m 1 m 2 on the element. Itshould be noted here that, when the adjacent elements are ona plane, the sum of all the sectional angle ranges is equal to2. This was restated in the work of Huber et al. 22 However,in a real discretized surface such as the triangulated molecularsurface in our case, the adjacent elements sharing a commonnode may deviate somewhat from a plane, and thus thesum of the angles might not be 2. Nevertheless, in ourpresent stress calculations on the node, we suppose that themolecular surface is smoothed enough and the adjacent elementsaround a node are nearly on a plane; Eqs. 35 and36 are still used for the boundary integral on each relatedelement. Because the integral on each element is also actuallytransformed onto an independent parametric triangle asis routinely done, the angle interval in the integral is /2 iftaking the singular node as the first node of each element inthe transformation.In this work, we implement two options to select thecollocation point, a singular point where the v ,i is to be calculated.One is to select the points in the element not onthe edge, which isare just the Gauss quadrature points forthe integration in Eqs. 29 and 30. We name this hypersingularintegral method type I, denoted as HS1. The otheroption is to put the collocation point at each corner node,and Eqs. 35 and 36 will be used, for which case the derivativeof the potential at any point in the element will beobtained by interpolation. This second method is denoted asHS2.For the first case where the singular point is selected asinside the triangular element, we just show the integral onthe parametric triangle where the boundary element integralis actually performed. Figure 3 shows the singular point in aparametric triangle.From the figure, the needed function ˆ can be calculated,1−a − bsin/4ˆ 1 = , − 1 − 2 ,sin/4 + ˆ 2 =ˆ 3 =acos − , − 2 3 2 − 3, 37bcos − 3/2 , 32 − 3 2 − 1 ,where 1 =arctan b/1−a, 2 =arctan1−b/a, 3=arctan a/b, and a,b are the parametric coordinates of thesingular point in the parametric triangle; e.g., if the singularpoint is in the center of the triangle, then a=1/3 and b=1/3.The values in N in Eqs. 31–34 are=1−a − b, =1N 0a, =2b, =3,andFIG. 3. A singular point in a parametric triangle.=− cos − sin , =1N 1 cos , =238sin , =3.Downloaded 02 Sep 2005 to 132.239.16.167. Redistribution subject to AIP license or copyright, see http://jcp.aip.org/jcp/copyright.jsp
Page 1: THE JOURNAL OF CHEMICAL PHYSICS 123
Page 6 and 7: 084904-6 Lu et al. J. Chem. Phys. 1
Page 8: 084904-8 Lu et al. J. Chem. Phys. 1

Calculation of the Maxwell stress tensor and the Poisson-Boltzmann ...

Create successful ePaper yourself

Delete template?

Save as template?